Tag Archives: Mathematics

“The Crow and the Pitcher” and Close Packing of Equal Spheres

Posted on March 16, 2020 by | 1 comment

 The Crow and the Pitcher

The Crow and the Pitcher is one of Aesop’s Fables, which tells a story of: A thirsty Crow found a high and narrow-neck pitcher with a little water in it, the crow could not reach the water no matter how it tried. Suddenly, the crow came up with an idea: picking up some small rocks and dropping them to the pitcher. With each pebble, the water rose higher and higher. Finally, the crow drank the water.

The Crow and the Pitcher, Aesop’s Fables. (Copyright: Milo Winter)

This story is very inspiring to many children, it told them to think flexibly when facing problems. However, is it possible for the crow to drink the water with rocks in real life?

Close Packing of Equal Spheres

The problem in the Crow and the Pitcher is that after the crow dropped rocks to the flask, the water filled the space between rocks first, then it raised the height of water. How much water was needed to fill the space between rocks, this is the closest packing problem.

The Close Packing of Equal Spheres was first put forwarded by Kepler in the 17th-century. Kepler thought that the close packing of equal spheres in a three-dimensional space looks like the following:

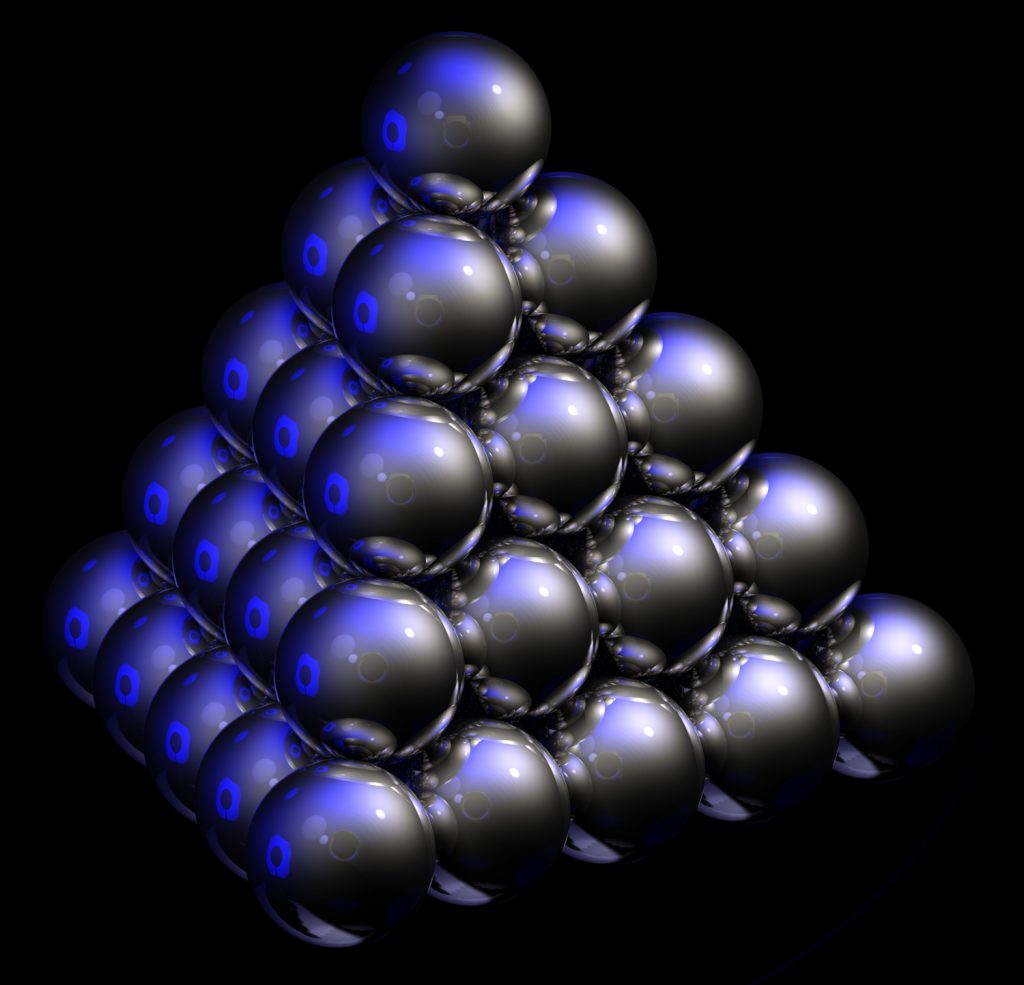
The cannonball stack: an “FCC” latice. (Copyright: Wikipedia)

The close packing of equal spheres can be widely found in chemistry, such as crystal structures of Magnesium and Copper atoms. It was calculated by Carl Friedrich Gauss that the greatest fraction of space occupied by spheres – that can be achieved by close packing of equal spheres is 74%. In other words, if the water in the pitcher is 26% (volume) or less, the crow cannot drink the water no matter how many rocks it put in the pitcher.

The close packing of equal spheres also called the Hexagonal Close Packing. In 2017, the scientist proved that the Hexagonal Close Packing is the densest arrangement in the three-dimensional space.

 How can the crow drink the water in the pitcher? 

Is there a way that the crow can drink the water in the pitcher without 26% of water? Mathematicians said that by combining the truncated octahedrons, tetrahedrons and octahedrons (2:1), or truncated cubes and octahedrons (1:1), or gossip mirrors and truncated cuboctahedrons (3:1), the crow can easily drink all the water in the pitcher.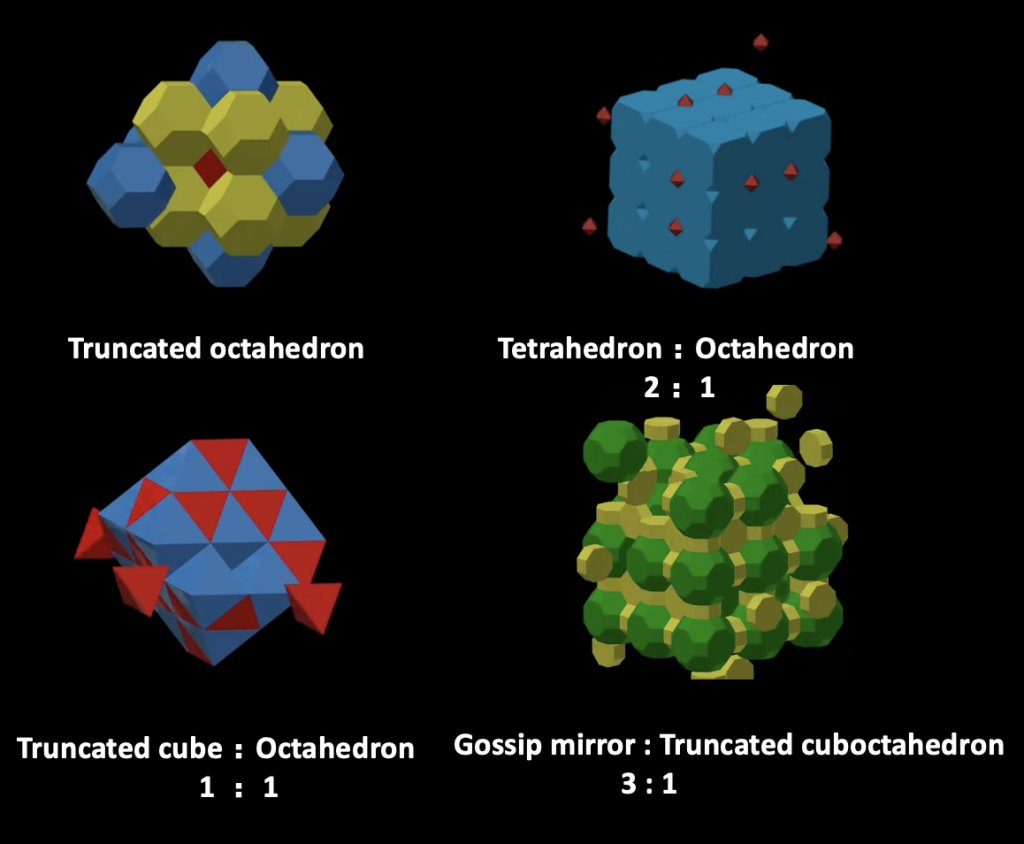

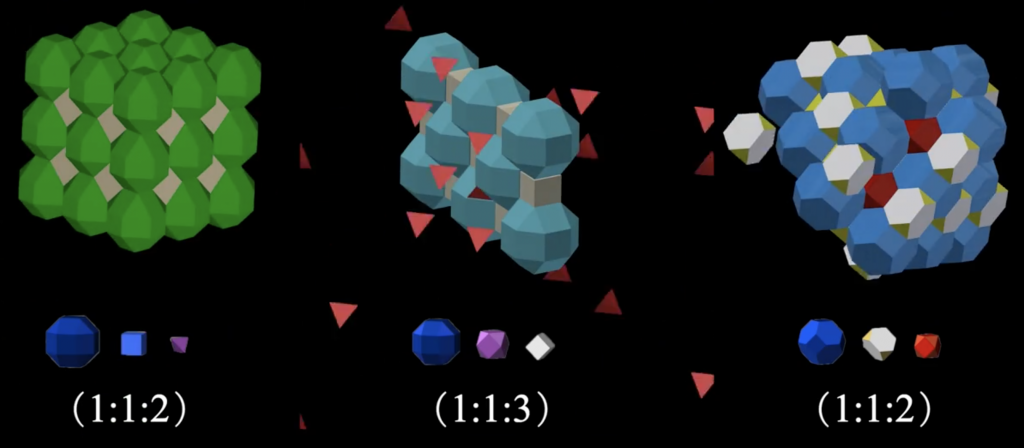
Alternative ways for the crow to drink water in the pitcher. (Copyright: ScienceDirect)

If it is hard for the crow to find the above rocks, it can also combine multiple shapes of rocks to get the water in the pitcher. Moreover, some scientists tested the effect of shapes of flasks on the increasing heights of water in flasks by using the Close packing of equal spheres. They found that the height of water in Erlenmeyer-flask-shaped flasks has the fastest increase. However, where can you find an Erlenmeyer flask? -A chemistry lab. It’s absolutely a bad idea to drink solutions in a chemistry lab.

1 Comment

Posted in Popular Science

Tagged , , , , ,

Strategic Dating: The 37% Rule

Posted on February 24, 2020 by | 3 comments

Dating and settle down are big problems for a lot of people, especially for many scientists, who spend their entire life in labs. For rich people, dating can be easily solved by economics. However, for the poor, dating is a metaphysics problem. How can we dating and settle down efficiently? It turns out that there are many mathematical rules that tell you how long you ought to search, and when you should stop searching and settle down.

Secretary problem

The secretary problem is a problem that demonstrates a scenario involving optimal stopping theory. In the secretary problem, an administrator is interviewing n applicants in turn to help the company to hire a best secretary. A decision must be made immediately after each interview, and once the interviewee has been rejected, it cannot be recalled. The question is about the optimal strategy to maximize the probability of selecting the best applicant.

Similarly, for dating and marriage, you must decide whether to settle down with your current boyfriend or girlfriend at some time points. This can be solved by the secretary problem. For example, assuming you have 3 different boyfriends in your lifetime, and you need to choose one to marry him. The best strategy for you is to break up with your first boyfriend regardless of how excellent he is, try the second one. If the second one is better than the first one, marry him, or try the third one. In this case, you have ½ probability to choose the best guy, which is more probable than choosing randomly.

Demonstration on the scenario talked above.

The 37% Rule

However, in the real life. The sample size is unpredictable, and largely depends on a lot of things. How large the sample size should be and how many boyfriends should you “try” before you make the decision?

First, we can make an assumption: There are n boys chasing you, you try first k (k<n) boys but reject all of them. From the k+1 boy, settling down if he is better than the previous ones. For example, when n=4, there’re 4 possible k values: k=0, 1, 2, 3. By listing all possible cases, we got when k=1, P(1)=11/24, which is the best strategy for you to choose the “Mr. Right”.

Possible cases for Sample size=4. (Source: DataGenentics)

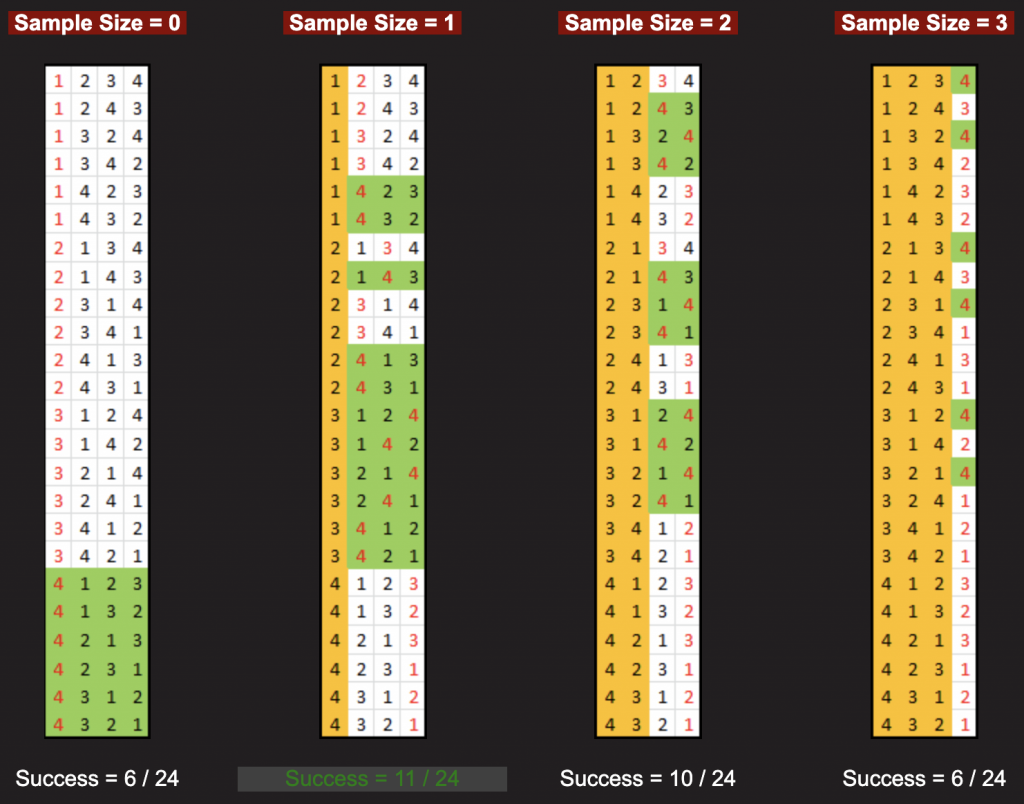
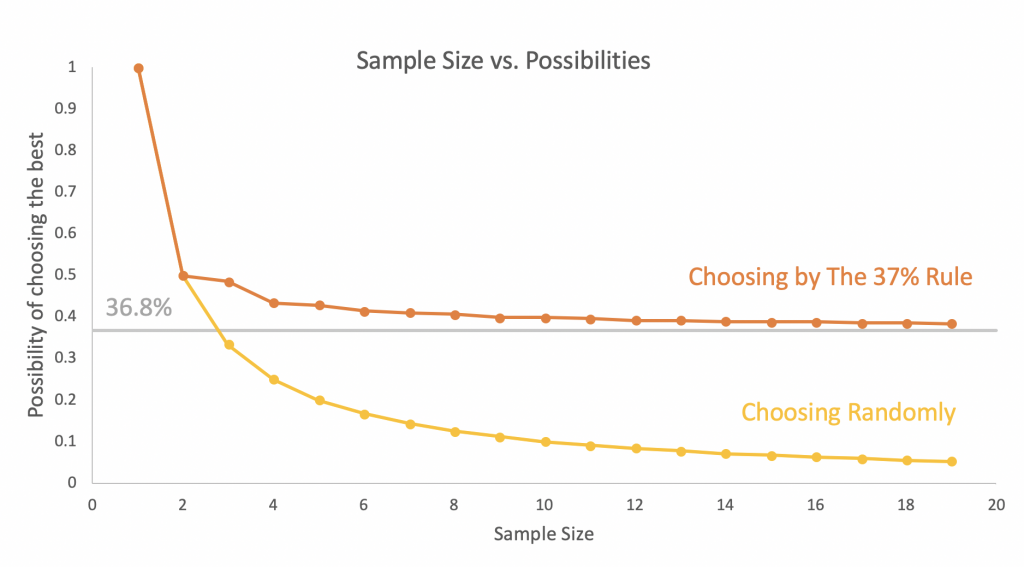
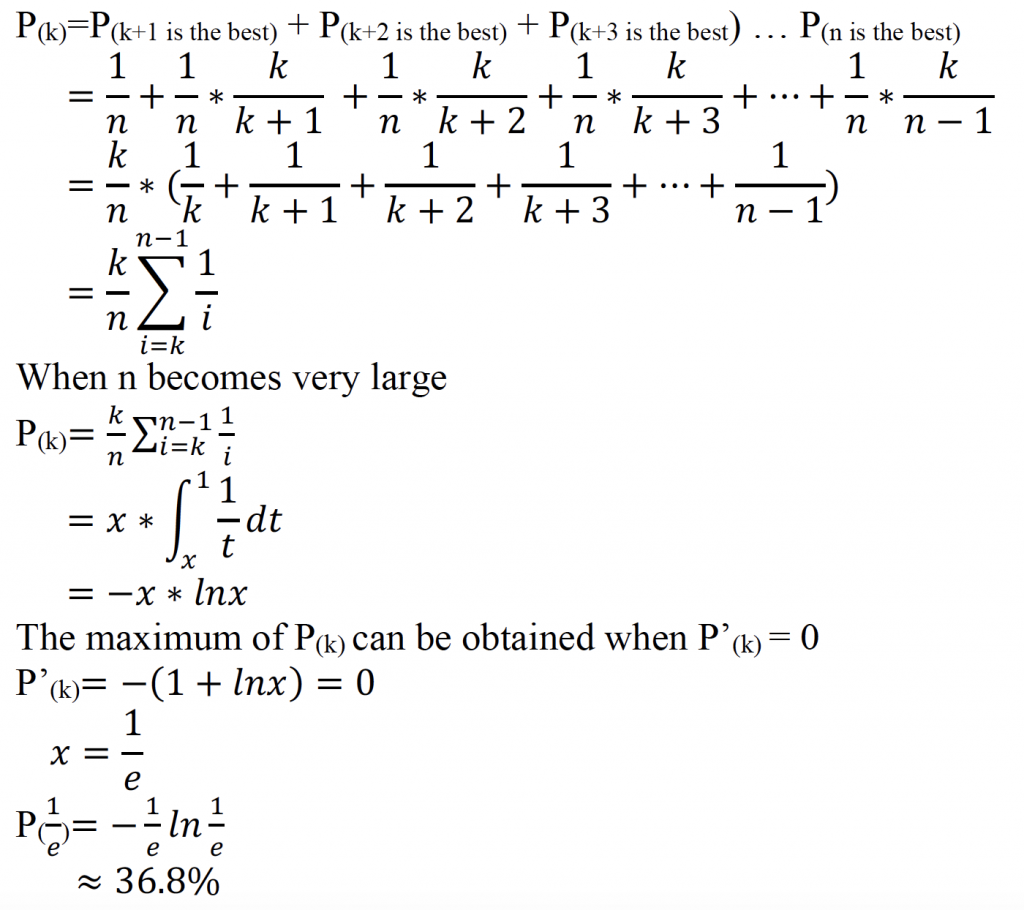More generally, for a very large sample size, the probability can be calculated from Riemann integral and its derivative:Which means you should settle down immediately when you meet the “best boy” after you try first 36.8% guys in your life.

Comparing with choosing randomly, it is obvious that when the sample size is larger than 2, using The 37% Rule is much more possible to settle down with your “Mr. Right”.

A comparison of choosing randomly and choosing by the 37% rule. (Data Source: DataGenentics)

However, in the real world, you never know how large the sample size is. Although we can approximate a n value by combining many factors like economic status, educations, personal experiences, family background, face score and etc. Life is not a game, so settle down in a relationship with your true love when you think he or she is your best choice.

3 Comments

Posted in Popular Science

Tagged , , ,