Tag Archives: inquiry

Illuminating Illuminations

Posted on by
Reply

In their investigation into the effectiveness of computer simulations, Finkelstein et al. (2005) concluded that “the conventional wisdom that students learn more via hands-on experience is not borne out by measures of student performance on assessment of conceptual understanding” due to their findings that “properly designed simulations used in the right contexts can be more effective educational tools than real laboratory equipment, both in developing student facility with real equipment and at fostering student conceptual understanding”. Providing students with opportunities to explore concepts in a variety of contexts enriches the learning environment and diversifies instruction to better meet student needs. In the mathematics lesson below, the concept of fractions is embedded in inquiry-based activities to help students visualize this concept while exploring selected interactive applets. Reflection plays a key role in the intended abstraction as a means of guiding students through a process of self-assessment to better understand their conceptual understanding.

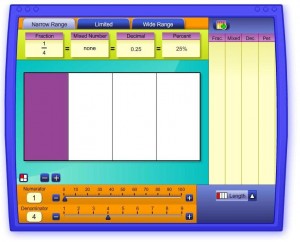
Illuminations Lesson for Fractions
(click link above for lesson)

Rationale

By Grade 7, students are expected to have a basic, but solid, understanding of fractions so that they can proceed with more in depth explorations of relationship comparisons and eventually addition and subtraction operations. Unfortunately, this is often not the case and measures need to be taken to assist students’ conceptualization of fractions in preparation of their extensive use in math strands in successive grades. Much of the problem seems to lay with students’ misconception and simplification of fractions down to sets of rules to be committed to memory creating inaccurate mental models. It’s not surprising then that they are frequently unable to adapt strategies they have used in one context to fit a new situation. Their knowledge of fractions remains superficial and does not lead them to a deeper understanding of what fraction symbols communicate as a representation of a whole. When learning abstract concepts, such as fractions, students must understand the fallacy of focusing on memorization as it “leads to ‘inert knowledge’ that cannot be called upon when it’s useful” (as cited in Edelson, 2001) resulting in a poor or non-existent transfer of skills.

Using Illuminations activities provides students with a “variety of visual cues in the computer simulations [to] make concepts visible that are otherwise invisible” (Finkelstein et al., 2005) or at least more difficult to visualize. When integrated into an inquiry-based framework, they can be used to enhance students’ abstraction of fraction concepts while promoting the acquisition of adaptive expertise and thinking skills.

Intertwining the constructivist principles of the Learning for Use framework and T-GEM instructional model provides and impressive foundation for math explorations. The GEM cycle stages of Generate – Evaluate – Modify are complemented by the 6 tenets of LfU, motivate, elicit curiosity, observations, knowledge construction, refine and apply. While collecting information and generating ideas, curiosity and motivation are provoked as students realize what they do not yet know, but need to in order to be able to complete the task. Through key observations, students construct knowledge as they begin to evaluate their assumptions around relationships between variables. As students work to modify their original theories, they need to refine and apply new understandings that have arisen from their investigation. Applications of the LfU and T-GEM frameworks to instructional design presume that overlaps in each of the stages will occur as they both involve a cyclical process of exploration and inquiry. In fact, several cycles may be needed due to the incremental nature of learning; however, the order of the stages remains a critical factor. In the lesson outlined above, two complete cycles of T-GEM and LfU can be observed.

 

British Columbia Grade 7 Math Learning Outcome (Number – A7)

  • compare and order positive fractions, positive decimals (to thousandths) and whole numbers by using
    • benchmarks
    • place value
    • equivalent fractions and/or decimals

Comparing percent to fractions and decimals is a Grade 6 outcome, but by Grade 7 this is consistently not understood well so it needs to be re-taught in preparation for Grade 8 expectations with percent (greater than 100% and fractions of percent between 0 and 1) providing students with a more substantive opportunity to understand the overriding relationships between all three values; therefore, in this activity, this Grade 6 learning outcome will be reinforced as an integral component of the task.

Grade 6 Math Learning Outcome (A6): demonstrate an understanding of percent (limited to whole numbers) concretely, pictorially, and symbolically.

Before beginning lessons involving self-directed exploration of Illuminations activities, students must possess sufficient background knowledge to prepare them for success with the simulation activity. If the expectations for student learning are high given their current context, they will have difficulty navigating the activity (Kalyuga in Srinivasan, S. et al, 2006) and finding the necessary motivation to learn what they need to know to be successful. In this scenario, essential prior knowledge includes an understanding of:

  • numerator, denominator, common/proper fraction, improper fraction, mixed number, whole number, simplest form, equivalent fractions, multiple, factor, benchmark fractions/decimals/percents, addition equations equaling 1 whole, decimal place value (tenths, hundredths), parts of one, relating fractions to decimal place value, percent

Along with having prior knowledge, students must be able to access and activate it; therefore, the initial introductory task is intended as a revision of fraction concepts and relationships, which become essential elements, within the subsequent scaffolded activities.

(This post serves as further reflection on the application of knowledge representation and information visualization as it applies to my future personal practice and includes the alternate activity requested in lieu of directly related papers on the use of Illuminations)

References

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching,38(3), 355-385. http://onlinelibrary.wiley.com/doi/10.1002/1098-2736%28200103%2938:3%3C355::AID-TEA1010%3E3.0.CO;2-M/abstract

Finkelstein, N.D., Perkins, K.K., Adams, W., Kohl, P., & Podolefsky, N. (2005). When learning about the real world is better done virtually: A study of substituting computer simulations for laboratory equipment. Physics Education Research,1(1), 1-8. Retrieved April 02, 2006, from: http://phet.colorado.edu/web-pages/research.html

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905.

Srinivasan, S., Perez, L., Palmer, R., Brooks, D., Wilson, K. & Fowler, D. (2006). Reality versus simulation. Journal of Science Education and Technology, 15(2), 1-5

Walking the Talk …

Posted on by
Reply

The most prevalent obstacle that impedes inquiry-based learning in educational settings is the instructor’s understanding of inquiry and pedagogical approaches as well as the ability to implement these successfully. This was shared through the expressed frustrations of the Jasper Series designers when teachers did not seem to recognize the value in exposing students to analog problems that were conceived for the purpose of improving transfer and abstraction of concepts and strategies, opting instead for adventures that introduced the need to use different skills overlooking the opportunity to increase adaptive expertise (Hatano, 1984). Within the WISE environment, customizing the platform for successful inquiry-based learning requires a level of competence that designers cannot necessarily assume teachers possess. The inquiry map alone, which directs students through the process, can present a significant challenge in that even Linn, Clark & Slotta (2003) caution that its level of detail affects student engagement. The prescriptive nature of WISE projects provide students with the necessary information to proceed independently, but also provide opportunities for teachers to misinterpret the structure of the investigation. Manipulating the available scaffolding steps along with the limited opportunities for socially constructing knowledge embedded within WISE provide a potential recipe for reinforcing the transmission model, albeit with animations and the technological affordances of accessing past progress. While the Jasper Series was founded on stronger pedagogical principles that provide valuable insight into TELEs and continue to describe essential qualities of powerful and effective learning environments, both it and WISE promote more of a packaged approach to inquiry that does not require teachers to explicitly understand the theory and pedagogy behind them before integrating them. As potent as they could be in bringing inquiry-based learning to the classroom, they could also be used to further entrench traditional instructional approaches that reinforce inert knowledge. It cannot be assumed that teachers possess the aptitude to integrate these TELEs. Just as students require explicit instruction to develop inquiry skills, teachers need to be “explicitly taught about interactions among pedagogy, content, technology, and learners” to develop their Technological Pedagogical Content Knowledge, or TPCK. This conceptualization is critical.

The Learning for Use design framework and T-GEM cycle of instruction, originally attached to My World and Chemland TELEs, offer the greatest potential for reform in the mathematics and science classroom. With a primary emphasis on the inquiry process rather than prescribed activity steps, it requires teachers and students to adopt an inquiry mind-set that becomes the foundation for implementing them. They are not distinctly tied to one particular curricular area or TELE, offering transportability to any number of educational contexts, within the classroom or outside of it. Their cyclical nature and use of abductive reasoning puts greater emphasis on the relationships between students and between students and the teacher highlighting the role social collaboration and collective understanding plays in the development of robust mental models that can help students conceptualize content and repair misconceptions. Understanding this pedagogy requires teachers to pursue a pedagogical model that exemplifies the development and refinement of useful and adaptive pedagogical knowledge because inert knowledge or memorization of a set of activities in an effort to apply either of these methods will not suffice. The broad scope of these two approaches compel educators to seek knowledge for understanding.

Integrating constructivist pedagogy into classroom practice is not a simple process. “The constructivist theories of learning apply to teachers and designers” as well as students (Edelson, 2001, p. 381). If teachers are going to be successful implement the Learning for Use framework or T-GEM instructional cycles, it is imperative that have parallel experiences with this learning process themselves to model best practice and become co-learners with students in a continued process of reflection and refinement.

image: Walking the line by Kalexanderson released under a CC Attribution – Noncommercial – Share Alike license

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching, 38(3), 355-385.

Edelson, D., Salierno, C., Matese, G., Pitts, V. & Sherin, B. (2002). Learning-for-use in Earth Science: Kids as climate modelers. Paper presented at the Annual Meeting of the National Association for Research in Science Teaching, New Orleans, LA.

Hatano, G. & Inagaki, K. (1984). Two courses of expertise. Research and Clinical Center for Child Development Annual Report, 6, 27-36. Retrieved from http://eprints2008.lib.hokudai.ac.jp/dspace/bitstream/2115/25206/1/6_P27-36.pdfbe

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905.

Khan, S. (2010). New pedagogies for teaching with computer simulations. Journal of Science Education and Technology, 20(3), 215-232.

Linn, M. Clark, D. & Slotta, J. (2003). WISE design for Knowledge Integration. Science Education, 87(4), 517-538.

Pellegrino, J.W. & Brophy, S. (2008). From cognitive theory to instructional practice: Technology and the evolution of anchored instruction. In Ifenthaler, Pirney-Dunner, & J.M. Spector (Eds.) Understanding models for learning and instruction, New York: Springer Science + Business Media, pp. 277-303.

Assessing the Affordances of TELEs

Posted on by
Reply

Anchored instruction in the Jasper Series, WISE’s scaffolded knowledge integration framework (SKI), the Learning for Use model when applied to My World, and applying the T-GEM cycle to Chemland explorations showcase the application of pedagogical design in response to ongoing research regarding effective technology-enhanced learning experiences (TELE) in mathematics and science classrooms. All four TELEs are driven by documented discrepancies between theoretical best practice and actual instructional approaches in all levels of education. Although varied in their application, each design is grounded in constructivist principles that focus on inquiry-based learning, mental models, socially constructed knowledge, and reflective conceptualization aimed at integrating both content and process outcomes of science or mathematics education. Reasons for pursuing this common pedagogical design are rooted in substantive conclusions of researchers who assert that “inquiry is associated with an array of positive student outcomes, such as growth in conceptual understanding, increased understanding of the nature of science, and development of research skills” (Khan, 2007, p.877). To achieve this authenticity within TELEs the design must be nourished by activities that “provide the opportunity to ground abstract understanding in concrete experience” (Edelson, 2001, p. 378). Reforming science and mathematics requires a pedagogical shift away from the passive “transmission approach [which] does not acknowledge the importance of the motivation and refinement stages of learning and relies too strongly on communication to support knowledge construction” (Edelson, 2001, p. 377).

While the tenets of How People Learn are most prominently applied to the Jasper Series and the development of anchored instruction, emphasis on pedagogically sound learning environments that embrace knowledge, learner, assessment, and community-centered principles is also woven into the pedagogical approaches attached to WISE, My World and Chemland. The degree to which each of these aspects are incorporated into the design structure of these TELEs varies, although all demonstrate a more concerted effort towards being knowledge and learner-centered beyond assessment and community-centered. The perceived authenticity of the inquiry plays a significant role in developing science process skills, conceptualization of content skills, and students’ connection with relevance of math and science outside of the classroom. All four TELEs strive to create an environment that promotes and nurtures learning from inquiry, as well as underscoring the importance of the facilitator’s role from a pedagogical perspective as technology is unable to independently and meaningfully guide students through this process.

Authenticity of the inquiry process is best illustrated in the Learning for Use framework and T-GEM cycle – each having potential in educational settings well beyond My World and Chemland. Investigating these two pedagogical approaches reveal a process-based structure that is emergent and tailored to students in a specific setting. Both offer cognitive and social affordances in learner-centered environments that move beyond the pre-packaged options of the Jasper Series or WISE projects. The depth of conceptualization possible in TELEs designed using these pedagogies provide students and teachers with an inquiry process that develops authentic problem solving skills, robust thinking skills and reflective practice. Every stage of the inquiry process is integral and must unfold explicitly for students if they are expected to develop effective knowledge organization indices that can be accessed in the future. Understanding the principles behind Learning for Use and T-GEM requires a broader comprehension of constructivism, situated cognition, abductive reasoning and inquiry-based learning. Implementing these approaches in a classroom involves the application of a holistic process that encompasses more than specific activity guidelines or steps, providing students with greater opportunities for skill transfer and improving teacher heuristics within technology supported inquiry learning (TSIL). The cyclical nature of Learning for Use and T-GEM parallels authentic inquiry in the scientific community and strengthens students’ abilities to evaluate and refine mental models as part of the process of abstraction. For successful integration, teachers must possess in-depth knowledge of their students and the ability to promote students’ gradual construction of knowledge individually and collectively.

“computer simulations are particularly valuable for science teachers because they help students visualize aspects of science that are either too large or too small to view, afford rapid testing of ideas, reveal trends in graphs or other representations, and provide extreme situations to support thought experiments and what if scenarios” (Khan, 2010, p.216)

Exploring these TELEs has created an increased impetus for reflecting on my own integration of computer simulations and technology enhanced learning experiences in my practice. Being able to better articulate my pedagogical approach in specific educational contexts and analyze how I am using technology to support students’ development of authentic inquiry processes has strengthened my TPCK, which in turn will strengthen my ability to design knowledge, learner, community, and assessment-centered learning environments that promote inquiry and conceptualization. T-GEM and Learning for Use pedagogy will be valuable resources in designing the inquiry-based classroom I envision. The scaffolded knowledge integration framework and anchored instruction principles have contributed to an increased understanding of inquiry-based learning and enriched my instructional design principles which will in turn positively impact my current and future practice. The limitations observed in the WISE project design have challenged my perception of how best to approach teaching inquiry using technology because a one-size fits all model, transmitting incremental procedural steps, is inadequate. I believe teachers need to carefully gauge students’ inquiry skills to determine authenticity or if they are merely witnessing the appearance of authenticity in the wake of poorly designed or poorly implemented pedagogy. First and foremost though, this necessitates a depth of understanding involving inquiry-based learning from an educator’s perspective that cannot be underestimated.

 image: Thinking by heyjudegallery released under a CC Attribution – Share Alike license

 

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching, 38(3), 355-385.

Edelson, D., Salierno, C., Matese, G., Pitts, V. & Sherin, B. (2002). Learning-for-use in Earth Science: Kids as climate modelers. Paper presented at the Annual Meeting of the National Association for Research in Science Teaching, New Orleans, LA.

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905.

Khan, S. (2010). New pedagogies for teaching with computer simulations. Journal of Science Education and Technology, 20(3), 215-232.

Linn, M. Clark, D. & Slotta, J. (2003). WISE design for Knowledge Integration. Science Education, 87(4), 517-538.

Pellegrino, J.W. & Brophy, S. (2008). From cognitive theory to instructional practice: Technology and the evolution of anchored instruction. In Ifenthaler, Pirney-Dunner, & J.M. Spector (Eds.) Understanding models for learning and instruction, New York: Springer Science + Business Media, pp. 277-303.

 

Zooniverse: Citizen Scientists

Posted on by
Reply

The first Zooniverse project was unveiled by the Citizen Science Alliance in 2007, but has substantially grown since then. Their projects rely on the efforts of people around the world, including many different classrooms, to collect and organize data on a specific research question. This results in extensive information that scientists and researchers have used to further their investigations in the scientific community. Unique scientific discoveries have been attributed to individual and collective contributions demonstrating the authenticity of the scientific process embedded in the projects.

The Citizen Science Alliance represents many different academic and professional perspectives from education, astronomy, and technology fields. Together they are committed to developing educational experiences in Zooniverse that foster inquiry in science. Their objectives include:

  • Education Research
  • Content development and tools to facilitate its navigation so visitors can carry out their own investigations using their data
  • Expanding options for interactions with Zooniverse beyond personal computer, such as mobile devices and museums

Technology has become a valuable component of science research for their in collecting and analyzing data as well as modeling and sharing research results. As Edelson (2001) indicates, “any effort to engage students in authentic scientific practices should reflect this trend” with technology. Zooniverse is an excellent resource that can promote inquiry-based learning in the classroom while situating the learning experience in genuine research. Within each project, the research process is modeled authentically and students gain experience in a realistic setting that connects them with scientists who provide purpose in sharing how the data contributed will ultimately help answer the research question. Initiating students in the introductory activities are set to motivate students so the recognize the need for new knowledge before they proceed. Cognitive affordances are observed in activities involving both scientific content and process relating directly to the research topic. Project designs also facilitate students` perception of scientists in their fields of expertise. The topics investigated in Zooniverse are scientific phenomena that cannot easily be observed. Integration of photographs, video, and interactive simulations provide students with scaled models to help students’ conceptualization. Social affordances are rooted in opportunities to collaborate in detection groups asynchronously with global audience members within the same project as well as share their thoughts and queries in connected discussion forums. Students can keep track of what they have contributed and how they have networked with other individuals during their inquiry process by using the “My Zooniverse” feature.

Some of the projects have specific teacher resources attached to them to provide teachers with the necessary information and tools to support and extend students’ learning. Solar Stormwatch is one such activity, and while this additional resource is not available in all projects, Zooniverse is in the process of adding teacher resources to more projects as they realize the potential educational benefits inherent in their overall design. Opportunities abound for integrating the science content within this site into other academic areas including language arts, math, and social studies.

 

References

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching, 38(3), 355-385.

Pellegrino, J.W. & Brophy, S. (2008). From cognitive theory to instructional practice: Technology and the evolution of anchored instruction. In Ifenthaler, Pirney-Dunner, & J.M. Spector (Eds.) Understanding models for learning and instruction, New York: Springer Science + Business Media, pp. 277-303.

 

T-GEM Possibilities …

Posted on by
Reply

After reading the articles this week, I find myself contemplating GEM in my planning … a lot. I am excited to try and implement this model of inquiry as it seems to be what I’ve been looking for. While I think I have been incorporating aspects of it already, it has given me a better foundation to reflect on my efforts to help my students develop key processes of inquiry, not just in science and math either … everywhere. I realize I need to put more effort into using “modeling and inquiry [to] facilitate the development and revision of abstract concepts” (Kahn, 899) in my classroom.

In Grade 7, students are expected to understand properties of matter. It can be a difficult concept for some as the abstract notion of particles and molecules aren’t readily observable. Creating enough hands-on activities to demonstrate this is also a challenge because changes involve increases and decreases in temperature, which produce safety concerns and impinge on time factors. A computer simulation can help students understand this concept beyond text-book facts and static images.

To begin with we would investigate quantitative and qualitative properties of matter, so students would be introduced to the concepts of solids, liquids, gases and particles as well as the rationale behind temperature being measured in kelvins. This would provide the appropriate background information before engaging in the PHET simulation – Properties of Matter.

GENERATE
Students are asked to collect data on neon and its particles in the 3 different states of matter. The first relationship they are asked to generate is how the particles compare to each other in each state.

EVALUATE
They need to test their hypothesis by investigating 2 other substances, argon and oxygen, in their different states of matter.

RE-GENERATE/RE-EVALUATE
Students collect further data by documenting temperature observed at each state with each substance. They are then asked to explain and test the relationship between temperature and the energy of the molecules, using the option of heating or cooling the substance.

EVALUATE/MODIFY
Finally, water is investigated to see if their theories of particle movement and interaction in relation to temperature continue to be valid. Students will be guided toward the solid model of water to investigate its particles further, its response to further cooling, and asked to evaluate/modify their assumptions about particles within solids – leading towards an understanding of the properties of ice compared to other solid substances.

Further investigations ~ this would extend into the next science class

Students use the temperature data they have collected to investigate what happens to different substances when they are heated or cooled to the same temperature observed by another substance in a particular state. E.g. Neon is observed in a gas state at 55 K … what does argon look at 55 K? What state of matter is most likely represented at this temperature?

Students are asked to comparatively investigate the substances and determine the impact variations of temperature would have on these substances according to a scale of temperature. E.g. Investigating boiling points, melting points and freezing points.

image: Beaded Molecules: preliminary tries by fdecomite released under a CC Attribution license

References

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905.

Khan, S. (2010). New pedagogies for teaching with computer simulations. Journal of Science Education and Technology, 20(3), 215-232.

 

Technology Affordances in Earth Science Education

Posted on by
Reply

Concepts in Earth Science can be challenging for students to grasp as real examples relating to curricular content are often difficult or simply cannot be brought into the classroom to provide students with first-hand experience with them. Size and accessibility are factors which compromise students’ abilities to form mental models that accurately reflect scale, so comparative models are often relied on in place of actual phenomena or their processes. For students to conceptualize these appropriately, spatial-thinking and scale must be understood which requires abstract reasoning that teachers cannot presume is already present. Lack of opportunities to collect first-hand data presents an additional problem, which results in an over-reliance on data banks that detract from the authentic mirroring of processes within the scientific community.

Technology offers innovative means of exploring Earth Science phenomena through computer-generated simulations and models as well as methods of data collection, data analysis, and ways of communicating scientific research (Edelson, 2001). It is a component of authentic scientific practice reinforcing its inclusion in classrooms, and considering its potential as a catalyst for educational reform, devising specific uses of computers to bridge content and process standards in science may provide educators with a sustainable approach for technology integration. It can also enhance the inquiry process by breaking down the walls of the classroom to connect with information and individuals worldwide, store content for future use or reflection, and present student learning to both a local and global audience synchronously or asynchronously.

With WorldWatcher being designed to “bring the power of scientists’ computational tools to learners (Gordin & Pea in Edelson, 2001), it presents an authentic learning environment in which students can develop inquiry skills through a scientific research process. Using data visualization and tools for analysis, students can explore Earth science phenomena and identify emerging patterns in data using scaled models. This provides a feasible solution to the challenge of students accessing realistic representations of the Earth by providing a window into understanding complex phenomena that students are known to develop misconceptions about. Not only do students have the opportunity to work with data collected by the scientific community in WorldWatcher, they’re also presented with the chance to apply their understanding from previous scaffolded lessons as they create and collect their own geographic data to further investigate the relationship between geography and temperature. This technology merges practical and realistic scientific inquiry-based pedagogy that motivate students to construct and refine knowledge that “support its future retrieval and use” (lEdeslon, 2001) and students’ ability to transfer this useful knowledge to new contexts.

image: Earth from Space by NASA Goddard Photo and Video released under a CC Attribution license

References

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching, 38(3), 355-385.

Edelson, D., Salierno, C., Matese, G., Pitts, V. & Sherin, B. (2002). Learning-for-use in Earth Science: Kids as climate modelers. Paper presented at the Annual Meeting of the National Association for Research in Science Teaching, New Orleans, LA.

Implementing WISE

Posted on by
Reply

Throughout my exploration of WISE, I entertained thoughts about how it could be integrated into the classroom. The animations and simulations embedded within the projects definitely have the potential to the enrich learning environment for students, but its promise of helping educators “create sustainable classroom inquiry instruction across the varied contexts learning takes place” (Linn, M., Clark, D. & Slotta, J., 2003) was not substantive based on the activities I had the chance to explore; however, I’m not ruling out that I have yet to tap into existing projects whose pedagogy would in fact impress me. I believe WISE has the potential to motivate students and facilitate knowledge integration, but as a constructivist portal for inquiry-based instruction, this platform leaves too much room for educators to copy and revise projects without staying committed to the pedagogy that WISE developers set out to promote with its design. Discovering examples such as this has made me skeptic of its universal benefits in classrooms and its potential role in developing inquiry skills if educators are not guided and scaffolded themselves in learning how to create and refine inquiry-based pedagogy. As Edelson (2001) points out that “the constructivist theories of learning apply to teachers and designers as well … [so] if they are to learn to use it successfully, they must go through a learning process themselves.” The effectiveness of technology integration is always determined by the hands who wield it. If WISE had more influence on teachers’ professional development to better ensure it was used in accordance with robust inquiry principles, it could do more to reform science education.

As it stands, I think WISE is best integrated with other means of instruction with or without  additional technology, face to face interactions in classrooms or within a distributed learning context. From my observations, I question the strength of the Scaffolded Knowledge Integration framework tenet, “helping students learn from each other” within the WISE context. Facilitating social opportunities that promote collaboration, peer feedback, and perspective taking are noticeably minimal in the projects I perused – another reason to  integrate it within a larger body of instruction so students can take advantage of the social nature of learning. WISE, as I see it, should not be implemented by educators  as a stand-alone unit. It must be supplemented in the best interests of learning.

image: IMG_4950 by bionic released under a CC Attribuition – Noncommecial license

 References

Linn, M. Clark, D. & Slotta, J. (2003). WISE design for Knowledge Integration. Science Education, 87(4), 517-538.

Nicaise, M., Gibney, T. & Crane, M. (2000). Toward an understanding of authentic learning: student perception of an authentic classroom. Journal of Science Education and Technology, 9(1), 79-94, doi: 10.1023/A:1009477008671

 

WISE Foundations vs Application of Inquiry

Posted on by
Reply

Although the motivation exists, building inquiry into the science classroom to better mirror realistic scientific discovery has been hampered by the need to reach a plethora of curriculum standards. The motivation behind the development of WISE is derived from the desire to remedy this. Linn, Clark & Sotta (2003) define inquiry as “engaging students in the intentional process of diagnosing problems, critiquing experiments, distinguishing alternatives, planning investigations, revising views, researching conjectures, searching for information, constructing models, debating with peers, communicating to diverse audiences, and forming coherent arguments” (Linn, Clark & Slotta, 2003). Through the use of a revised Scaffolded Knowledge Integration framework and its four main tenets (making thinking visible, making science accessible, helping students learn from each other, and promoting lifelong learning), this was a much needed shift towards inquiry-based learning positioning WISE as an innovator in the field of science education.

With these design principles in mind, my exploration of some of the projects within WISE has prompted more inquiry into how it might be used in a classroom, the thinking and understanding it encourages in students, and the overall instructional design as it applies to learning theory. The theory behind the design offers great potential for Vygotsky-inspired social constructivist activities in that the intent is to provide students with a model that promotes meaning making and the construction of knowledge along with learning with and from others, as well as teacher scaffolding that includes the introduction of necessary cognitive tools . A deeper analysis  of an independently chosen project – Photosynthesis 2012 – uncovered a number of issues I feel may become obstacles to WISE’s progress towards meeting social constructivist goals. Given the evolution of the possible online learning spaces, WISE appears very linear in nature (more like an LMS), fairly dependent on a text-based medium (i.e. understanding is demonstrated primarily through reading and writing activities similar to pieces of worksheets), and offers limited opportunities for collaborative and social learning. In reading the Quick Start Guide and the Teaching Tips available within the project overviews, it appears that one of the founding principles, “helping students learn from each other”, is primarily addressed within teacher-designed activities external to the WISE project. Teachers are encouraged to group students in pairs during the project process, structure discussion and discourse within the classroom, and integrate group/partner activities to meet the goal of socially constructed learning. WISE offers an asynchronous discussion forum that can be a powerful medium for sharing and reflecting on student perspectives, but it seems to fall short of embedding truly collaborative opportunities. In the case of the photosynthesis project that I selected to revise, not one asynchronous discussion activity had been included. Students seem to continue to be on the consuming end rather than the producing end and while the foundation for knowledge integration is present in WISE, what’s stopping a teacher from using it to perpetuate a transmission model of learning? Because it is possible to revise projects for this purpose as well. Online learning environments like WISE have incredible potential to redefine students’ classroom experiences, but they are only as effective as the individuals using and adapting them for their own pedagogical use. Developers envision  a more critical approach to science process and concepts being implemented using the WISE, but as the educational philosopher Paulo Freire pointed out “computers were not technologically determined to compel students to use them in a critically conscious manner”  (Papert in Kahn & Kellner, 2007), so how well WISE helps students develop inquiry skills is dependent on the individual designing it and the individual wielding it. Likewise, Ivan Illich’s cautionary advice  that “technologies like computers could either advance or distort pedagogy, depending on how they were fit into a well-balanced ecology of learning” (Kahn & Kellner, 2007) is an integral component of WISE’s future success in bringing a greater degree of inquiry into the science classroom.

Using the Authoring Tool, I explored the inner framework of the Photosynthesis 2012 project after saving a copy of it so I could edit and revise it as my own. My version has been renamed Exploring Photosynthesis. As I investigated the three activities that each included multiple steps, I made improvements to ambiguous language and sentence structure. I also altered the html code to add more text features, like bolded and italicized words as well as bulleted lists to separate ideas. I was also able to locate the code for the hover text for glossary words so I added it into the first page where it suggested students explore an example of it, but no example was to be found. I felt it was necessary to embed a short video in Step 1.6 as this form of media had not been previously included within other steps, yet it stands to enrich the options for presenting critical information to students. I was surprised to find that video wasn’t used more often, although there are far more projects within the WISE pool than I had time to explore. I was heartened to discover an audio tool within the Extras of the Authoring Tool, but I was disheartened to find that I was unable to access it to see what it offers. When considering diversified instruction, balancing the text with audio components would meet more students needs and potentially minimize the barrier of language that can impede conceptualization for some students. I also found that some steps lacked sufficient information for students without considerable background knowledge (even if the intent was to cover some concepts during class) to proceed and be motivated to innately inquire further. I was happy to see that the steps involving MySystem technology offered students opportunities to revise and apply feedback as well as potentially share their understanding with others if this is enabled. I intend to investigate this option further to get a better grasp of its benefits. With this particular project, I found instructional strategies frequently utilized that seemed to focus on recall, which could be improved on while still maintaining the scaffolding principle that is an integral component of WISE. Effort had been made to scaffold the acquisition of content; however, the development of inquiry skills, which was the underlying goal of this environment, was not adequately supported in a manner that provides students with opportunities to “improve their art and technique of inquiry” (Nicaise, Gibney, & Crane, 2000) through repeated and explicit practice. There are opportunities to use inquiry skills within the steps, but  without initial steps that help students hone inquiry skills and deepen their motivation to inquire more. While I haven’t added the extra step yet, I feel it’s important to ask students about what questions they need to ask and be able to answer if the intention is to discover the best method of growing energy-rich plants. Knowing how to formulate “good” questions is a critical skill in the inquiry process. I also found it interesting that the initial inquiry question had minimal impact at the conclusion of the project. Emphasis was not placed on presenting their understanding to peers or the teacher regarding how they can help Mary. My subsequent investigations and revisions will hopefully ascertain whether this is a grievous oversight or an inadequate inquiry question that can be revised and strengthened to promote greater learning. Time will also tell if these first impressions are substantiated by my future investigations into alternate projects or if the developers of WISE are steadfast in their desire to continue revising and collecting data on the effectiveness of this learning environment in the pursuit of meaningful inquiry-based pedagogy.


image: Why by Tintin44 – Sylvain Masson released under a CC Attribution – Noncommercial – No Derivatives Works license

 

 References

Kahn, R. & Kellner, D. (2007). Paulo Freire and Ivan Illich: technology, politics and the reconstruction of education. Policy Futures in Education, 5(4), 431-448. doi:10.2304/pfie.2007.5.4.431

Linn, M. Clark, D. & Slotta, J. (2003). WISE design for Knowledge Integration. Science Education, 87(4), 517-538.

Nicaise, M., Gibney, T. & Crane, M. (2000). Toward an understanding of authentic learning: student perception of an authentic classroom. Journal of Science Education and Technology, 9(1), 79-94, doi: 10.1023/A:1009477008671