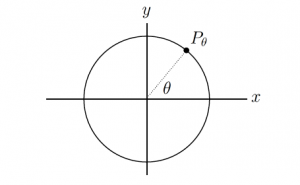
We’ve worked on the Intermediate Value Theorem (I’ll call it IVT in rest of my article) recently, according to the image, here goes a problem about IVT application, its mainly idea is to prove there exist a antipodal of P0

.

When convincing people who has no background knowledge about IVT, we can use the airplane flying trajectory  to clarify:

When a plane have to fly from Southern Hemisphere to Northern Hemisphere like Australia to Canada, no matter what trajectory it would follow, that plane will definitely fly through the Equator. When drawing a picture of what I’ve presented, the airplane’s trajectory will go across the equator no matter what.

We can suppose that Australia is point a and Canada is point c, which correspond to interval (a, b), it is continuous. When connecting it to a xy-axis(y is the equator) graph, that interval can have a value interval (f(a), f(b)), according to this, the cross point of flying trajectory and equator is the c in interval (a, b), it has a value f(c)=0 and in the interval of (f(a), f(b)).

Mathematically speaking, the IVT definition is:

When a function is continuous, we can pick a c in the interval of [a, b], and there exists a f(c)=L in the interval of [f(a), f(b)].

Recently, we’ve worked on some problems about sequence converges and series converges. For certainty, there are some expressive sequences and series that I want to discuss.

We say that {an} is Cauchy  if an and am are arbitrarily close to each other provided n and m are sufficiently large.

This is how Professor Leung described in our pre-reading assignment. Cauchy sequence is a converge sequence which also has a converge subsequence. We can find that everywhere, we describing a diagram, composing a stock curve etc. Cauchy sequence applies in every part of our life especially in software and finance.

When considering a series, I will definitely link it with the annual saving rates of commercial bank. Longer the time you save your money in it, higher the saving rates you can get, just like a adding series.

Every time we playing sport like basketball, we could probably found out that after we throwing the basketball, flying trajectory was like a perfect curve function, which has a horizontal asymptote.