Explanation:

According to the definition of antiderivative and integration, antiderivative is the opposite process of derivative and it was introduced when we discussed the Fundamental theorem of calculus, if the F(x) is the antiderivative of f(x), then the integration of f(x) from a to b equals to the value of F(b) subtract the value of F(a). So basically, the anti-derivative of a function(a) is finding where the function(a) derivative from. In the sense that if function(b) experience derivative can get function(a), so that function(b) is the antiderivative of function(a). Besides the antiderivative is also been called indefinite integrals.

And for the integration, it is a basic and fundamental part of calculus and this includes different types of integrals. We started to learn this from Riemann integration, which is known as definite integral. And this definite integral will end up with a number.

In terms of whether a function have antiderivative or not integral or not, if the function is not continuous they don’t have antiderivative. But it may still do integration in terms of definite integral.

Example:

In physics, the time-speed question is a really great example to explain the definitions of antiderivative and integration.

For example:

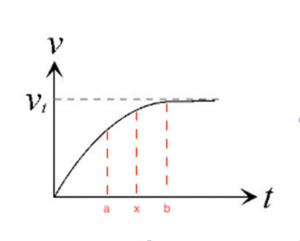
To calculate the distance from time a to b, we need to use the definition of integration. Since a and b are two constants with certain values. From formula d’(t)=v(t),

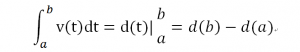
While if we want to express the distance from time a to an uncertain x, the expression of distance is calculated by antiderivative.

Since x is not fixed, the antiderivative of v(t) from time a to x should be d(t). To be more specific, if v(t)=, by the definition of antiderivative , we can get: d(t).

In this way, we can explain the difference between antiderivative and integration.