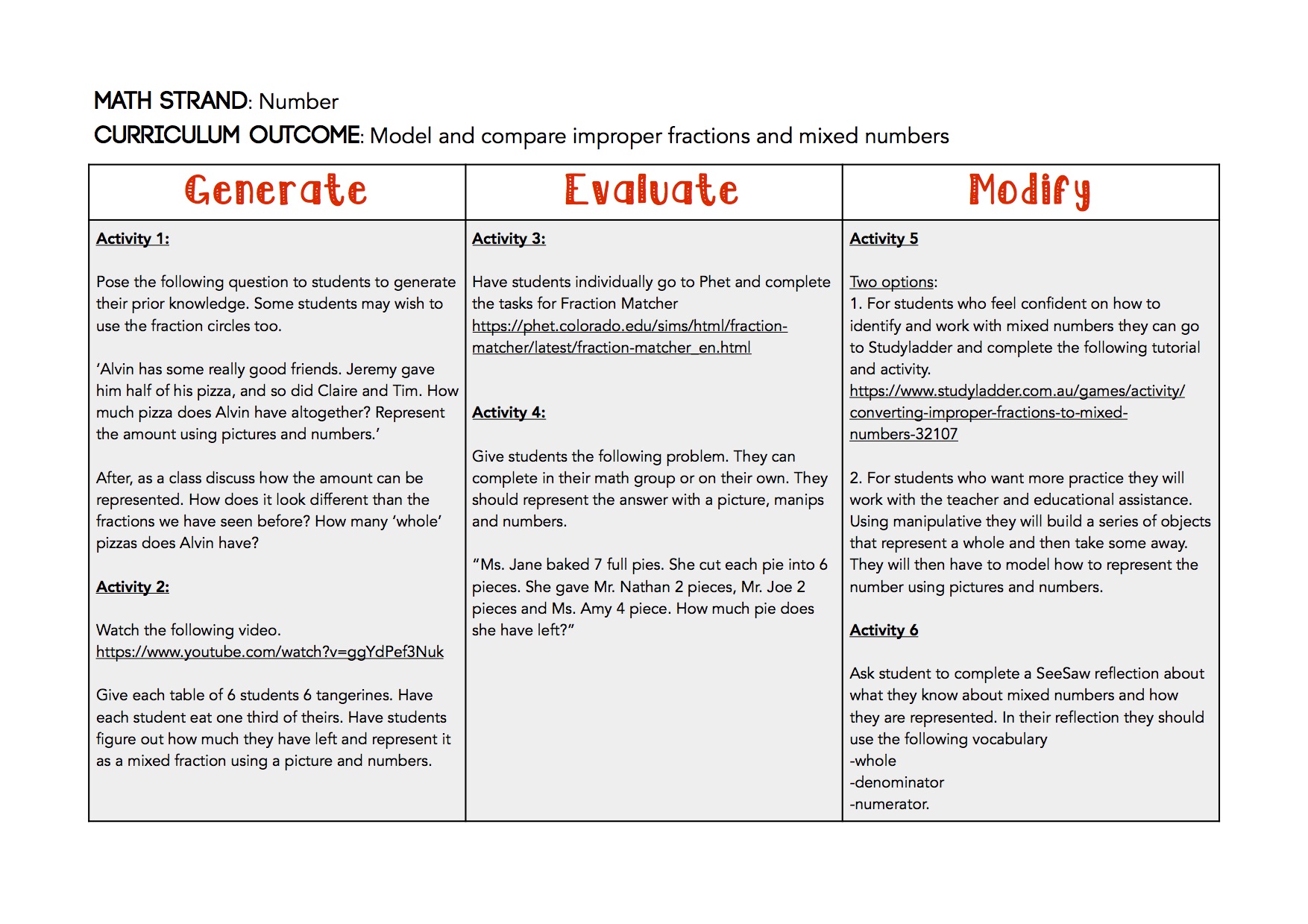
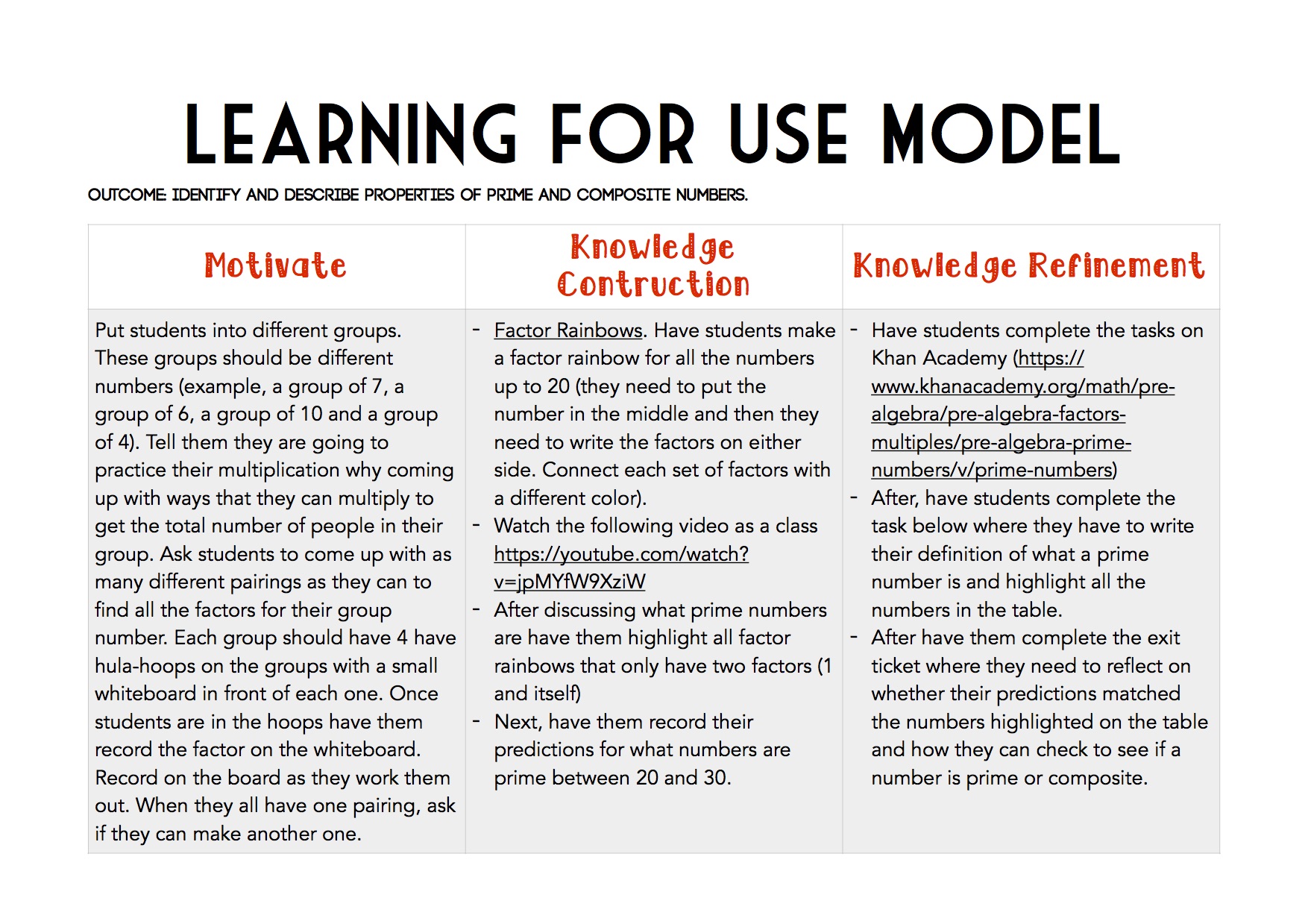
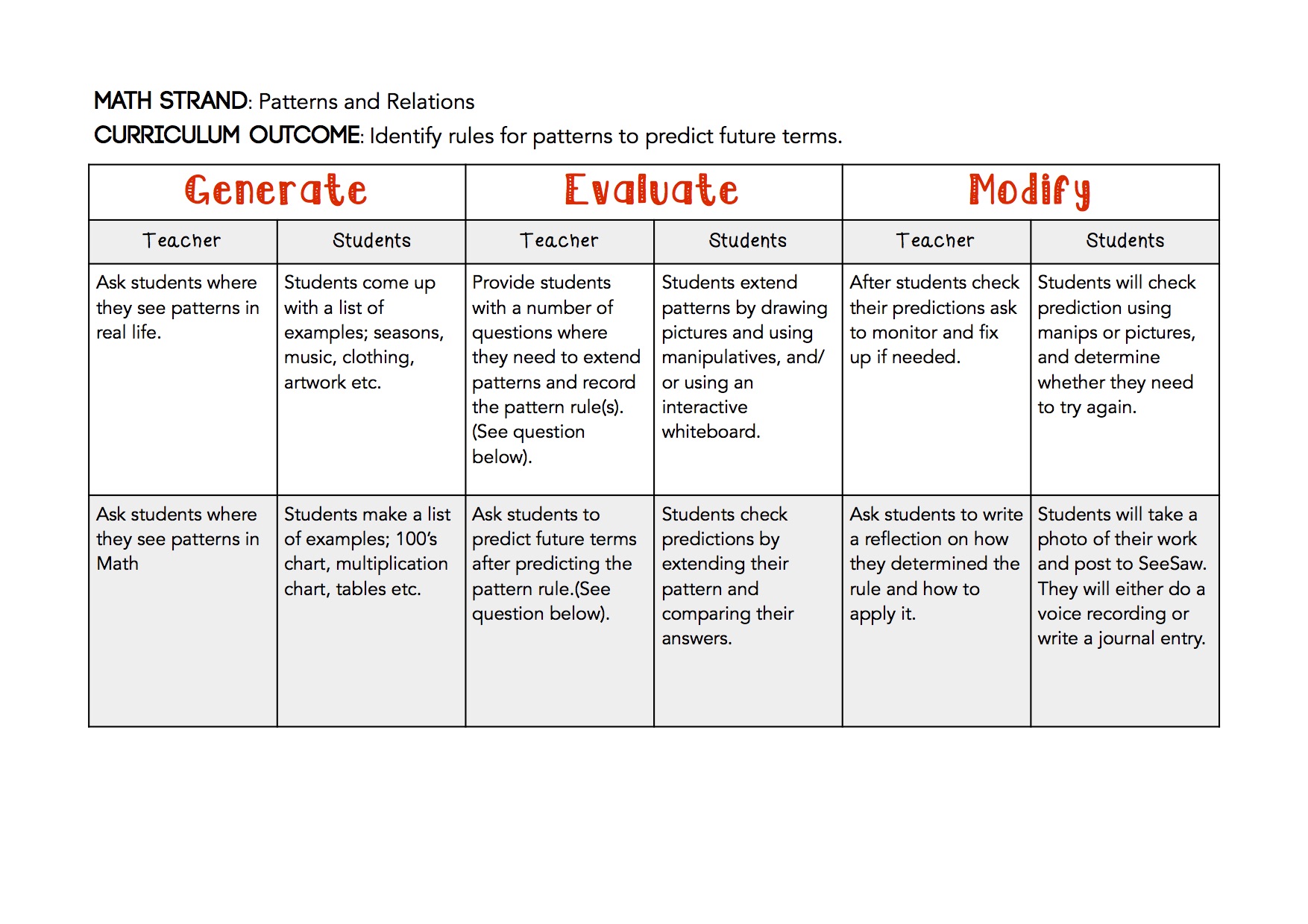
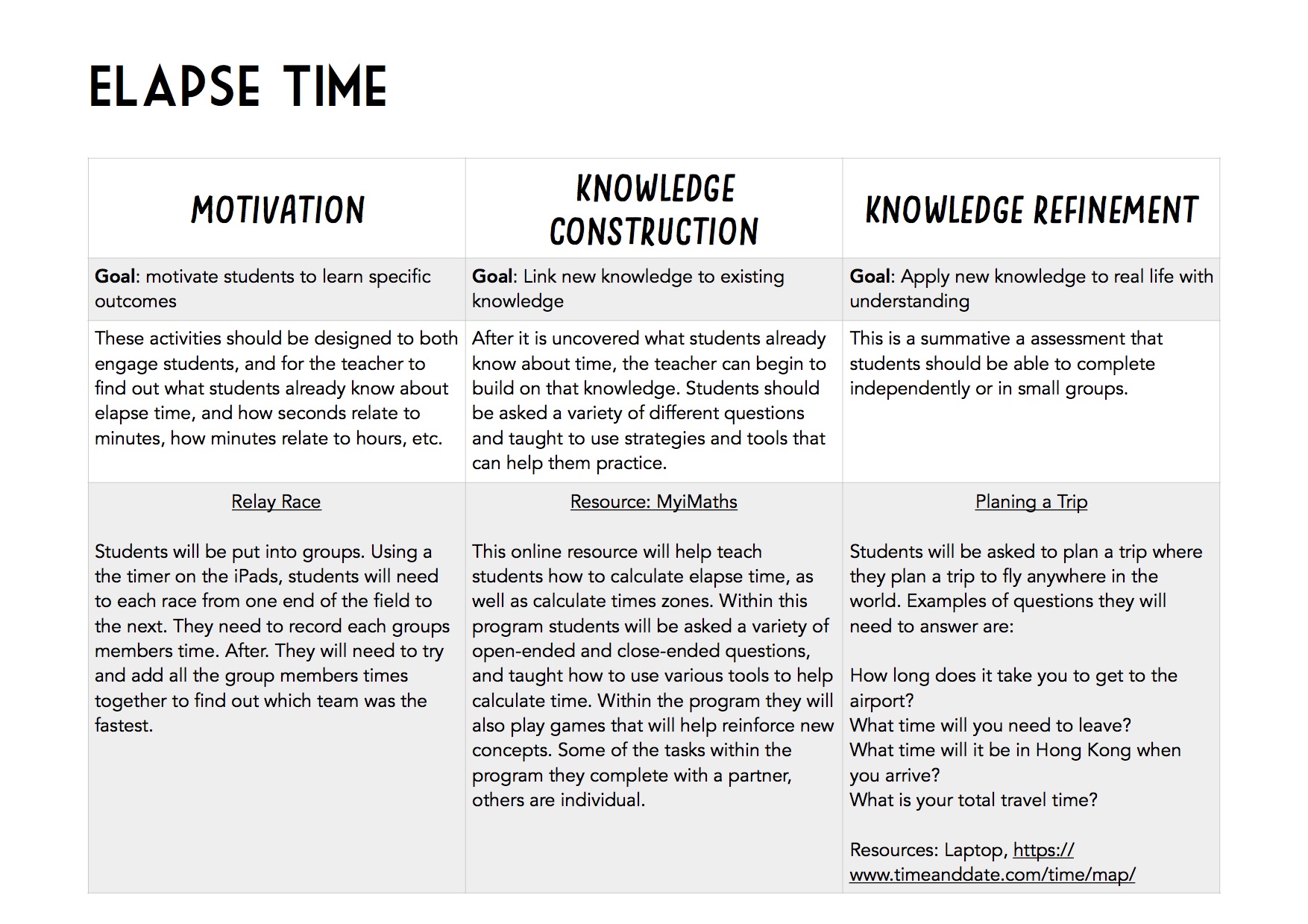
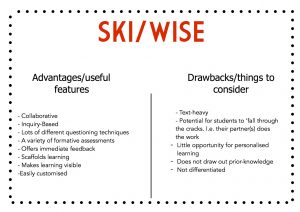
Author Archives: sarah fitzpatrick

Information Visualization Reflection

Like any of the technologies we have explored so far in this course, when planning, teachers need to be thinking about how the technology will enhance and redefine the learning for their students. In order to do this teachers need to have a good grasp on PCK and TPCK in their subject area. When looking at using simulations teachers need to ask themselves what the purpose of using them is? Is this technology going to give students an experience that they otherwise wouldn’t be able to get? Or is it being used to reinforce or practice a concept? As Stephens & Clements (2015) mention “Because simulations are intended to convey dynamic visual information, teachers may be tempted to believe that simulations are automatically effective in communicating complex models to students.” Teachers can’t assume that students will understand the material in a simulation and need to carefully plan pre-assessments as well as ongoing formative assessments to ensure that students are on track and not falling behind by missing crucial information.

Simulations have advantages when used carefully and intentionally. They can give student opportunities they wouldn’t otherwise receive, help students practice and reinforce concepts previously learned, motivate students and keep them engaged, as well as help them make connections to the outside world. Before using simulations, however, teachers need to ensure that students have appropriate prior-knowledge. Stephens & Clements (2015) and Srinvasan et al. (2006), all reinforce this idea, and it is crucial for teachers to plan appropriate pre-assessments as well as explicit lessons to teach a concept before using a simulation. When using PhET for example, it would be ineffective for students to use this resource without having any prior knowledge or experience with the materials in the modules. Most students would have a difficult time navigating and accessing the content and may become disengaged and frustrating, losing the motivation to learn. Finkelstein et al. (2005) discuss that useful simulations should be designed to be interactive, engaging and highly visual which can help students make connections with the content to their everyday life. When adequately scaffolded, PhET has the potential to do just this, but teachers need to ensure that students are prepared and have enough understanding to complete the tasks independently.

After completing this week’s readings and going through my classmate’s posts, I am more motivated to use different simulation in my class when possible. I have to admit that I am guilty of using them right now as a ‘filler’ task for students to complete when they finish a task early. For instance, I’ll tell students who finish 5 or 10 minutes before others to hop on to Mathletics and complete some assigned tasks in their folder. Using simulations more intentionally and carefully can have many benefits, I think it takes time to plan how you will introduce them and keep track of students progress. Planning them within any TELE framework can help teachers see when to use them, as well as how to scaffold the learning approproaitely so they are introducing them at a time which will be most effective.

References:

Finkelstein, N.D., Perkins, K.K., Adams, W., Kohl, P., & Podolefsky, N. (2005). When learning about the real world is better done virtually: A study of substituting computer simulations for laboratory equipment. Physics Education Research,1(1), 1-8.

Srinivasan, S., Perez, L. C., Palmer,R., Brooks,D., Wilson,K., & Fowler. D. (2006). Reality versus simulation. Journal of Science Education and Technology, 15(2), 137-141.

Stephens, A. & Clement, J. (2015). Use of physics simulations in whole class and small group settings: Comparative case studies. Computers & Education, 86, 137-156.

Mixed Numbers & Mixed Simulations

This week I decided to use the T-Gem model to create a lesson on mixed numbers as a way to build on students knowledge of fractions. In a fractions unit before moving onto mixed numbers students should have a strong understanding that a fraction is part of a whole. When students begin to learn about mixed numbers and improper fractions they often struggle to see what they mean conceptually as they have spent so much time learning that fractions are part of a whole.

As Stephen and Clements (2015) mention, before using any simulations students should have adequate background knowledge. Therefore, I added a simulation activity in the Evaluating Knowledge and Modifying Knowledge sections. The first activity in Generating Knowledge is a way for teachers to assess what students know already about mixed numbers and how to represent them, followed by an activity where the class can unpack what they mean together. Next is when they move to apply what they learned in the simulation activity followed by a reflection.

Srinivasan et al. (2006) discuss how simulations are compelling and can help motivate students but you cannot simply tell students that simulations are the same as real life. Students need to be able to apply their knowledge in real-life contexts as well. Therefore, to build on these activities, I would blend more simulations using programs like Mathletics and Khan Academy with hands-on materials like fraction towers, fraction circles, etc. Once students reach a strong conceptual understanding, my ideal summative tasks would involve a real-life application such as using money.

T-GEM & Mixed Numbers

References:

Srinivasan, S., Perez, L. C., Palmer,R., Brooks,D., Wilson,K., & Fowler. D. (2006). Reality versus simulation. Journal of Science Education and Technology, 15(2), 137-141.

Stephens, A. & Clement, J. (2015). Use of physics simulations in whole class and small group settings: Comparative case studies. Computers & Education, 86, 137-156.

Informal Learning Spaces

▪ How is knowledge relevant to math or science constructed? How is it possibly generated in these networked communities? Provide examples to illustrate your points.

Throughout this course, we have been discussing how students construct knowledge and understanding. In short, I think we can all agree that the most practical way to teach students is to give them multiple opportunities to explore and collaborate while scaffolding their learning and providing different means for them to express and apply their understanding. Traditional methods of memorization are no longer viewed as best teaching practices. Instead, we want students to build knowledge through concepts so they can apply what they have learned in problem-solving contexts. In Erlwanger’s (1973) article he reminds us of the danger of teaching mathematics without ensuring that students have a proper conceptual understanding and that memorizing procedures through rote learning can be ineffective. I can definitely relate to this article as I have seen many examples of when students have memorized how to complete a set of questions but cannot apply what they know in a real life application or when they are given an open-ended problem. Many of my students go to Kumon so they can complete a long series of questions quickly but many have no idea what they are doing. They have simply memorized an algorithm. For instance, I have a student who can complete 20 2-digit by 2-digit multiplication questions in under 3 minutes. But when asked to complete 22 x 4 in his head he cannot. Other students in my class who have no idea what the traditional algorithm for multiplication is, let alone how to use it, could answer this question because they understand what multiplication is. They know that it is adding equal groups and can partition the numbers using mental math and will add 20 groups of 2 to 2 groups of 2.

It was very interesting to read about how to use informal spaces to construct knowledge and build conceptual understanding. The topic of field trips is something we are continually talking about at school because we want them to enhance learning and help construct conceptual knowledge, but more often then not, they become stand-alone lessons where the students are learning in isolation information that isn’t necessarily connected to the goals we want to achieve. Yoon et. Al (2012) examines the potential that these informal spaces can have to increase students conceptual understandings of science. They found that using augmented realities to replace tradition text guides would keep visitors more engaged and therefore “improve access to information and increase exhibit functionality.” (Yoon et al. 2011). After browsing the Exploratorium Museum’s website that is located in San Francisco, you can see that they have created many galleries and exhibits that would let visits explore concepts using elements of embodies learning. Instead of just viewing and listening to information, visitors have the opportunity to test and create and explore different ideas. This is very similar to the pedagogical model that we want to see in schools.

I think that using informal spaces and going on field trips can be influential in building conceptual understandings. When planned and executed in a meaningful way, it can give students opportunities to apply what they have learned in class in the real world as well as see ideas and gain new perspectives. Just like we have seen with materials like Jaspers, teachers don’t always need to be the ones who are delivering content. Using spaces like museums is another tool that we can utilize to help students inquire into the world around them and construct their own knowledge.

References:

Erlwanger, S. H. (1973). Benny’s conception of rules and answers in IPI mathematics. Journal of Children’s Mathematical Behavior, 1(2), 7-26.

Yoon, S. A., Elinich, K., Wang, J., Steinmeier, C., & Tucker, S. (2012). Using augmented reality and knowledge-building scaffolds to improve learning in a science museum. International Journal of Computer-Supported Collaborative Learning, 7(4), 519-541.

Embodied Learning and LfU

E-folio question/task – ‘Design an activity to challenge a specific misconception in math or science using one of the frameworks in Module B and one of the mobile technologies discussed. Explain your pedagogical design decisions drawing upon embodied learning.’

As we have been discussing this week embodied learning is “how our physical bodies serve to externalize the activities of our physical brains in order to connect cognitive activity to the environment” (Winn, 2003). By utilizing the whole body, helps all students regardless of learning style stay engaged, retain information and make physical connections to content (Lingred & Johnson-Glenberg 2013). Through reflecting we have seen that many elementary and primary teachers use embodied learning and have it embedded in their practice. Students at this age are consistently being given opportunities to explore the physical world and move around. We have discussed that perhaps emersing these young children in mixed/augmented and virtual realities isn’t developmentally appropriate yet, as they should be working more with their physical environment. Using mixed-realities is something that students can be exposed to, but more as a stand-alone learning engagement. Therefore, below I created a task where students work through a common misconception in a TELE using the that uses both embodied learning and technology.

Students often believe that prime numbers are odd numbers. For instance, 80% of my students believed that the numbers 21 and 27 were prime numbers before any instruction. Using the Learning for Use model, I created a series of learning engagements for students to complete to understand the concept of what a prime number is. This is meant to go over the course of four to five 40 minute periods.

The first task involves students using their bodies to find factors to different numbers. Students should already have a strong understanding of what a factor is and how to find different ones for numbers up to 20. By getting them to move and find different pairings will help motivate them as well as keep them engaged. After tuning them in with this activity, they will watch a video to introduce the concept of prime numbers and then work through tasks on Khan Academy to reinforce what prime and composite mean. After the final task, the teacher can decide whether they need more practice before moving on.

Prime & Composite

Prime Numbers

Exit Ticket

References:

Lindgren, R., & Johnson-Glenberg, M. (2013). Emboldened by embodiment: Six precepts for research on embodied learning and mixed reality. Educational Researcher, 42(8), 445-452.http://www.move2learn.education.ed.ac.uk/wp-content/uploads/2015/04/Lindgren-2013-Embodied-Learning-and-Mixed-Reality.pdf

Winn, W. (2003). Learning in artificial environments: Embodiment, embeddedness, and dynamic adaptation. Technology, Instruction, Cognition and Learning, 1(1), 87-11

Embodied Learning and Mixed-Realities

It was fascinating to read and learn about embodied learning this week because as a primary teacher I see and use this type of learning regularly across the school, especially in the early years. Think of the song ‘I’m a Little Teapot’ or ‘The Itsy-Bitsy Spider’ for instance. While learning those songs everyone learns the lyrics while learning the gestures that go with it, and I would put money on the fact that most adults would be able to recite the words along with the movements years after being taught. According to Winn (2003), cognition does not just involve the brain but the whole body. Embodied learning then is “how our physical bodies serve to externalize the activities of our physical brains in order to connect cognitive activity to the environment” (Winn, 2003). Lingred & Johnson-Glenberg (2013) explain that in recent years there has been a lot of attention given to improve and introduce new instructional methods that focus on ways to include using the body to make meaningful connections with content in math and science. These new innovations have lead to the emergence of “new technologies that accept natural physical movement” such as gestures touch and body positioning. Combining the real world with the physical world is what Lingred & Johnson-Glenberg (2013) describe as mixed-realities (MR). In many schools today we have a wide-range of tools at our disposal to emerge students in mixed-realities. “These technologies typically involve [using] real-world objects, such as our bodies…[alongside] some type of digital display.” (Lingred & Johnson-Glenberg, 2013). While participating in a MR, students are in a controlled context where they have the chance to interact physically with the content to further understand concepts and explore cause and effect directly.

Reflecting on embodied learning and Module B, I think that planning with embodied learning in mind and mixed-realities could fit into any of the TELEs we investigated. Using this type of approach aligns well with the principles we discussed in the last module. It promotes learning through collaboration, it’s motivating, reaches all learning styles, as well as gives the teacher an opportunity to rethink and administer unique and well-planned assessments.

In Math class, I occasionally use an embodied learning approach and mixed-realities. For instance, a few weeks ago we made human graphs in different ways to teach students about scale, and we incorporated the robot Sphero when teaching students about angles. It definitely has a place in the classroom and I can appreciate how using the whole body and getting kids involved can help them make more connections, as well as be more involved and invested in their learning.

Questions I have are:

When should we introduce mixed/virtual/augmented realities etc? Should young students be exploring and making connections with their natural environments before exploring things outside of their physical reach?

Is embodied learning something that teachers do naturally? Does it need to be explicitly planned? Or is it something that is woven into planning organically through best practices?

References:

Winn, W. (2003). Learning in artificial environments: Embodiment, embeddedness, and dynamic adaptation. Technology, Instruction, Cognition and Learning, 1(1), 87-114.

TELEs Synthesis

The four environments we explored in this module are excellent examples of how our pedagogical approach to teaching and learning in Mathematics and Science should be hands-on, collaborative and inquiry-based. In all environments, students are given different opportunities to engage in learning that is both meaningful and engaging. All these TELE’s encompass aspects of the constructivist learning approach and aim to integrate technology that redefines the learning experience rather than just being a minor substitution for other tools.

While reflecting on these four environments and the tools we investigated, I looked at how I could apply them to my school’s pedagogical framework to teaching. We currently use the IB’s inquiry cycle for planning and specifically the Mathematics inquiry cycle for our Numeracy units. Within this framework we have students work through a cycle (not necessarily linear, sometimes going back and forth) of ‘Constructing Meaning,’ ‘Transferring Meaning,’ and ‘Applying with Understanding.’ Any of the models and tools we investigated would fit in the ‘transferring meaning’ phase after students have taught new concepts. The tools investigated all give students a chance to work with the ideas and new knowledge they have been shown and practice them to build conceptual understanding. Once established they are given a summative assessment where they apply these understandings in a meaningful way

Below is a table to help synthesize the four environments and explain the pros and potential cons to each one.

Module B Synthesis

Patterns in G3

Similar to the LfU model, I appreciate how the T-GEM approach to teaching is through inquiry. I chose to create a lesson around a ‘Patterns and Relations’ outcome. In my experience, in the early years, students have a strong understanding of how to identify, extend and replicate simple patterns such as AB patterns, ABC etc. Next steps in their conceptual understanding is to identify pattern rules for increasing and decreasing patterns and then apply them to an algebraic equation. This is a concept that many students in Grades 4 and 5 struggle with. I teach Grade 3, and our goal is to prepare students for this by having them predict future terms by analyzing and thinking about patterns critically. In the plan outlined below, I integrated technology as both an optional tool for students to use in their investigation, and as a tool for students to explain their thinking. As Khan (2007) explains, this is a cycle that students should go through many times, therefore students would be asked to repeat the ‘evaluate’ and ‘modify’ activity in different ways to help achieve mastery. Example question for ‘Evaluate‘

Replicate the models below by making a drawing, using tiles, or using your interactive whiteboard. Identify the pattern rule and then make Case 4 and 5.

Fill in the table below.

Analyse the pattern rule. Can you predict what Case 7 would look like? How many tiles would it use? Case 10? Make your predictions and then check your answer by building each one.

References:

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905

Time with LfU

I really appreciate the features of the LfU framework. LfU is a model that teachers at any level can adapt and work onto an inquiry enriched context. One aspect I think all teachers would enjoy is that it is broken up into three sections. You often see frameworks and models that are multilayered, but LfU managed to embed and include essential features of multiple teaching and learning theories into three parts which makes it a manageable user-friendly model. By adopting this type of framework, teachers can ensure that students aren’t merely memorizing facts but instead building knowledge through activities that foster conceptual understandings. Learners can then apply what they are learning in their real life (Edelson, 2000). Furthermore, the framework also lends itself nicely to technology integration. By adding this layer allows learners to work on their spatial literacy. The advantage to this is that students will be able to “manage, visualize, and interpret information” which Perkins et al. (2010) describe as something that will be necessary for employees in our future workforce.

One concept that my Grade three students often struggle with is the concept of time and specifically elapsed time. Time is one of shape and space outcomes that I see as being one that is most applicable to real life, but often not a lot of time (ironically) is spent on it in school. This is why I chose this outcome. Below is a table that I created and included an activity for each section of the LfU model. One crucial aspect that is not on this table is ‘reflection.’ While I haven’t covered it here, I think it is one that is of utmost importance in any subject. One activity I might have my students do is repeat the motivation activity and have them reflect on how their knowledge improved and what strategies and tools they used the first time and what ones they used the second time.

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching,38(3), 355-385

Perkins, N., Hazelton, E., Erickson, J., & Allan, W. (2010). Place-based education and geographic information systems: Enhancing the spatial awareness of middle school students in Maine. Journal of Geography, 109(5), 213-218.

SKI/WISE Reflection

WISE was created in the 90’s as a way to bring a new way of learning to the classroom by taking advantage of new technological advances and the internet (Slotta & Linn, 2009). Using the SKI framework, WISE projects are developed to be inquiry-based and have a number of different elements that are consistent with the constructivist learning theory. Projects are designed to be collaborative, use various features that appeal to a wide range of learners, use open-ended questioning techniques, as well as incorporate useful tools to help make students thinking visible.

Like the Jaspers materials, WISE encourages students to work together, and its contents are accessible to all learners. Some questions are open-ended, and there are attempts to make the material applicable to real life. WISE projects are more extensive, however, and students are gaining and applying knowledge as they move through them. The Jaspers videos are used for students to apply and practice concepts previously learned (Linn et al., 2003).

I would use WISE projects alongside classrooms instruction and projects. I found the materials to be very text heavy and a lot of material for students to go through on their own. In my context with EAL learners, I would want to make sure that students had a good understanding of what the vocabulary was before going through the activities. It would also be ideal for students to be involved in a hands-on experiment or project alongside the material as a way to reinforce the concepts learned. Including more videos within the online activities would also be helpful as a way to reduce the amount of reading. Finally, I would add having tuning in activities and pre-assessments that activate prior-knowledge, draw out misconceptions and also encourage students to ask relevant questions.

I created this table after reading posts from others and considering other perspectives.

Linn, M. C., Clark, D., & Slotta, J. D. (2003). WISE design for knowledge integration. Science education, 87(4), 517-538.

Slotta, J. D., & Linn, M. C. (2009). WISE science: Web-based inquiry in the classroom. Teachers College Press.