Category Archives: B. PCK

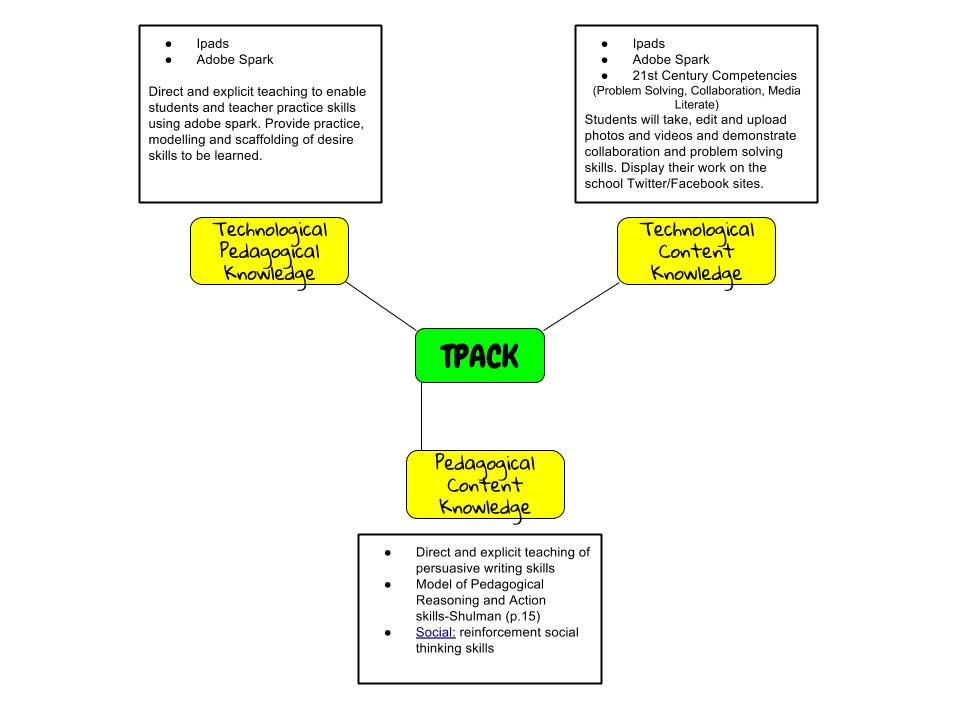
In second grade, fluency in single and double-digit addition become early stages of multiplication. In my current class, we are now looking at multiplication, though these second graders are a strong group and have made big jumps, thanks to their foundation teacher last year, who is a whiz at explaining and reinforcing number sense.

We begin with looking at the students’ strengths with number bonds (to 10, then 20), and we use manipulatives produced by the Numicon program to help our maths understanding.

Having a strong understanding of number bonds (7 + 3, 6 + 4, 8 + 2, etc.) helps them ‘make a ten’ and recognize and name other mental math strategies. It has been incredible for me, to use and explain the concept of number bonds with Numicon shapes, as simple and as lego-like as they are. The program was originally designed as a remedial resource, but the school I work at has adopted it as a foundation for all students, and I strongly feel that it has improved students’ number sense and confidence with manipulating numbers by a large margin. Here’s a short video on YouTube if you’re unfamiliar with what these manipulatives look like. When dealing with larger numbers, we introduce Cuisinaire rods as manipulatives, and begin to move towards developing and relying on Mental math strategies like these:

We explicitly do not look at the traditional algorithm in maths, until students demonstrate strong understanding of partitioning numbers, and feeling comfortable manipulating large numbers the same way they do with their smaller number bonds. Then, we teach the ‘lining it up’ method, where students can deal with smaller sums as a shortcut. This only comes after they can explain the place value of all of the numbers in the equation, and can explain step by step what they are actually adding. With a strong emphasis on “you solve it however it is easiest for you to see the numbers”, the only thing we ask students to do is to show their thinking. Whether that’s with a number line, compensation, partitioning, or otherwise. Funnily enough, even after using the traditional algorithm, many students in my class in particular still opt to add numbers in a lengthy way, because they feel it’s more visual and understandable. For example:

359 + 492 = 300 + 50 + 9 + 400 + 90 + 2, and

300 + 400 = 700, 50 + 90 = 140, 9 + 2 = 11, and 700 + 140 + 11 = 851

or more recently with respect to multiplication:

202 x 4 = 202 + 202 + 202 + 202

200 + 200 + 200 + 200 = 800, and 2 + 2 + 2 + 2 = 8, and 800 + 8 = 808

Word problems are a part of the teaching all the way through, and identifying the language that commands a certain function (“find the difference, how many altogether”) is recorded and referred back to regularly.

Admittedly, I was afraid of teaching math for a long time because it was something I lacked confidence and fluency in. Thinking about PCK for math scared me as a fresh graduate of teacher’s college, because my foundations for content knowledge were shaky at best. And as Mishra and Koelher point out, “…having knowledge of subject matter and general pedagogical strategies, though necessary, was not sufficient for capturing the knowledge of good teachers” (Mishra & Koelher 2006), which made it even more intimidating. Tools like Numicon and and the GloSS assessment for mental maths have been incredible for laying out processes in problem solving and mental math strategies. I strongly believe that these resources, in addition to seeking out help and advice from those much stronger than me, has allowed me to get a little closer to what Mishra and Koelher describe as “find[ing] different ways to represent it and make it accessible to learners” (Mishra & Koelher 2006). Though I clearly still have a long way to go, I’m confident in explaining different ways of thinking about numbers (at the elementary level, mind you!), and this allows me the perspective of being critical of technology integrated in my pedagogical practices, unless it is truly beneficial for the learning and allows students to further explain their thinking or explore concepts that they could not see otherwise.

Reference:

Mishra, P., & Koehler, M. (2006). Technological pedagogical content knowledge: A framework for teacher knowledge. The Teachers College Record, 108(6), 1017-1054.

Image source

I found Shulman’s (1987) view of teaching profounding enlightening; he stated that “teaching ends with new comprehension by both the teacher and the student” (p. 7). In other words, the priority of education is not just for student learning, but equally significant is the teacher’s comprehension. I could not agree more; teaching should involve presenting ideas that both student and teacher can make constructive meaning. Shulman (1987) argues that teachers do not need be expects of the knowledge, rather “the key is distinguishing the knowledge base of teaching lies at the intersection of content and pedagogy[PCK] p.15). This reminds me of a particular student teacher I had who had a degree in engineering and worked in the corporate world for several years before pursuing an elementary teaching career. They understood the text and it fueled their lessons, unfortunately they lacked the aspects of pedagogical reasoning. For instance, the student teacher had difficulties adapting and tailoring to the diverse needs of the students, did not allow for discovery or inquiry learning, and was not open to new understandings of the content. Clearly, this student teacher needs to do critically analyze their performance and the experience of the students.

This is one the first time I have seen TPACK during my MET journey, as it is an educational framework for understanding technology integration for both teacher and students. The central ideas around TPACK are; Technological knowledge, Pedagogical Knowledge and Content Knowledge, as they work together to support good teaching practices. Mishra, P., & Koehler, M. (2006) “[argues] that the TPCK framework [allows teachers] to guide curriculum design and [helps] create conceptually and epistemology coherent learning environments. [They] call [this] approach learning technology by design” (p.1034). I have attempted to create a flowchart of my TPACK teacher experience, where students needed to use their persuasive writing techniques to create a video to support their agreement whether fidget spinners should or should not be allowed in the classroom.

Mishra, P., & Koehler, M. (2006). Technological pedagogical content knowledge: A framework for teacher knowledge. The Teachers College Record, 108(6), 1017-1054.

Shulman, L.S. (1987). Knowledge and teaching. The foundations of a new reform. Harvard Educational Review, 57(1)1-23. 

TPACK is an online framework that includes 3 distinct and important areas: Pedagogical Knowledge, Content Knowledge and Technological Knowledge.

TPACK grew from Pedagogical Content Knowledge (PCK). PCK refers to the strategies we use in teaching course content. As Schulman (1987) states PCK is a “special amalgam of content and pedagogy that is uniquely the province of teachers, their own special form of professional understanding.” PCK is an intersection of content knowledge and pedagogical knowledge. We generally acquire content knowledge as teachers through professional development, our teacher training, and whatever personal education we have experienced. Our pedagogical knowledge is the strategies we have learned, such as project-based learning, think-pair-share, direct instruction etc.

When we discuss Technological Knowledge it may refer to an understanding of things like google suite, smartboards, Khan Academy, Scratch, Kahoot!, Prezi, Socrative etc. As teachers, we must decide what technology is best to provide the most engaging and productive learning for our students. Our ability to do this refers to our Technological Content Knowledge (TCK).

If we combine the three areas we have TPACK, which is “the basis of good teaching with technology and requires an understanding of the representation of concepts using technologies; pedagogical techniques that use technologies in constructive ways to teach content; knowledge of what makes concepts difficult or easy to learn and how technology can help redress some of the problems that students face; knowledge of students’ prior knowledge and theories of epistemology; and knowledge of how technologies can be used to build on existing knowledge and to develop new epistemologies or strengthen old ones.” (Mishra & Koehler, 2006). TPACK then is building on the pre-constructed knowledge students already have, using technology from an informed and researched pedagogical approach.

An example of TPACK I could use was my experience teaching coding last year. I taught one grade 10 IT class for the year and I began a coding unit in the first term, starting with the hour of code. This began on code.org and then moved on to scratch where I taught a modified version of the curriculum for basic coding that is available. After progressing through this, students were asked to create a story related to a topic of study in one of their other courses. The story had to be told via coding in scratch. It was a very interesting exercise that the students benefitted from greatly. Math, science and humanities all intersected as the project carried out. I believe in this instance technology, pedagogy, and content mixed in a way that Mishra, P., & Koehler, M. (2006) described.

Mishra, P., & Koehler, M. (2006). Technological pedagogical content knowledge: A framework for teacher knowledge. The Teachers College Record, 108(6), 1017-1054.

Shulman, L.S. (1987). Knowledge and teaching. The foundations of a new reform. Harvard Educational Review, 57(1)1-23.

As a teacher who was trained first in a faculty of education and then went back to school for degrees in French and English I found this week’s reading interesting. In Alberta and Saskatchewan at least, elementary generalists are trained to know a little bit about everything and an undergrad degree in education contains one class in each of the content areas plus many courses in pedagogy. Moving into the classroom, however, teachers realize they need to not only know how to teach (PK) but also what to teach (CK). I have been in the classroom long enough to see a pendulum swing from phonics instruction to whole language to phonics instruction and I see that many teachers are missing an understanding of phonics and how language is structured and are not able to include that in their teaching. As such, professional development opportunities become essential. In my division in the past couple of years there has been a fund developed to send school teachers back to university for maths courses (once I’m done my masters I’m definitely planning on taking advantage of this). As Shulman argues, there is definitely a balance for classroom teachers between all of the elements of TPCK and I think an effective teacher must be a master of not only the content (many is the university prof who knows their content inside out and backwards but is unable to teach it) but also the pedagogy and how technology augments the learning. As an elementary teacher, I see the math and science we do as being foundational and this is where a teacher with a tenuous hold on math concepts can still teach the math but may inadvertently introduce misconceptions to students that have to be corrected later in their learning journey. Something as innocuous as using “makes” instead of “equals” (ie. 3+7 makes 10 instead of 3+7 equals 10) makes it difficult for a learner to make sense of algebra later when the equal sign does not always appear at the end of a question. For this reason, I think it’s a good thing that many universities are moving to an education degree that begins with a bachelors in a content area followed by a 1-2 year education after degree.

Shulman, L.S. (1986). Those who understand: Knowledge growth in teaching. Educational Researcher, 15(2), 4 -14

“New comprehension does not automatically occur, even after evaluation and reflection. Specific strategies for documentation, analysis, and discussion are needed.” (Shulman, 1987, p.19)

This quote is crucial to evaluating mathematical word problem solving skills. Students require direct instructions to support the development to skilfully extract information from word problems, identify missing attributes, select most approach analytical method and compute and make sounded conclusions. GRASP is an explicit metacognitive strategy that trains learners to effortlessly process word problems.

Using the GRASP Method to teach word problem solving skills

What is GRASP?

GRASP is a strategy to solve mathematical word problems. Below is the one of the many definitions of GRASP.

G – Give Information (i.e. operation signal words/ phrases)

Students are to extract information from a given word problem.

R – Required Information

Students are to identify parts that need to be solved.

A – Analyze (i.e. mathematical strategies)

Students are to select appropriate strategy (or strategies) to develop an answer.

S – Solve the question (i.e. computation)

Students are to use the select strategy (or strategies) to solve the question.

P – Paraphrase the answer

Students are to communicate their answer in a way that solves the problem.

Pedagogical Knowledge

Pedagogically, the GRASP method is a suitable framework to teach students how to solve word problems because this strategies shows thinking processes. The method helps make thinking visible by asking students to isolate and document components of the word problems. Linn (2000) believes that making thinking visible makes the metacogntive processes inspectable. In the case of GRASP, students can reflect upon their work and inspect the areas that led to an erroneous answer. For example, if it were a computation error, the analysis part would contain mistakes.

Teachers can clearly insepect how a student comprehends authentic word problems. Since students have to mindfully select appropriate analysis strategies based on given information, it mobilizes an integrated understanding of problem solving strategies. Moreover, authentic word problems make comprehension and problem solving accessible and relatable. Students are also more motivated to solve problems that were created by their peers.

Additionally, this strategy requires teaching practices such as scaffolding and modeling. Without this pedagogical strategy, students fail to independently apply the GRASP method. It is best to model as a whole class demonstration. Here, one can employ the cognitive apprenticeship. This pedagogical design helps foster problem-solving success by providing independent practice time. Moreover, this pedagogical awareness and experience helps promoting lifelong mathematical learning and success by installing a plausible approach to solve word problems.

Another pedgagoical consideration is about differentiation. The GRASP method enables all learners to find success in solving word problems. Some students find that advance operations (i.e. multiplication and division) are easy. In contrast, some students require direct operational signals to solve problems. This problem-solving approach allows educators to differentiate for slow or excelling learners. For example, teachers can modify the wording, include computation choices and provide sentence starters to fit the needs of their learners. Nonetheless, this depends on how well educators know about their students’ needs and strategies to support the needs.

Content knowledge

Shulman (1987) suggests that teachers serve “as the primary source of students understanding of content knowledge” (p.9). In the case of GRASP, teacher’s knowledge of signal words (i.e. math words and phrases that signals operations) and age-appropriate problems solving strategies influence how successful GRASP is. Explicit teaching of math vocabulary and computation strategies is key. Educators should be able to help students develop a strong repertoire of this knowledge. In this instant, educators need to develop content rich anchor charts to supplement students’ understanding of computation strategies and words that signal specific operations. Students also benefit when they are front-loaded with this information. These ideas are consistent with Shulman’s (1987) emphasis on the importance of teacher’s content knowledge. Without building a strong foundation of words and strategies, students fail to employ the GRASP method on more challenging word problems. Learners may also lack skills to develop their own problems.

GRASP & Technology

Although GRASP can be used without technology, simple word-processing knowledge would be beneficial. Educators can make set word problems more organized and attractive by printing off typed word problems and adding pictures. Additionally, to demonstrate that some problems are differentiated, teachers can place a picture on the opposite side.

Another key advantage of using the GRASP method is that educators can analysis the isolated parts of the word-problem solving process. Educators can digitally document students’ area of strength and or weakness. More specifically, teachers require higher digital competency to code an Excel sheet to automatically map student data and the evolving performance trends. For example, after values are imputed, the Excel can use color to highlight consistent errors and populate other visuals to show trends for student performance.

For learners, the computation part the GRASP can be digitized. For example, as students use GRASP, they can replace writing and drawing via technical means. More specifically, students can use Excel to make a graph or use Google drawing to visualize and manipulate variables to improve computation efficiency and accuracy. This is consistent with Jonassen’s (2000) claim. He believes that “that the most productive roles for media are as computation and memory tools for off-loading unproductive cognitive task that may interfere with knowledge construction by the learner.” (Jonassen 2000, p.33) Furthermore, students can also discuss their GRASP methods on student forums to extend their and extend their thinking.

Food for thought

In Mishra & Koehler (2006), although the overlapping circles appeal to be a useful method to talk about integrating these different types of knowledge, it may not accurately represent the extent of each type of knowledge. Rather, a power map may provide more information. In a sense, each example has its own power map. Here is what the GRASP model may look like:

Visibly, the success of GRASP relies heavily on pedagogical and content knowledge.

A follow up question is how educators evaluate whether this is a ‘good’ mix of Msihra & Koehler’s idea of TPACK? Are these variations dependent of content?

References

Mishra, P., & Koehler, M. J. (2006). Technological pedagogical content knowledge: A framework for teacher knowledge. Teachers college record, 108(6), 1017.

Jonassen, D. H. (2000). Computers as mindtools for schools, 2nd Ed. Upper Saddle River, NJ: Merrill/ Prentice Hall. Retrieved from Google Scholar: http://scholar.google.com/scholar?q=Jonassen+mindtools&ie=UTF-8&oe=UTF-8&hl=en&btnG=Search

Linn, M.C. (2000). Designing the knowledge integration environment. International Journal of Science Education, 22(8), 781-796.

Shulman, L.S. (1987). Knowledge and teaching. The foundations of a new reform. Harvard Educational Review, 57(1)1-23.

PCK and TPACK – best practices

When reading these articles, it really struck me that these authors really get it.  For a classroom or a lesson to be effective, the teacher has to not only know and be able to interact with the content, but also with the methodology that is most effective to the context of the topic.  For many years I taught high school math, and while I could explain well, I was never able to make it interesting or relevant to the students.  I wasn’t immersed in the context. 

For science, and in particular, biology that has not been a problem for me.  I have been interested in the world and creatures around me for as long as I can remember, and still find it fascinating.  To use anecdotes, analogies, and methodologies to make it interesting has just been an extension of what I already want to do.  While I appreciate the value of the PCK and TPACK model, I wonder if there is a lack of focus on the individual teacher as well.  Putting theory and practice together while combining TPC knowledge is a great skill to have and will undoubtedly improve the engagement and learning in the class.  I would argue, however, that a teacher with a love for their students and their course while lacking some framework may be just as effective if not more so, than a trained teacher without that love.  My favourite topics always seem to go over the best regardless of the methodologies used for them or other topics.

One activity I have done regularly is for the homeostasis (human body systems) unit in biology 11.  For this, I have developed a number of different medical scenarios or case studies.  Each group is assigned a “patient” with a medical situation that they have to diagnose and develop a treatment plan for.  They needed to research symptoms, request diagnostic tests and technologies, ask questions and interact with the patient and doctor via e-mail (it’s me) and create a tactful bedside manner report on what is ailing them and what they are going to do about it.  In addition to learning the body systems, they also learn many other skills – collaboration, responsibility, deduction, and technological skills like research, organization, and layout.  For the most part they are very engaged and thorough.  I believe this activity meets the criteria for the TPCK overlap, as the technology supports the learning of the content in a way that engages the students creatively and with collaboration.

I have also begun using WIKIs and digital artifacts as ways to have students construct their knowledge.  Once most of the topics have been addressed at least once, I think I would like to go the next step of designing their knowledge – give my students choices to design games or activities that demonstrate understandings of the concepts.  In ETEC510, we read a number of articles (Kafai, 2006; Kafai & Resnick, 1996; Brennan & Resnick, 2013; Kalantzis & Cope, 2010; Mouza & Lavigne, 2013) that emphasize the power of design for learning – first for the teacher, but also for our students.  Mishra and Koeler (2006) also used case studies from a MET program designing course that further elaborate this point.  Mouza and Lavigne suggest a needed shift from children as consumers to children as designers,  writing “as young people design interactive media, they go through an iterative process of imagining, creating, playing, sharing, and reflecting” (p. 11).  When learners are most involved is when they contribute the most – to their own learning, to the class, and to society at large.

When I first read those articles, I wrote “Playing is fine for younger kids learning motor and social skills, but high school must be more serious- there’s content, information, and skills to develop.  Can students really be trusted to learn for themselves?  What about the basic knowledge needed for future courses and the workplace?  Life skills?  Budgeting, tax returns, giving out change?  Safety?  Our bodies?  The 3 R’s?  What if it’s not what I expected, or wanted?  I must confess I REALLY don’t know.  I’m not even sure if I have any ideas.  I think I need to ask my kids!  Some small part of me is also saying “ask your students – talk to them – give them some say in their education”.  I think I will.  I have no idea where this will lead, it’s kind of scary, but maybe, just maybe, worth it?”

After reflection, I think I’m still in a similar place.  I still don’t have the answers, but my knowledge and willingness to learn and try things continues to grow, and I still believe it’s worth it!

Questions for Discussion or Reflection:

1. Is being outside your comfort zone a good place to be?  If we are not the intuitive knowledgeable experts that TPACK is seeking, does this mean we should stick to more traditional methodologies?
2. Interestingly, Apple’s philosophy appears quite similar as shown by their stated goals for Apple Classrooms of Tomorrow – Today.  How does this framework align with TPACK?  Is it a good thing for commercial interests to take this close of an interest in educational pedagogy?  What biases or influences would they bring on teachers or into classrooms?

Figure 1: Three major influences on 21st century learning.  Reprinted from Apple Classrooms of Tomorrow—Today: Learning in the 21st Century by ACOT2, 2008.  Retrieved from http://ali.apple.com/acot2/global/files/ACOT2_Background.pdf.

Figure 2: Six Design Principles.

Reprinted from Apple Classrooms of Tomorrow—Today: Learning in the 21st Century by ACOT2, 2008.  Retrieved from http://ali.apple.com/acot2/global/files/ACOT2_Background.pdf.

• Brennan, K. & Resnick, M. (2013). Chapter 17: Imagining, Creating, Playing, Sharing, Reflecting: how online community supports young people as designers of interactive media.In C. Mouza and N. Lavigne (eds.), Emerging Technologies for the Classroom, Explorations in the Learning Sciences, Instructional Systems and Performance Technologies. New York: Springer Science &Business. DOI 10.1007/978-1-4614-4696-5_17

• Kafai, Y. B., & Resnick, M. (Eds.). (1996). Constructionism in practice: Designing, thinking, and learning in a digital world. Hillsdale, NJ: Erlbaum.
• Kafai, Y. (2006).Playing and making games for learning: Instructionist and constructionist perspectives. Games and Culture. 1(1). 36-40.
• Kalantzis, M. & Cope, B. 2010.The teacher as designer: Pedagogy in the new media age. E-learning and Digital media 7(3). 200-222.
• Mouza, C. and Lavigne, N. (eds). 2013.Chapter 1: Emerging Technologies for the Classroom. Explorations in the Learning Sciences, Instructional Systems, and Performance Technologies. New York: Springer Science + Business Media.

• Shulman, L.S. (1986). Those who understand: Knowledge growth in teaching. Educational Researcher, 15(2), 4 -14.
• Shulman, L.S. (1987). Knowledge and teaching. The foundations of a new reform. Harvard Educational Review, 57(1)1-23.
• Mishra, P., & Koehler, M. (2006). Technological pedagogical content knowledge: A framework for teacher knowledge. The Teachers College Record, 108(6), 1017-1054.

As a Technology Integration Specialist, I spend much of my time helping teachers “to reason soundly about their teaching as well as perform skillfully” (Shulman, 1987, p. 13). The process of reasoning is collaborative as teachers must have a stronger concept of pedagogical content knowledge (PCK) and I usually have stronger concept of technological pedagogical knowledge (TPK). Thus, teachers have a better sense of “what makes the learning of specific topics easy or difficult” (Shulman, 1986, p. 9), and I have better “knowledge of the existence, components, and capabilities of various technologies as they are used in teaching and learning settings” (Mishra & Koehler, 2006, p. 1028).

A typical exchange with a teacher would start with a meeting where we discuss lesson plans and/or curriculum maps; we would go over the learning outcomes and discuss pedagogical practices that have been effective in developing student thinking and knowledge. For instance, one of my Math teachers discussed using the Harkness method and how it helped students work through more difficult problems collaboratively. From my previous interactions with the English Department, I suggested a new application called “Parlay Ideas” which facilitates Harkness style learning; with this application, students feel more comfortable sharing ideas and teachers can quickly track student learning with visual snapshots of each student’s participation/performance. After the implementation and trial period, several follow-up meetings were conducted to refine the use of the technology.

In a perfect TPACK world, every teacher would understand their content, what strategies are useful in helping students learn that content, and which technologies can provide effective enhancement to the learning process. One might argue that this framework sounds awfully teacher-centered; there is a huge push for teachers to fall into the background and allow the “human learner (to be) involved in learning and ultimately the construction of knowledge” (Jonassen et al., 1994, p. 31). Is TPACK the best model to promote student learning? How far should the pendulum swing between teacher-centered and student-centered learning?

Jonassen, D. H., Campbell, J. P., & Davidson, M. E. (1994). Learning with media: Restructuring the debate. Educational technology research and development, 42(2), 31-39.

Shulman, L.S. (1986). Those who understand: Knowledge growth in teaching. Educational Researcher, 15(2), 4-14.

Shulman, L.S. (1987). Knowledge and teaching. The foundations of a new reform. Harvard Educational Review, 57(1)1-23.

Mishra, P., & Koehler, M. (2006). Technological pedagogical content knowledge: A framework for teacher knowledge. The Teachers College Record, 108(6), 1017-1054.

I first encountered the TPCK framework in one of my earliest MET courses yet I still think about it every term, whether it comes up formally in the readings or not. At the risk of sounding like every classic educational reading, I credit the persistence of my reference to TPACK due to its simplicity as a framework, one that elegantly encompasses so many key ideas that lie at the heart of education.

The clearest description I encountered of the original PCK framework came from Shulman (1987), who describes it as the “blending of content and pedagogy into an understanding of how particular topics, problems, or issues are organized, represented, and adapted to the diverse interests and abilities of learners, and presented for instruction.” Although first written in 1987, the statement resonates strongly over three decades later and continues to inspire (well, to inspire me, at least). I always viewed PCK as a practical application of the classic expression “greater than sum of its parts”; while pedagogical knowledge and content knowledge are essential, the greatest lessons are created using both at once.

Not to leave the most relevant knowledge type (to ETEC533) out to dry, Mishra & Koehler (2006, p. 1029) extended Shulman’s framework to include technology, which details TPCK as:

• the basis of good teaching with technology;
• requires an understanding of the representation of concepts using technologies;
• pedagogical techniques that use technologies in constructive ways to teach content;
• knowledge of what makes concepts difficult or easy to learn and how technology can help redress some of the problems that students face;
• knowledge of students’ prior knowledge and theories of epistemology; and
• knowledge of how technologies can be used to build on existing knowledge and to develop new epistemologies or strengthen old ones.

Mishra & Koehler continue by giving ideas on how to develop lessons using the framework with technology included.

As for an example of how I teach a particular concept, I’ve chosen my most recent “version” of teaching Integration by Parts in math.

Notes

I start by either handing out notes physically or making sure my students have a copy of the notes through some platform like D2L. If students don’t use a physical copy I encourage them to use a program on their mobiles that allows them to take and save notes.

Mind Map – Assessing and Building on Prior Knowledge

I’ll write “Integration by Parts” in a circle on the board and ask a bunch of questions about what students think it means, what topics we’ve covered in Calculus up to this point and so on. I have them “remind me” what integration techniques they know so far. This is a mix of direct questions or passive observations. Depending on what the class comes up with I may assign a few short review questions or solve some quick problems on the board. I’ll usually return to this throughout the class and make connections (literally, with lines) between what they’re learning today to what they (should) already know. Ultimately the whole point on this part is to help them determine that they do not yet have a strategy to solve the types of integration problems they’re seeing today.

Formal Introduction to Topic

Once the scene is set I’ll work through some examples with them and introduce them to a new formula:

∫udv=uv-∫vdu

I often help students connect this formula to a prior formula (‘Product Rule’ in differentiation) through a short, non-rigorous “proof”. This tends to help them remember it, or at least buy in slightly, as it gives them some context of where it came from.

Piece-by-Piece

Without getting too detailed (in this post), I spent the next few minutes working through an example with students and explaining the philosophy behind how to approach solving problems like this, one step at a time. I began including a mnemonic, LIPET, for students who struggled badly with this, and this tends to help them succeed assessment-wise.

Checking for Misunderstanding

After this I have students break out into groups to work on the first set of practice problems, one designed to iron out misconceptions or misunderstandings of the concept before they have to do any real calculations. I circle the room while they discuss the problems with each other and compare solutions. A student (or a few students – time depending) is asked to write their solutions on the board – or cast it using ScreenBeam or AirPlay – and them we discuss them as a class.

(For those who know the topic of Integration by Parts, this activity asks students to look at a set of expressions to determine 1) which function should be labeled as u, which will in turn give du, and 2) which function to label as dv, which will in turn give v).

Practice Time

Once we’ve had a discussion why may range from from pairs, to groups, to the whole class, a set of practice problems is assigned to work on solidfying what they have been working on today. Students may work independently or in groups, while I check in on all students throughout. Eventually students are provided full solutions which may be projected on the board at the front of the class or accessed online through D2L or similar, and they check each other’s work. Issues are flagged and I may choose to review questions either on a per-student basis on for everyone on the board. It depends on how many students had the same issue, and whether or not a student could instead be used to help another student.

Using Technology to Redress Problems

To end the lesson I provide an “Integration by Parts Exit Card” which is essentially 2 quick multiple choice questions that contain question types students often struggle with. This is either a physical card that they circle an answer on, or more commonly I will have them respond using something like Google Forms. Once they finish the card and submit it they can leave, and I’ll aggregate and review the results prior to the next class. This allows me to ensure that I can focus on specific problems my class is having with the topic before we build on it.

And that’s about it! I don’t leverage tech too much for this lesson because I haven’t found too much tech that seems like it would actually benefit the students, but I feel that the tech I do use is beneficial.

Do you have any suggestions on how I could improve here? I’d love to get some feedback!

Thanks for reading,

Scott

References

Mishra, P., & Koehler, M. J. (2006). Technological pedagogical content knowledge: A framework for teacher knowledge. Teachers college record, 108(6), 1017-1054.
Shulman, L. (1987). Knowledge and teaching: Foundations of the new reform. Harvard educational review, 57(1), 1-23.