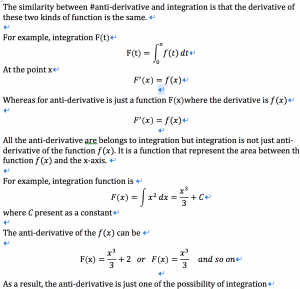
Definition of antiderivative:
Antiderivative is the inverse operation of derivative and is related to definite integrals through the first fundamental theorem of calculus. Given a function f(x), the antiderivative of that function can be F(x), which means F’(x)=f(x). It is the process to reverse the differentiation.
In math, a continuous function can have lots of antiderivatives. Therefore, we prefer to call a group of antiderivatives instead of a single antiderivative. G(x)=F(x)+c. We say G(x) and F(X) ARE both the antiderivatives of function f(x).
Definition of integrals:
Integration has a defined limit which It can be used to find areas and volumes with intervals. For the function f(x). The form , where c is a constant number. Here, the symbol means indefinite integral, function f(x) can be a integration with the constant number c. Usually, dx and the integral sign need to be used at the same time as a pair. It means the function f(x) is integrated with respect to x. The sign dx also tells people where the integrating function ends. If we change the variable x to t, the integrating function needs to end with dt.
We have learned fundamental theorem in class. In the first part of the theorem, the indefinite integral has close connection with antiderivative. If the function is continuous, we can guarantee the existence of the antiderivative of the function f(x). In the second part of the theorem, we can calculate the initial function f(x) by the given of any f’(x). If F(t) which is the anti-derivative of f(t) was given, then we can know the integral of f(x) on the interval [r,l]. However, if the integral is indefinite integral, we need to add a constant number C after the anti-derivative F(x) since any two antiderivatives differ by that constant.
But we actually know that antiderivative and integration are two different things in total, as we know, integral is a kind of area, if you don’t give me a number, but just an interval, I could give you an exact number, however, if I say that you give me the same function, but no exact numbers like “x=1”,”x=2”or something like this, it is impossible for me to give an exact number to you; On the other hand, the definition of these two things are different, antiderivative is the function before the derivative, but the integration is just an area that 1 or 2 functions can form; And actually, it is not rarely to think that there two concepts are two different things, like you cannot compare banana and plane, antiderivative is more like that, I have a program that coded in Dr.racket, I ran it, if without a real number, the program could not run forever, but actually if I input a number, then my codes will tell me that , “All Test Passed”, and on the other hand, integration is more like, how high the tower is, how much sand you’ll spend on it, the concepts are different from the beginning; And the other character that shows these two things are different is that, integral has an interval, but antiderivative don’t, because you’ll need the interval to calculate the value of the function; The second thing is that, we’ll never put an exact number into the function body that used for integral , but the definition of antiderivative shows that, we’re not sure that if there’s a “c” inside the initial function.