I was shocked that I had not encountered the LfU framework prior to this week. It seems to pull many of the concepts and theories that resonate with me into a nice package, and one that is meant to be easily applied to design activities. Brilliant!
Specifically, I enjoy how it leverages some of the most powerful aspects of constructivist, cognitivist and situated learning perspectives (Edelson, 2001, p. 357) and makes them concrete by providing specific criteria that each activity must meet — motivation, knowledge construction, and knowledge refinement, (I started calling it MCR). The paper even provides an amazing reference table that I promptly recreated, which I’ll append to my post.
If I was in the classroom this term I would want to jump on this framework immediately and try it out. Even so, I can think of a number of ways I might use it. I have been trying to pick apart Desmos from all angles this term, and so in searching for neat activities I stumbled upon Will it Hit The Hoop? (http://bit.ly/2clkfWj)
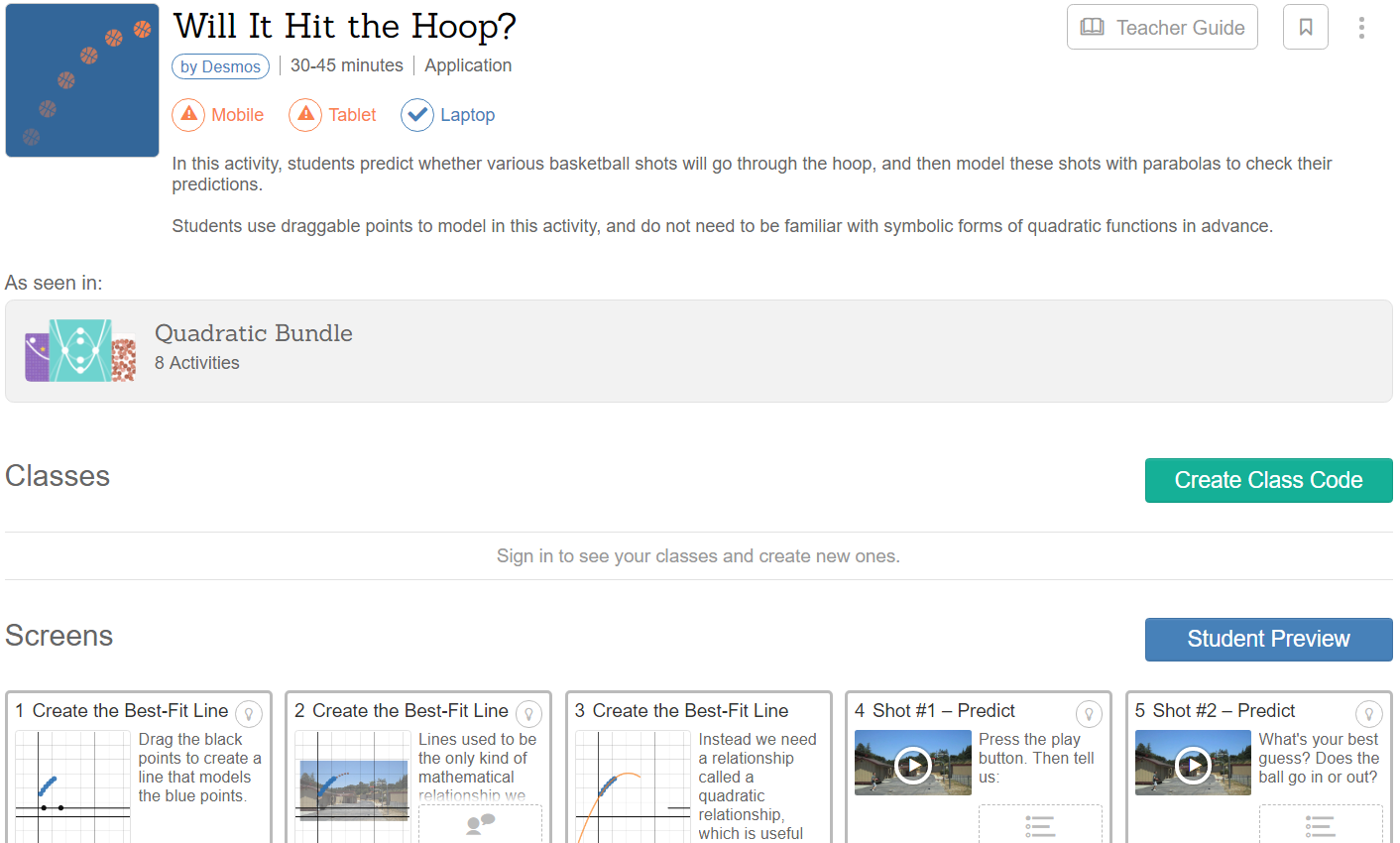
— For context I recommend you quickly try out the Student Preview, it’s pretty great —
Here are my thoughts about the activity, organized using the Table 1 from Edelson’s paper:
| Step |
Process |
Design Strategy |
| Motivate | Experience
curiosity |
The activity starts by having students attempt to fit a basketball shot with a line of best-bit. Noticing this in real-life context, with help from a video, could elicit curiosity and cause students to address the problematic gap or limitation in their understanding of how basketballs travel. |
| Experience
demand |
Students are encouraged to guess answers early in the activity, but later questions create a demand for the knowledge as they require students to have explored previous questions, and use what they have learned about quadratic functions and parabolic motion. The extension activities cannot be solved unless their knowledge is applied. | |
| Construct | Observe | The introductory activity paired with videos of real-life basketball shots provide direct experience with the novel phenomena they are exploring (considering the flight of a ball frame-by-frame), and in order to make the predictions expected of them early in the activity they must be observant. |
| Receive
communication |
Early in the activity, students will be providing a range of answers to the prediction questions followed by small-group and whole-class discussions. This communication on several scales allows students to learn from one another (ZPD comes to mind), as well as start to build new knowledge structures based on the communications taking place. | |
| Refine | Apply | In order to take their new knowledge structures from declarative to procedural form (Edelson, 2001, p. 359) it must be applied. This activity expects students to make use of their new knowledge structures, using the real-life videos and discussions as indices, to analyze basketball shots using mathematical equations. This direct manipulation of graphs, when overlayed on top of an image/video of a contextually-relevant basketball shot, reinforces what they have learned. Follow-up questions, analysis of actual student predictions with and without mathematical help, and extension questions, meshed with ongoing discussion and teacher guidance, all help students to reorganize their understanding into a useful form. |
| Reflect | Opportunities for reflection are woven into the activity at key points to force students to reflect on what they have observed or learned up to that point. This allows them to reorganize and reindex their knowledge prior to having to extend their new knowledge structure when information is encountered later in the lesson.
Note: The manner in which these reflection questions were incorporated reminded me of the structure of My World GIS, where investigations and discussions were spread out to allow incremental mastery of a concept. This is an excellent strategy that ensures that students are able to transfer information from short-term memory to long-term memory by rehearsal before they encounter new information. |
If I were delivering this lesson I would likely incorporate an over-arching reflection session at the end which forces students to process everything they had learned. I would then, depending on the skill level (and Grade level) of students, love to have them do their own experiment where they take their own videos of throwing a ball and confirm that even their own throws can be modeled using mathematics. This would encourage even further buy-in for the students than a video of someone else.
What does LfU afford students and teachers?
Well, it should be clear by its very name, learning-for-use. Well-designed activities would finally remove the need for students to ask “when will we ever use this?” because the activities are steeped in real-world contexts.They should, due to their constructivist/cognitivist nature, be designed to set students up for success because they are highly student-centred. Having to consider motivation, construction, and refinement of knowledge before even delivering the activity ensures the students will walk away having been afforded far more than a one-way lecture ever could have. For teachers, LfU provides clear direction both in the design and facilitation of each activity, and allows ample opportunity for teachers to guide and facilitate. It’s a win-win.
But what if students still ask, as they often do, something like “when will we ever use this in real life? I mean, it’s not like calculating trajectories and creating parabolas will help us on a basketball court”. Which is true, they probably won’t. But, the concepts they learn, the understanding and knowledge of the processes they will have internalized, could help them to extend what they learn and apply them to future projects. The teacher could discuss with them the power of these equations – how they are one way to truly predict the future. I bet they couldn’t do that before. The main point is that once they understand that all objects launched adhere to these rules (barring air friction) then the world is their oyster; they could use the concepts to create their own catapult even, or use the equations to program their own computer games. Actual, honest-to-goodness real-life skills. Neat!
References
Bodzin, A. M., Anastasio, D., & Kulo, V. (2014). Designing Google Earth activities for learning Earth and environmental science. In Teaching science and investigating environmental issues with geospatial technology (pp. 213-232). Springer Netherlands.
Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching,38(3), 355-385.
My World GIS videos, ETEC533 Module B.
Appendix – Table 1 from Edelson (2001)
| Step | Process | Design Strategy |
| Motivate | Experience
demand |
Activities create a demand for knowledge when they require that learners apply that knowledge to complete them successfully. |
| Experience
curiosity |
Activities can elicit curiosity by revealing a problematic gap or limitation in a learner’s understanding. | |
| Construct | Observe | Activities that provide learners with direct experience of novel phenomena can enable them to observe relationships that they encode in new knowledge structures. |
| Receive
communication |
Activities in which learners receive direct or indirect communication from others allow them to build new knowledge structures based on that communication. | |
| Refine | Apply | Activities that enable learners to apply their knowledge in meaningful ways help to reinforce and reorganize understanding so that it is useful. |
| Reflect | Activities that provide opportunities for learners to reflect upon their knowledge and experiences retrospectively provide the opportunity to reorganize and reindex their knowledge. |
Hi
I like the fact that you shared an excellent activity that would encourage sport minded students.
I wonder if for the same educational outcome there could be different LfUs? You gave an example for the sports-minded student…what about students that do not like or understand sports?
A good next step might be to explain to me why students will try to make basketball shots outside their range? When I was coaching junior teams, many of the players would try to make shots from the 3 point line — even when they could not make the basket from 4 feet away.
Christopher
Hey Christopher, and thanks for your reply!
I’m sure that the LfU framework would allow for meeting this outcomes through other means. The example I gave was essentially helping to understand parabolas as opposed to straight line, using motion as an example. If this were a physics class I would likely stick to the idea of an object travelling through the air. This just makes sense considering how key projectiles are to 2D kinematics. Of course it does not need to be confined to sports, nor should it be. I suppose the problem is that most every-day experiences involving simple parabolic/projectile motion relates most closely to sports. Bullets could be relatable, but not to most, and bullets experience serious drag which clutter the equations. Planes don’t count because they aren’t in free-fall. The classic “shooting a moneky out of a tree” problem isn’t isn’t great because 1) animal rights amirite (kidding) and 2) it’s totally not relatable to the average person.
The more I think of your question the more I question how easily learning-for-use could be applied to a physics problem involving parabolas. Perhaps not to easily, especially if we’re looking for scenarios that connect the learning to the real world for students, preferably in a literal hands-on way. I’ll have to continue pondering that one.
As for parabolas and quadratics, though, there are many options. Teachers could approach it from the perspective of shapes; match the parabola to a human smile, someone’s chin, mapping to the Gateway Arch in St. Louis, things like that. Perhaps the teacher could poll the class ahead of the topic being introduced to get a feel for what they’re interested in and use that to help guide the lesson design. That’s not a fully-formed idea but it’s a start.
The hardest part for me is to consider how to design the activities so that they’re actually USEFUL. Traditionally structured courses related to math and science tend to teach concepts but more rarely the hands-on application of it; labs excluded here. It’s not like trades, where you learn how to solder or wire a house by literally soldering or wiring a house. In that case there is no barrier between what’s being learned and the skills being applied; learning and application are happening simultaneously in a context that negates the need to question why it’s being done. In academics… well… more creative thought is required by the teacher to make those most practical of connections.
Finally, you mentioned Stop Motion and observing processes closely, in small increments. I can think of numerous topics that deserve closer focus, one of these being trigonometry and its relationship to the circle. I firmly believe that if more students truly understood the Unit Circle, and how trigonometric relationships (and complex numbers) are derived from investigating circles, students would perform better on these topics. To be specific I mean the types of relationships explained here:
https://youtu.be/ovLbCvq7FNA
These relationships could be extended all the way up to quite intense math such as Fourier topics: https://youtu.be/r18Gi8lSkfM
These videos are a little heavy but if they were picked apart by a thoughtful teacher with activities designed around them (make their own stop motion animations connecting the unit circle to triangles?) perhaps students would have more “Ah ha!” moments rather than having to recite SOH-CAH-TOA a hundred times to remember it!!
Maybe wishful thinking… 🙂
Thanks for your response!
NOTE: Sorry, part of this post replied to Christopher and the end replied to Alice! I got all mixed up. I’m sure you can pick through the confusion anyway 🙂
As for students shooting from the 3-point line when they can barely make a shot directly next to it… I think it’s just because it seems like a really cool thing to do.
If you make a 3-pointer , even if you are fairly terrible at basketball in general, it’s pretty impressive… especially for adolescents that may feel like they have something to prove 🙂
It’s probably the same reason that I, being someone who could barely make a 4-foot shot, used to try and throw the basketball from one side of the court to the other and try and get it in the net!!
(and believe it or not one time I actually made it!!!)
Speaking of which… https://youtu.be/ZjwecDcdNYc
Dear Scott,
I like how you used a table to organise your ideas. I also think it was a good idea to use the words ‘demand’ and ‘direct experience’.
The ‘demand’ part solves the issue you tried to address at the end of your post – i.e. LFU assuages the question of why students have to learn a specific skill or a set of knowledge. Knowledge gap is made apparent at the beginning and throughout the learning process. Traditional teaching are instruction centred, hence, it didn’t matter if there were learning gaps.
As for ‘direct experience’, I think you skilfully distilled one of the key characteristics for LFU. STEM learning is about having first hand experience to discover and observe. LFU believes in the fact that student based actions determine knowledge construction.
The idea of studying split frame reminds me of an earlier post (& videos) about using stop-motion as a method of learning. I still have my reservations when using stop-motion as I see them as time consuming activities. However, I do appreciate closely observing a set of motion in small increments. What other topics (especially in math) would you suggest that also deserves this focus?
Alice