My final observation for CATL was completed in a course that I’m very familiar with: ISCI 330A – Symmetry. The instructor for this course is not one of my mentors for CATL, but I’ve been his TA for this course four times, and was a co-instructor with him twice for the version of the course that was run through the Vancouver Summer Program. I also completed one of my practicum lessons for this term in his class. The Symmetry class has about 40 students that are mostly in third year. It is not a required course for Integrated Sciences students, but it can be used as one of a certain number of Integrated Sciences courses that they are required to take for their degree. Because of this, I would think that many of the students are in the course because they want to be, and not because it’s required. This could increase their motivation to do well in the course and participate in the lessons. Also, teaching symmetry naturally involves incorporating active learning into the lesson, so the lesson involved lots of hands-on work identifying symmetry operations in various 3-dimensional shapes.

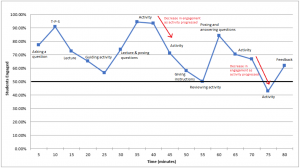
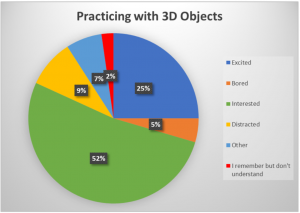
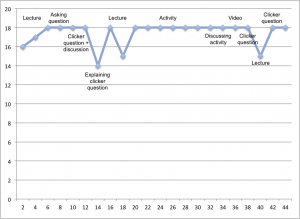
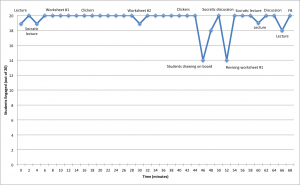
The lesson that I observed was on 3-dimensional point groups and built on the material that I had covered in the previous lesson for my practicum. I’ve also taught this specific lesson in the past, so I was aware of the challenges associated with the content. This class covers something called “roto-inversions”, which is a symmetry operation that involves both rotation and an inversion through the centre of the object. Roto-inversions are not only difficult to comprehend in theory for many students, but they are often difficult to identify in the 3-dimensional objects that students are working with, even after lots of practice. To help students visualize roto-inversions, the instructor showed the students some tricks to identify each type of roto-inversion, instead of just telling students what the different roto-inversions look like. He created representations of the objects on his PowerPoint slides and stepped through the motions sequentially to demonstrate which part of the object had a distinct feature. Then, he showed them real examples of objects that contained each of the roto-inversions they were learning, and reiterated the tricks to identify them. He even built a giant version of a 3-dimensional object containing a 4-fold roto-inversion to make the symmetry operation easier to see. I think showing the objects to the students did help them see the roto-inversions, but students sitting at the back of the classroom may not have gotten a good enough view of the objects to see what was going on. This could be solved by moving around the room with the objects to ensure that all students got a chance to see it up close.

I was happy to see that the instructor has continued to ask ABCD card questions during his lessons. I had first started using ABCD cards in Symmetry last year after one of my mentors suggested I try them out since we didn’t have an iclicker base in the classroom. I then showed them to the instructor and provided him with the file to print out his own cards. He seems interested in continuing to use ABCD cards and has also expressed interest in incorporating iclickers into his lessons in place of the ABCD cards. I’m going to try and get an iclicker base for this classroom so that he can start to use clickers in this course and also in the other course that he teaches in the January semester. Personally, I find iclickers easier to use than ABCD cards, particularly because there are less issues with anonymity. This instructor has never used iclickers before, but he seems eager to try them out and learn the proper techniques to meaningfully incorporate them into a lesson.

In the future, I’d like to keep attending this instructor’s Symmetry classes to see any changes he makes to his lessons. He seems to be starting to incorporate more active learning into his lessons, and I’d like to see how that develops. I’d also like to continue to engage in conversations with him about teaching techniques that he could consider implementing in his courses.

I taught my final practicum lesson for CATL in EOSC 516: Teaching and learning in Earth, Ocean & Atmospheric Sciences. This is a graduate-level course is very similar to the Instructional Skills Workshop (ISW) that is offered at UBC through the Centre for Teaching, Learning & Technology (CTLT) and at other institutions around the world. Unlike the ISW, which is completed within three days, this course is stretched out over 6 weeks. Each week alternates between theme sessions in which the facilitators guide learners through key concepts in teaching and learning, and “mini-lesson cycles”, where each of the learners give a 10 minute mini-lesson on a topic of their choice. There are only 10 students in the course, 1 instructor, and 2 TAs (including myself), creating a unique learning environment in which learners are more comfortable to take risks and challenge themselves as instructors.

I facilitated an hour-long session called “Facilitating Discussions”. The motivation for this session is that instructors, especially new ones, are often intimidated by the thought of using a discussion in their classroom. They might worry that they will try and start a discussion and no one will participate, or that there will be one or a few dominating students that derail the discussion to focus on their own agenda. In this session, learners participate in discussions about discussions that are structured using two different techniques: snowball and debate.

Before starting the main part of the session (the discussions themselves) I asked the learners to reflect on how they’ve seen discussions used in classrooms settings and how they felt during those discussions. They had most commonly experienced the traditional form of discussions where the instructor poses a questions, tells the class to “discuss”, and asks another question when the conversation is exhausted. This is what I had expected, and was why I had planned more unique discussions for them to participate in.

The first discussion technique I used was a snowball discussion. Learners start out thinking on their own about a question, which in this case was “Think about a classroom discussion you’ve experienced. What happened during the discussion and what did the instructor do?”. After learners reflect on this for a minute or so, they pair up with someone and discuss a slightly more complex question (How do you feel about discussions in the classroom?). Next, each pair pairs up with another pair to become a group of 4, and discusses another, slightly more complex question (relating to the strategies used to run an effective discussion, and the challenges that may arise during a discussion). Finally, we returned to the entire class and I asked questions that related to and elaborated on what they had discussed so far, namely the benefits and challenges of using a snowball discussion. Personally, I like this discussion technique because by gradually building up the size of groups it makes the discussion come more naturally, and makes it easy to scaffold the discussion from easier to more complex questions. It is also good for learners that aren’t comfortable speaking in front of the entire class. Most learners will feel comfortable talking to one other person, or a group of 3 other people.

The second discussion technique was a debate, with the two sides being discussion is always good in a classroom, and discussion is never good in a classroom. Before the session started, I had given each learner a coloured piece of paper, intentionally ensuring that people that often worked together were each given a different colour of paper. When the debate started, I let them know that the colour of paper they received represented what team they were on for the debate. Each team was given 5 minutes to plan their argument, following which they each had 4 minutes to argue for their view. That was followed by time to plan the rebuttal, and 2 minutes each to present their final arguments. After the debate, I asked the entire group to discuss the benefits and challenges of using a debate as a discussion structuring technique, similar to how I had led the final large group discussion in the snowball activity, and recorded it on the board. In this way we had the benefits and challenges of the two different techniques used written side-by-side on the board, making it easier for learners to compare the two. I think that a debate is a useful discussion structuring technique for specific situations. The topic being discussed should have two clear sides, and the class size needs to be relatively small, since the debate needs to be moderated and everyone should have a chance to speak. It’s probably a technique that would be better for later in the course when students are more familiar with each other. A challenge with debate is that it could put students that are more introverted in an uncomfortable situation. A way around this that I’ve seen is to give each person on one side of the debate a different coloured sheet of paper, and the same set of colours to the other team (i.e., one person on each team will have the same colour paper). Instead of having an open debate where anyone can speak, the facilitator chooses a colour and that pair has to debate one aspect of the group’s argument. This ensures that the quieter members of the team get a chance to speak.

I didn’t use a feedback form specific to my session because we ask learners for general feedback on the entire session, and I thought a second feedback form would be time consuming and redundant. For the feedback, I asked learners to answer three questions: What helped you learn?, What hindered your learning?, and What was your “shining star” from the session? The debate came up a lot in the feedback. It seems like most people enjoyed it, giving comments such as “helped solidify ideas and concepts”, “liked the debate part, really helped learning”, “debate = motivation”, and “the debate was fun and useful!”. One person felt that it was “painful”, which I’m assuming is because they were uncomfortable trying to push their opinion on the other team. This was an important reminder that debates don’t work well for all learners. The snowball discussion was also mentioned a couple of times in the feedback, with comments such as “helped my learning – I shared a lot more with the group than with the open class” and “helped learning – group pairing after thinking ourselves”.

In terms of my own teaching, my role in this session was to facilitate, so the style was quite different from the practicum lessons that I have given in the past. I think that the breadth of ideas the learners came up with during the session is evidence that I was able to efficiently facilitate and draw out their thoughts and ideas. The list of benefits and challenges of each technique that they generated included all those that I would have identified myself, and some I hadn’t thought of. I think that taking on the role of a facilitator rather than a teacher always presents different challenges but that I am becoming more comfortable with it and continuing to grow as a facilitator and an instructor.

Last week I observed one of my mentors teach a class on Landslides in EOSC 114: The Catastrophic Earth. This is a large, introductory geology course that is often used as the first-year science requirement for many students. The course has two sections – one in the morning and one in the afternoon – and the same instructor teaches both sections. Each section has 150-200 students.

Something interesting about this course is that it is taught by different instructors throughout the term, each of which teaches their own specialty. I see the advantage of this as being that students are taught by leading academics in each of their fields. However the disadvantage is that switching instructors every two weeks makes it very difficult to establish and maintain a classroom environment and classroom guidelines. My mentor was the instructor for the first two weeks of class, during which he established a participatory classroom environment by including lots of clickers questions, small and large group discussion, and activities in each of his classes. Once his section of the course ended, the students were taught two more topics, each by a different instructor. When he returned to the class to teach the landslides portion of the course, he found that students were no longer as willing to participate and got off task much more quickly than before. This was extremely evident during my observation. I sat in near the middle of the lecture hall so that I could also observe the students that were sitting around me. Even though the longest stretch of lecture during the class was ~5 minutes, the students that I was observing would continually get off task after only a minute or so of lecture. Even during think-pair-share (TPS) discussions, the students would discuss the question quickly, and then switch to talking about something unrelated to the course. The only thing that seemed to hold their attention for longer were the YouTube videos of various types of landslides that the instructor would frequently play (likely to regain their attention) and then discuss as a large group. I was really surprised by this because when I’ve observed students in my mentor’s classes in the past, they were extremely engaged (relative to other courses I’ve observed) and eager to participate. Since my mentor was employing the same teaching techniques that he has in the past, this suggests to me that the students were negatively impacted by the constant change in instructors throughout the course.

I think it would be more beneficial to students if this course was taught by only one instructor. The course is a 100-level, introductory geology course, so I don’t think that the material would be too difficult for someone that doesn’t specialize in a particular topic. In fact, I think it can be easier to teach a topic that you don’t specialize in because you can at least somewhat avoid the “expert blind-spot”. I’ve seen this course taught by only one instructor two summers ago. I was a TA for the course and taught one class for the regular instructor when she was out of town. From my experience observing and teaching that class, students were much more engaged and willing to participate, even though each class was 1.5 hours long instead of 50 minutes. However, this could also have been influenced by the course demographic. This offering of the course was during the summer, so the students that were taking the course likely had less classes than they would have during the regular semester. On the other hand, they may have been less excited to be there if they were enrolled in a summer course due to failing a course during the regular term.

In summary, observing this class was an eye-opening experience for me since it showcased how quickly students can get off-task, even during a class that requires a lot of student participation. It emphasized that participatory activities such as iClicker questions and TPS are useful in regaining students’ attention, but may still not result in high engagement during the entire class. It also revealed how strongly a classroom environment can be influenced by an instructor (or in this case, by multiple instructors).

Yesterday I taught a lesson in a third-year Integrated Sciences course: ISCI 330A – Symmetry. I’m quite familiar with this course as I’ve TAed it three times and have also co-taught it twice for the Vancouver Summer Program. Since I was comfortable with the content it allowed me to focus more on the activities and my interaction with students during the lesson.

I invited one of my mentors and another instructor to observe the class and complete Behavioural Engagement Related to Instruction (BERI) observations. Their observations showed that the engagement during the class was lower than in other classes I’ve taught. I noticed this as well as I was teaching the class and tried to make modifications to increase engagement. It was especially apparent during the pure lecture portion of the class during which I could tell students were getting off task and were not as interested in the material. To try to counteract this I ended up rushing through this portion of the class. In hindsight, this was a bad strategy because it came up in my feedback that I had gone through some content too quickly. Furthermore, although speeding through the lecture portion to get to an activity did mean that those students were engaged again at the beginning of the activity, they soon returned to being off-task after they had been working on the activity for ~10 minutes.

This class was the first small class that I’ve taught where I wasn’t also a TA for the course. Being an unfamiliar face to the students may be part of the reason that engagement was lower than it has been in the other classes I’ve taught. Teaching this class really made it evident to me how quickly students can get off-task. It seems like it’s better to alternate frequently between different teaching methods and activities than to have larger chunks of lecture and still include a really long activity. Engagement always increased dramatically when I switched to something new, but it would still decrease during an active exercise. However, that decrease was slower during an activity versus a lecture.

I ended up handing out the feedback form about 5 minutes before the end of class. Giving a bit of extra time to complete the feedback resulted in some very thoughtful feedback from a lot of students. I find that students often rush through feedback forms at the end of class because they have to run to their next class, and the resulting feedback is very brief and fairly uninformative. The extra time I allotted for the feedback was definitely well worth it. The most common comment was that the hands-on practice manipulating 3D objects and identifying their point groups (the biggest LO for the lesson) was helpful, and that the demonstration I gave to the class before the activity helped make this more clear. My rushing through some content was noticed in the feedback, so I’ll have to keep that in mind for my next lesson as I know I’m prone to doing that.

Overall, I had a positive experience teaching this class. I think that the biggest take away was all the detailed feedback I received from the students, which I can use to inform my teaching in my next class.

Last week I completed my second observation for this term in ENVR 200: Introduction to Environmental Science. This fairly small (~40 students) seminar-style course is one of the first that students take after deciding to major in environmental science. During the class I observed, the students were participating in a mock UN climate change negotiation that is run through climateinteractive.org. Teams of students were each assigned a different country or group of countries (USA, other developed countries, India, other developing countries, etc.) and after three rounds of deliberations had to achieve an agreement that would limit the increase in global temperature since the industrial revolution to the desired target of 2 degrees C or less. Each team had to agree on targets such as a carbon emissions peak year, a carbon emissions reduction year and an annual reduction rate, deforestation and afforestation efforts, and billions of dollars per year to contribute/receive to/from the global fund for mitigation and adaption. Before even starting the class, the first thing I think the instructor did to get the class’ attention was to make the team of “other developing countries” sit on the floor instead of at their own table like all the other teams. This not only sent an important message that developing countries often don’t have a fair say in climate negotiations, but also peaked students’ interest in the activity when they realized that some of their classmates were sitting on the floor in the back corner of the room.

For this observation in between the two Signature Pedagogies sessions, we were asked to reflect on two questions during our observation. The first was: “When you are observing the practicum take notes under whether the teacher is trying to get the students to think in a particular way, perform in a particular way and/or care/value in a particular way or about particular things? Offer concrete examples.”

I definitely thought that the instructor was trying to get students to think in a particular way. The instructor was trying to get students to adopt the way, or to reinforce the view they may have already had, that climate change is a real issue and that a plateauing and eventual decrease of carbon emissions is necessary if the human population is to continue to survive. The instructors wants students to value this cause so that they can promote change in the real world. An obvious piece of evidence for this is that there was no debate on if the 2 degree C target was reasonable, or if countries should invest time and resources into combating climate change. The 2 degree target was introduced in a way that made it seem like the right thing to do. The instructor read from a script which said things like: “When I look around I see people that are younger than me and that will have to deal with the consequences of your decisions.”, “Emissions reductions must be achieved that will stabilize temperature reductions well below 2 degrees C warming.”, and “…share the costs of mitigation and adaptation to fund the most vulnerable nations.”. I don’t see a problem with the instructor imposing their views and the views of climateinteractive.org on the students because it is an environmental science class, so I think students should come into the course assuming that the instructor is going to strong views on combating climate change. Of course, if students don’t agree with the instructor’s views, they should be allowed to express their own views in a diplomatic way while backing up their opinions with evidence.

One thing the instructor did somewhat contradicted the values she was trying to get them to adopt: the instructor never told the students that they were allowed to talk to the other teams instead of just negotiating within their own team. This likely resulted in it taking more time for the teams to reach an agreement, contradicting the value that climate change is an important issue and should be tackled with the best possible plan of action. Halfway through the second round of deliberations, the students discovered on their own that they were allowed to talk to the other teams, but a better agreement (i.e., less of an increase in global temperature) may have been able to be reached if they had spoken to one another sooner.

The second question we were asked to reflect on was: “When you are observing the practicum take notes under what the instructor is doing (or having the students do) to teach the students the practices of the discipline through thinking, performance or caring (could be all)?“

The instructor taught students about the practices of the discipline in the following ways:

• A debate was likely selected as the activity for this class because they tend to get students invested in what they’re arguing for.
• Having a variety of countries participate in the debate showed students that when assessing climate change you have to look at many different players, not just the big first world countries. Developing countries and countries with power but that have different views from many western countries (e.g., China and India) were included in the debate to get students to think of a variety of view points when considering how to best curb global warming. Speaking to and trying to reach agreements with different parties is a key practice in environmental science.
• The pre-readings for the class contained mentions of disciplinary values as they stated that greenhouse gas emissions needed to be reduced by half.

This was a really interesting class for me to attend since, although it is not my discipline, I’ve always enjoyed learning about environmental science and seeing how other people view climate change and society’s role in it. I am a TA for a sustainability course in the Integrated Sciences Department, so I’m hoping that I can incorporate this activity into that class next year!

Last week I taught a class about geologic time in EOSC 110: The Solid Earth. This was the same class offered through Vantage College that I had observed two days earlier. Since this is a small class I decided to try a new activity since a smaller group of students would be easier to monitor to make sure an activity is going smoothly. I also thought it would be a good environment to try a new activity in because it’s a first year course and I was hoping that students with less university experience would be more forgiving if something didn’t go as planned. I also asked the instructor of the course to complete a BERI observation to monitor their level of engagement throughout the class.

The activity required students to construct a geologic time scale by placing key events in Earth’s history in order from oldest to most recent. Many of the events were the evolution of certain species, plus key features in Earth evolution that I thought they would have heard about without taking other geology courses. Examples of these include the appearance of species such as dinosaurs, birds and fish, the first appearance of macroscopic organisms and organisms with hard-parts, and the first fossil and oldest rock. I printed out cards with a picture of the events and a short description. Since there were 32 students in the class, I made two sets of 16 cards so that there would be one event for every student. I split them into two groups by giving everyone a small piece of coloured paper when they came into class. Since I handed out alternating colours it made sure that they wouldn’t end up with all the people they knew in the class, and would have to work with some people they didn’t know as well.

Before starting the activity I gave them a brief introduction to the age of the Earth and the concept of absolute age dating. From my pre-assessment (simply asking them how old the Earth was) I knew that everyone knew at least a little bit about Earth history, so I felt comfortable asking them to complete this activity. I gave them about 10 minutes to tape all the cards up on the wall in order from oldest to youngest, then we moved back to the desks and I revealed the correct order while occasionally asking them to tell me which event came next. I also tried to explain any common misconceptions, e.g., that mammals evolved after dinosaurs had gone extinct.

The results of the BERI protocol were somewhat surprising: the lowest point of engagement was not during the lecture, as I would expect, but while I was explaining the answer to a clicker question. However the instructor did note that they were likely chatting to each other, and therefore not engaged according to the BERI protocol, because they were surprised by the correct answer, so their perceived lack of engagement at that point may not have been a bad thing. Overall I think that I maintained a high level of engagement, and since I’m using that as a proxy for motivation, a high level of motivation throughout the class. Overall I had lower engagement than in my last practicum, but I think that some of that can be attributed to this being a first-year course in which students tend to be less engaged and motivated. Still, I can constantly try to increase the level of engagement in classes of all years that I teach in the future.

I also asked students to complete an anonymous feedback form at the end of class. It was very similar to the one I used for my last practicum, with questions about how they felt during the activity and one of the main lecture topics, as well as what they liked and what they would change about the class. Overall, most students felt “excited” or “interested” during the activity on geologic time and while learning about absolute age dating during the lecture. The vast majority of them said that the activity was the most interesting part of the lesson, as while as a video about earth history that I showed immediately after going over the answers to the activity. The main criticism they had was that I went through the material on relative age dating very quickly. I had thought that this part of the class was too fast, so it was good that their feedback backed up what I already thought. After doing the activity, there wasn’t a lot of time left to get through the rest of the lecture material, and I also wanted to make sure I had time to do my feedback form, so I ended up rushing through the slides and assigning some of the small questions I had intended for them to do in the class as homework. If I ever have the chance to teach this class again, I would cut out some of the material from earlier in the class to make sure that I could thoroughly cover the second half of the material, and also have time to do some of the examples I had planned in class.

There were two main benefits that I gained by teaching this particular class and group of students. First of all, I had already taught a class in the regular version of EOSC 110 for my practicum last term, so although this time was a different topic, teaching the same class gave me the ability to see if some things worked better in a smaller or larger class, such as clicker questions, discussions, and types of activities. I thought it was much easier to gauge when I had given enough time for a clicker question discussion, but that could also be because I’m getting better at clicker “choreography” (my mentor also mentioned this in our meeting after the class). This Vantage College class was also unique because all of the students have learned English as a second language, so I had to be conscious of what language and expressions I used to make sure that the concept was not lost in too much jargon. It also meant that a couple of my attempted jokes fell flat, but that sometimes happens with fluent English-speakers :). The second benefit was that it was a very small class compared to how big first-year Earth Science courses at UBC normally are, so it was easier to try a more complicated activity. It was exciting to try something that required a bit more preparation rather than just a worksheet that’s normally done for the class on geologic time. The classroom could also hold a lot more students than were in the course, leaving lots of room for the two groups to spread out for the activity, so it was even easier to implement than it would have been in a normal class setting. Overall, I’m happy with the how the class went and have some ideas on how I would improve it for next time.

Yesterday I observed a class in EOSC 110 – The Solid Earth: A Dynamic Planet, offered through UBC’s Vantage College. EOSC 110 is normally offered in the Department of Earth, Ocean & Atmospheric Sciences (EOAS) where it has an enrolment of up to around 300 students, whereas the Vantage College course is much smaller with just over 30 students. Since I’m used to observing high enrolment first year EOAS courses, such as 110, it was a good contrast to see a similar course in a small class setting.

One thing I noticed immediately when arriving for class was the unique setup of the classroom. It had a few tables, like most smaller classrooms do, but then there were bigger lounge chairs, couches along the back wall, and higher tables and chairs around the perimeter. It’s an interesting idea to make a classroom more comfortable and have a more relaxed feeling; on one hand it could put students in a better mood and being more comfortable could make it easier to pay attention but on the other the unique setting could be distracting, at least for the first few classes, or even make students feel sleepy. One big advantage of a classroom like this is, which was aided by the small class size compared to a room that could hold many more students, was that there was lots of space to spread out to do activities. There was enough room that the instructor could have two separate spaces: one for traditional lecture and another for participatory learning activities. Since I’m going to be teaching this class tomorrow, seeing the different classroom made me excited to try a new activity that I knew would require a fair bit of space.

The class itself was on natural resources. Even though the students hadn’t yet been introduced to natural resources, the instructor started off the lesson by getting them to brainstorm a list of natural resources in small groups. The instructor then compiled a list of the ~15 resources the students had come up with on the whiteboard, and then asked them to pick one and discuss the benefits and drawbacks of using/extracting that resource. I thought starting the class like this was beneficial to the students because it showed them that they had the ability to define and assess natural resources on their own, before any instruction. Once the lesson shifted to more content delivery, students were likely more motivated to learn since they had already been engaged with the material and discovered some of the content on their own.

Another interesting thing that the instructor did in this class was with one of his clicker questions. One of the answers to the question was a common misconception. He didn’t reveal if many students had selected that answer but he made a point to explain why that answer was wrong and why it was often mistaken for the right answer, and then had a short discussion with the class about it. Clarifying misconceptions is important not only to ensure that students understand why the misconception is incorrect, but also to reinforce that if they chose that incorrect answer, they’re not the only ones who did and it’s a common mistake to make. The discussion with the students should help to ensure that they remember what the correct answer was and why the other answer is wrong.

A final thing I noticed about the instructor’s lecture slides was that throughout them there were links to YouTube videos that students were encouraged to watch later. YouTube videos are a fun tool that can be used to cover more content that may not have been intended to be part of the class, or to reinforce more challenging concepts. Plus, they’re far more interesting that reading an article on the topic so it’s much more likely that students will revisit them later.

This observation was really useful for me because it gave me the opportunity to meet some of the students that I’ll be teaching in my next practicum and it gave me an idea about how much prior knowledge they are coming into the lesson with. It was also helpful to see the classroom: I’m sure that will make my activity run more smoothly!

My first lesson of my practicum for this term was in ISCI 360: Systems Approaches to Regional Sustainability. This is a course that I am a TAing for the third time that is composed entirely of guest lecturers on various topics relating to sustainability. The topic of my lesson was systems thinking with an introduction to earth systems science, something that is a bit out of my comfort zone. The learning goals I wrote for the class were as follows:

1. Describe the basic properties of systems.
2. Solve stock and flow problems graphically.
3. Apply the earth systems science model when analyzing sustainability and appreciate the interconnectedness of earth systems.

As part of my SoTL proposal I wanted to assess student engagement as a proxy for motivation. To do this, I used the Behavioural Engagement Related to Instruction (BERI) observation tool, developed by Lane & Harris (2015). This tool was recommended to me by one of my mentors and was developed by my supervisor for my geoscience education portion of my thesis project. The tool uses student behaviour to gauge whether students are engaged or disengaged. For example, engaged behaviour could be following along with the lecture on computer or taking notes in a word document, while corresponding disengaged behaviour would be surfing the web, checking email, or online chatting. Observers each take a subset of students from the class and note the number of those students that are disengaged at 2-5 minute intervals, depending on how experienced they are with observing students. They also note the type of activity that was occurring during the time interval (lecture, discussion, activity, etc.), any relevant instructor actions (questioning, humour, real-world examples, etc.), and any extenuating circumstances (outside noise, technical issues, etc.). Two of my mentors were able to attend the lesson, and the course instructor was also able to observe students, so I was confident that they would be able to sample a large enough subset of the class to get an accurate representation of their overall engagement.

The figure below shows the compiled data from the three observers normalized to 20 students (each person was observing 12-20 students each, depending on their positioning in the classroom and how many students they felt they could accurately observe). It clearly shows that students were engaged for the majority of the class. The lowest points of engagement were during “students drawing on board” and “revisiting worksheet #2”. The first low point of engagement occurred when I had asked a group of students to come up to the board and explain how they got their answer to a question on one of the worksheets. A significant proportion of the class wasn’t paying attention when they were writing on the board and the observers noted that there were still students in the back of the class that didn’t seem to understand how the answer was determined. For next time, I’ll try showing the answer on the document camera instead because part of the reason for the low engagement could have been that the blackboard was too far away and hard to read for some students. I could have also included the answer in my PowerPoint slides, like I had done for the other two previous questions. The other point of low engagement was when I revisited a worksheet from earlier in the class. The reason for revisiting this worksheet was to clarify misconceptions that most people have about how reducing greenhouse gas emissions affects the stock of greenhouse gases in the atmosphere. I hadn’t considered that my students had read a pre-class reading that should have changed their preconceptions about the stocks and flows of greenhouse gases, so they didn’t need any time to revise their answers. Luckily I was able to quickly assess that students didn’t want any more time with the worksheet and we moved on to the next part of the lesson.

I also got students to fill out a short feedback form at the end of the lesson that contained questions about their engagement during various parts of the lesson. Some of the questions I asked were, “How did you feel during the bathtub activity?” with the options for them to select being excited, bored, interested, distracted, or other (fill in the blank). They could also choose if they couldn’t remember the activity, or if they could remember the activity but didn’t understand the point of it. Most of the feedback I received was that the activities made students feel excited or interested about the topic. Interestingly, a few students thought that the most interesting part of the lecture was the short discussion on the earth spheres, so for next time I would make that discussion longer.

I met with both of my mentors after the class to discuss how it went. One noted that my iClicker “choreography” had improved, which was something he had suggested I work on from one of my term 1 classes. I knew when a discussion was needed after a clicker question and when the question was easier and could be quickly answered by the students. Both my mentors noted that I was confident during the class and didn’t seem nervous, which is something I’m continually trying to improve on. Some things I can work on for next time are not using the phrase “if you want…” because students will automatically tune out. I also have to work on regaining the class’ attention after working on an activity. This time I tried to start talking over students after I couldn’t get their attention to start the lecture again, but they noted that some students that are trying to pay attention could get annoyed at their classmates for talking, so it’s better to wait and call on people to be quiet multiple times if necessary. Finally, during one of the activities there were two groups of students that were finished ahead of the others and ended up becoming disengaged because I didn’t have anything else for them to do. In the future I can use those students as a resource by splitting them up and getting them to help other students that are still struggling with the activity.

Overall, I think did a good job delivering the content while at the same time incorporating lots of activities and class participation, and I can definitely see things that I have improved on from my term 1 practicums. I will use my next practicum to work on the finer points of the lesson, such as effectively regaining students’ attention and being extra cognizant of the way I introduce activities and discussions to ensure that they don’t sound optional.

I gave my second lecture for my practicum in EOSC 110, which is a core 100-level geoscience course on the Earth’s origin, composition, structure, and natural resources. This is a large class of over 200 students, making it a stark contrast to my first lecture that was a smaller class of only 40 students. This was an intentional choice on my part to ensure that I gained experience in a variety of class sizes.

This lesson was particularly challenging for me because it was on a subject that I’m not very comfortable with and struggled with when I learned about it during my own undergraduate degree: geophysics, specifically the structure of the Earth’s interior and its magnetic field. Therefore my knowledge of the material before I started preparing for the lecture was probably not that far above that of the students, and I had certainly not attained the level of an expert by the time I delivered the lesson. I hope that this worked to my advantage in that I avoided some expert blind-spots that I may have fallen into in a subject that I was more familiar with.

I think the biggest challenge for me during this lesson was trying to juggle the use of a PowerPoint, iClicker software, and YouTube videos, all while trying to keep 200+ students engaged. In the very first part of the lesson, I was so focused on not forgetting any of the content I had to deliver that I missed opportunities to ask the class questions as I went along. Later in the lesson, when I started asking more questions I had a lot of trouble getting students to answer them. This may have been because I hadn’t starting asking questions and encouraging student participation from the very beginning. That being said, something that I think I did particularly well in this lesson was to not back down when I didn’t immediately get a student volunteer to answer my question. Instead I just continued to look around the room, even though it sometimes felt like minutes had gone by since I first asked the question, and eventually a student would raise their hand. As I progressed through the lesson I started trying new ways to get students to answer questions, such as by specifically asking someone from the back of the room to answer. If these were students that I was more familiar with and had been teaching for longer, I may have resorted to calling on a student, but I wasn’t comfortable doing that with a new group of students.

At one point during the lesson a student answered one of my questions with an explanation that I didn’t fully understand, and that in my mind seemed overly complicated to explain the concept. I was tripped up by his answer and felt that if I didn’t completely understand it then it was likely that at least one other person in the class was also confused and was looking for an alternative explanation. This prompted me to ask the class for an alternative, simpler explanation of the same concept. After doing this I realized that it may have made the first student feel like their answer wasn’t adequate enough, even though they were correct, and that they may have felt embarrassed. I mentioned this to my mentor in our meeting and he said he knows the student and highly doubted that the student was affected by it. Regardless, I think this situation was a good reminder to always put myself in the students’ place and I’ll make sure to remember it in the future.

I ended the class with an activity that tied the two parts of the lesson together by comparing the structure Earth to other planets and relating that to their magnetic fields, or lack thereof. This gave students the chance to apply what they’d learned during the lesson and to integrate their knowledge of both concepts. I left enough time at the end of the lesson for all students to complete the activity and hand it in at the end. This was something that I had worried about because I thought I might have too much content for the allotted time.

In my meeting with my mentor, he said that my lesson went really well, especially since it was only my second time teaching a large class (and third lesson given on my own). He said that I had a good tone of voice and pace, and that he liked how I tried to try to make small jokes to make students feel more comfortable. Although that was unintentional and usually happened because of my nerves, I’m glad that he thought they had a positive outcome. The main thing I have to work on are the details of how I administer clicker questions.

Some things that my mentor mentioned were that I often gave 45-60 seconds to answer a question, whereas he suggested that 25-30 seconds is sufficient. This is because students will often “race” to submit their answers when you give them a 5 second warning, regardless of how long the poll was open for. Also, at the beginning of the lesson I got students to pair up and discuss their answers after all the clicker questions, telling them to “turn to your neighbour and convince them that you are right”, and then re-vote on the answer. I’d actually decided to do this because in the lecture of his that I observed I noticed that he told students to discuss their answers after each clicker question. It turns out in all those cases not a high enough number of students had gotten the answer right, so discussions were needed. He suggested only having a discussion when <60-70% of students got the right answer. Having a discussion after every question risks that clicker questions will start to seem redundant, and that students may start thinking they’re a waste of time. To justify the need for discussion when students perform poorly on a question, he suggested I could explain why a discussion is needed by saying things like, “There was a really good split between answers for this question so discuss it with your neighbour and vote again”, or, “A lot of you could benefit from a discussion of this question”. Finally, he suggested ways to get students to volunteer to explain why they voted for a certain answer to a question. Instead of trying to ask someone that chose the wrong answer to explain their reasoning (which is very unlikely to happen in front of the whole class) I could say things like, “Why do you think someone chose this answer?”. Then even someone who got the right answer could volunteer to explain why someone might choose another answer. If this still doesn’t work to get a volunteer, I could instead ask them to discuss my question with their neighbour.

Overall, I think this lesson was a great experience for me to practice teaching large class sizes. For my next lesson using clickers, I am confident that I will be able to use the new techniques I learned so that I can use them more effectively and ensure that students see the value in clicker questions.

In your next teaching practicum:

Identify a connection between something in the world that you are trying to explain and a theory that is used to explain that.

In my next teaching practicum, I will be trying to explain how geologists have inferred the structure of the Earth’s interior using the theory that seismic waves will reflect and refract at boundaries between layers of varying composition and density. This is a difficult concept to explain since we cannot see the interior of the Earth so students have to accept this theory as proof that the interior of the Earth is highly differentiated.

How are you helping your students make that connection?

Reflection is a common concept that is generally well understood so the focus in explaining this theory will be on refraction. A common example of refraction is how a pencil “breaks” when it is placed in water because the light is refracting as it travels from air to water. This is a good example to use because students will have likely seen this effect in their daily lives.

This theory will also be explained using P- and S-wave shadow zones. Students will be shown images of where on Earth P- and S-waves would be detected if an earthquake that originates at point X could generate seismic waves that could travel through the entire Earth. The connection here is not as strong because we cannot model that S-waves can’t travel through liquids, which is the evidence used to explain the S-wave shadow zone. If they accept this they can then understand that the outer core must be a liquid to create the size of the observed S-wave shadow zone.

Does your process help your students be able to make connections beyond that particular exercise? How does it do that? and if not, what can you do to help them be able to do that?

I found this question difficult to answer since I’m only giving one lecture in the course and will not be interacting with the students after that, so I think it’s hard to say if they will make connections with this theory after my lecture. I think the use of a real-world example (the pencil in water) is memorable and I hope that students will think of that example when they have to apply refraction in other situations. Also, if students decide to continue studying geology, the concept of a differentiated Earth will be referred to time and time again and they will learn countless more examples that support this theory. Specific to my lecture, I will also be talking about the Earth’s magnetic field, which can only occur because the Earth is differentiated. This is a second example that requires the Earth to be differentiated and will hopefully allow students to make connections between seismic refraction and the Earth’s magnetic field.

In your next Observation:

If the instructor is talking about a concrete experience or phenomenon, are they making connections between that concrete experience and a theory in their discipline? If yes, how?

Rhy was explaining how the rock record of the Palaeozoic changed through time with the change in marine life. This is a real phenomenon because geologists have noted real changes in the rock record and they have attributed that to the amount of hard parts that marine animals possessed at that time. Rhy did a good job of connecting this idea to the real world by using humans as an example of how organisms would be preserved in the rock record based on the compositions of various hard parts in our bodies.

Does the activity they are using to do either of the above cases help the students make connections beyond the particular application they having students experience?

The activity was designed to get students to make connections between the composition, fossil content, and age of a hypothetical rock. Students were split into small groups that fell into four different age ranges based on the row they were sitting in in the classroom, with the oldest rocks at the front and the youngest in the back. They had to use their clickers to share information about their rock with the class. While other groups were providing information to the class, the students were expected to make notes so that by the end of the activity they would have a picture of the entire Palaeozoic. I thought using clickers was a really great way to share information in a large class. It would obviously be better if students could form new groups and share their information with their new group members but with 80+ students it would likely be a logistical nightmare. Once students saw the results of all the data from the 4 groups, they could see how the rock record evolved through time and how the composition of the rocks was dependant on the types of fossils they contained. I think this idea has broader applications because it demonstrates how a rock can be “dissected” to provide a wealth of information about extinct organisms, paleo-geology and even paleo-climate. It also gives students insight into how palaeontologists were able to recreate the biology of extinct organisms using the rock record.