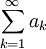Previous: A Second Motivating Problem for The Integral Test
Next: An Integral Test Flowchart
Based on our two previous examples, you may have noticed that we were able to establish that the series converged or diverged because
- our functions were positive on some interval
- our functions were decreasing on some interval
In general, when applying the integral test, we do require these conditions, and also that our function is continuous. The conditions come out of the proof of the integral test.
The Integral Test
The integral test is given by the following theorem.
| Theorem: The Integral Test |
|---|
|
Given the infinite series
if we can find a function f(x) such that an=f(n) and that is continuous, positive, and decreasing on [1,∞), then the given series is convergent if and only if
converges. |
Discussion
The integral test tells us that if the improper integral
is convergent (that is, it is equal to a finite number), then the infinite series is convergent. If the improper integral is divergent (equals positive or negative infinity), then the infinite series is divergent. There are of course certain conditions needed to apply the integral test. Our function f must be positive, continuous, and decreasing, and must be related to our infinite series through the relation 
. However: when we encounter an infinite series that does not meet our criteria to apply the integral test, we can sometimes use some algebraic manipulation. We will explore this concept in a later example.
Previous: A Second Motivating Problem for The Integral Test
Next: An Integral Test Flowchart
