[previous] [next]
In the following problem, we don't work out details on applying convergence tests. Rather, we discuss general strategies for convergence testing.
Problem
State what convergence test you would use to determine whether the following series converge, and explain why:
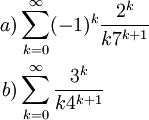
Complete Solutions (and Discussion)
Part a)
Because of the (-1)k term, the series is alternating, so we can try using the alternating series test (the ratio test could also be applied, but would require slightly more work to compute the ratio).
Part b)
Unlike Part a), we do not have an alternating series, but the presence of constants raised to the power k suggests that the ratio test could be applied.
Possible Challenges
Applying the Divergence Test
You may have noticed that in parts a) and b) that the divergence test yields an inconclusive result. Because the divergence test is easy to apply, you may find that it is one of the first tests you tend to think about, but it isn't one that can tell us when a series converges.
Not Recognizing The Form of the Series
Knowing how to classify a given infinite series requires being able to recognize what components of the series are helpful. If we consider Part a):
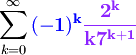
The (-1)k term (highlighted in blue) suggests that we might try using the alternating series test. Other components (highlighted in purple) tell us what the outcome of the test will be.
[previous] [next]


Well now… for part a) I would have just used the absolute convergence test… the absolute series converges too. In fact, the absolute series of part a) and the series in part b) take the exact same form.
The absolute series’ are easy to determine. Each has a ‘k’ in the denominator, which makes each term smaller than the a^k / b^(k+1) form that comprises the other factor. So by direct comparison, if a^k / b^(k+1) converge then so do the absolute series’.
Lastly, the remaining factor is a convergent geometric series.