Initially, I found it challenging to apply T-GEM to mathematics as I found it hard to picture, but after completing this activity, it seems to be very similar to many of the activities I already strive to do with my students. A challenging concept for my students in secondary math is creating and understanding equations for lines and quadratic polynomials using graphs or scenario details. This challenge has been identified through concept pre-assessments, the nature of their questions throughout their work, and continued struggles on summative assessments. They struggle to make the connection between the information they are given and the algebraic, abstract representation.
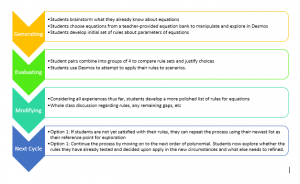
Generating: For the generating phase, I would have students brainstorm what they already think they know about equations with regards to what they mean and how they can be used. Students will randomly select equations from a centralized equation bank to explore using Desmos (available either online or as an app). Working in pairs, students will develop a set of rules for creating equations based on their explorations of manipulating equations within Desmos.
Evaluating: Once students have developed their guidelines, pairs of pairs will be joined into groups of 4, in which students will compare their initial hypotheses and justify their perspectives. Ideally, reflection would occur as students need to explain how they arrived at particular conclusions. At this point, scenario-based problems will be introduced to the original pairs, expanding upon the initial work with base equations. Students now need to determine if the rules they established in their initial phases apply appropriately to their new scenarios. If they are not able to use their rules to accurately create an equation to represent the scenario and use it to solve problems, they will need to identify the gaps and determine what adjustments need to be made. Desmos will continue to be the technology tool at this level, as students are able to easily test, adjust, and visualize their inputs.
Modifying: In their pairs, students will reevaluate their list of rules for equations, taking into consideration their initial hypotheses, their discussions with peers, and their testing of their hypotheses. As a class, we would come back together for a large class discussion to compile their ideas into a community-based understanding.
This process could be further expanded to include different types of polynomials. For example, students initially working with equations of lines could then generate, evaluate, and modify new hypotheses regarding quadratics, based on their work with linear relationships. Subsequently, work with quadratics could be further cycled to work with cubics, then quartics and other higher order polynomials, as appropriate.
By having students use Desmos to work with the different parameters of the equations, they are able to actually experiment to see the effects of changes, rather than simply being told to memorize, for example, that the c value of a quadratic equation determines the vertical position of the graph but doesn’t directly affect its shape when a and b stay constant.
I believe that many mathematical principles and concepts can be approached using similar strategies to those used for scientific principles and concepts, and aim to include them when possible in my teaching. One challenge I have in senior math is the perception of teachers regarding the comparable value of experimentation and hands-on math in the university-bound courses as compared to the middle school or college/workplace-bound courses. I am often met with resistance from colleagues who don’t believe there is a place for experimental or hands-on learning in the higher-level university-bound courses, and that such activities are frivolous at that level. Do you feel that there is value in hands-on math learning for the senior level university-bound math students?
Sources Consulted:
Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905
Khan, S. (2010). New pedagogies for teaching with computer simulations. Journal of Science Education and Technology, 20(3), 215-232.
Hi Stephanie, Love, love, love this idea for senior math. Having students initially start in pairs, then go to s super-group, then back to pairs again, is also a terrific idea. What is really great about Desmos, is that you can turn this into a Desmos lesson yourself, that can then be shared with the rest of us. I have never made a Desmos lesson using their portal, but once I have free time again I will give it a go– it is definitely on my teacher bucket list. ~~~ Last week, I asked a First Year Physics professor if he had ever used Peer Instruction in his class. He had just finished explaining how frustrating it is for him to see 1/4 of his class choose a completely, nonsensical answer for a conceptual physical question. I then explained to him the process of Peer Instruction, and I was immediately shown a look of perplexity/horror. “Why would I permit students to talk to each other during a lecture, and risk them teaching them the incorrect information? I KNOW when I explain it, that it is correct.” Perhaps with some topics, Peer Instruction, GEM, Inquiry, etc. is better suited than others. Although I am always keen to try something new in my approach, not all of us are. And yes, academic Math, Physics, Chemistry, and Biology teachers do have a responsibility to prepare their students for the next level. I do not think that we have to sugar coat everything we teach, either. However, as educators, we KNOW when something really works in our classrooms. It is too bad that some folks aren’t willing to at least TRY and mix up their approach— I think that most traditionalists would surprise themselves! One thing that I have morphed towards over the years is not showering my students with heaps of practice. I tend to give a minimal amount of Practice, with further suggested questions if needed. Then, in exchange, I am using a significant portion of my class time, orchestrating reinforcement activities. My students get very little time in class to work on Practice, but instead, they are getting their misconceptions dealt with in class, and hopefully, immediately. So yes!!! I do see the value in hands-on, social learning in academic math class. It just need to be balanced. Cheers, Dana
I have learned about making lessons in Desmos, but I have never actually done it. I am thinking this activity may form the basis of my final project for this course, so I am hoping to be able to explore the program capabilities further. I would love to be able to create something with the program that removes some of the dependence on the physical teacher in the moment.
Thanks for the thoughtful comment!
Great Stephanie to post a math/STEM T-GEM along with others in the class. (For some reason, the image is not appearing on my screen)… I agree that, “A challenging concept for my students in secondary math is creating and understanding equations for lines and quadratic polynomials using graphs or scenario details.” Indeed, identifying when and where to use slope and for what purpose is a challenge for students yet useful for problems in practice. I like how students socially construct rules: Working in pairs, students will develop a set of rules for creating equations based on their explorations of manipulating equations available within Desmos. It seems that in the evaluation phase, the scenarios provided will be key. Can you share how you plan to match the equations available in Desmos that they have explored to generate rules? Are the rules new equations from Desmos, and do the scenarios permit them to apply it? Also, will an anomalous scenario be including? This is a wonderful opportunity for us in math/STEM education to think about how to engage students in generating and modifying rules in math. Thank you for the contribution of this prescient example, Samia
Hi Samia,
I tweaked the image format a bit so perhaps it will show up now. It has been showing up on my end since the original post, so I’m not sure where the error is.
I envision the provision of equations to be teacher-generated, either within the program itself or as a type of deck of cards. This ensures that students have an opportunity to work with a range of circumstances, including fractional slopes or intercepts, z-intercepts, positives and negatives. Ideally, students would work with positive-value and whole number values of slope and y-intercept, then the other situations of negative and fractional values would each provide anamalous conditions that would require additional confirmation or exploration. With the deck-of-cards style provision of the equations, this progression could be accomplished through colour-coding, with different colours secretly denoting different changes to the nature of the equations. For example, students may be instructed to first choose 5 red cards, then 5 purple cards, then 5 green cards, or something similar. In the context of quadratics and higher order polynomials, there are additional opportunities to expand the impact of the variables involved.
Thank you for doing a math post! Even though I am primarily a math teacher, I find myself sliding towards science activities in my posts because they feel like the frameworks lend themselves more to science. I am also still trying to ‘crack the code’ when it comes to authentic inquiry based project work in math. Have you had any good experiences with that? I always hear people give the example of commerce/finance, but that only takes you so far and covers a few concepts. Thanks again for your post!
I have some ideas, I just want to find the resources I used in the classroom so I can best reply. I will come back to this post.
Thanks for the interest.