Author Archives: STEPHANIE IVES

Desmos TELE – Graphs Galore

I have designed a series of activities for students exploring parameters of linear, quadratic, and cubic relations. The student version can be accessed at https://student.desmos.com/?prepopulateCode=zst5x and the teacher version (which can be cloned and edited) can be accessed at https://teacher.desmos.com/activitybuilder/custom/58e6e9fa3e588a060f483868.

My accompanying guide is attached to this post at the following hyperlink Ives 533 Final TELE Project

I would love to hear from anyone who has an opportunity to check it out or even try it.

Sketching Transformations

I have come to recognize that many of my senior math students do not make a connection between transformations and algebraic processes. They struggle to make the connection between physical movement and algebraic effect. This activity is intended to assist these students in building connections in this area.

Step 1 – Review Cartesian planes and coordinate systems
1. Access prior knowledge
2. Identify any misconceptions or gaps in learning that should be addressed in these areas before moving forward.
Step 2 – Student Hypothesis
1. Working in pairs, students will create rules for translations, reflections, and rotations while working with coordinates (e.g. when translating horizontally, the x-coordinate of each point is adjusted accordingly)
2. While working on developing their hypotheses, students have access to a tabletop grid and shape cutouts to manipulate
Step 3 – Test hypothesis
1. Use Geometer’s Sketchpad to test hypothesis using prescribed “test” transformations
2. Students access transformations from a list within the Geometer’s Sketchpad file
3. Transformations will include confounding situations, such as a rotation in the opposite direction or a reflection in something other than an axis.
Step 4 – Refine rules/hypothesis in consultation with a small group
1. Students combine into groups of 3-5 students to discuss findings, inconsistencies, confirmations, etc
2. Groups come to an agreed upon set of rules by discussing and justifying their perspectives.
Step 5 – Use transformation rules to design a patterning activity for other groups in class
1. Each group will use Geometer’s Sketchpad to design a problem scenario involving transformations that will require other groups to apply their transformation rules.
2. Examples of problem scenarios could include designing a quilt pattern, wall or floor tiling, yard landscaping, etc. The scenario context will be an essential component of the framing of the application because “contexts allow the learner to reflect on and control for the meaning and reasonableness of their developing ideas” (Dixon, 1997, p. 140).

Dixon, J. K. (1997). Computer use and visualization in students’ construction of reflection and rotation concepts.School Science and Mathematics, 97(7), 352-358.

The Whole is Greater than the Sum of Its Parts – GLOBE and Virtual Field Trips

How can learning be distributed and accelerated with access to digital resources and specialized tools and what are several implications of learning of math and science just in time and on demand?

Digital resources and specialized tools expand the diversity of opportunities available to students and teachers. Limitations such as financial resources, geographic location, and student circumstances can be at least somewhat addressed through digital options. The building of learning communities helps promote distributed learning. Magdalene Lampert (1990) explains that a participation structure has been defined by Florio, Erickson, and Shultz as “the allocation of interactional rights and obligations among participants in a social event; it represents the consensual expectations of the participants about what they are supposed to be doing together, their relative rights and duties in accomplishing tasks, and the range of behaviours appropriate in the event” (p. 34). Her article is focused on the social and student-led creation and experience of learning mathematics. Community and discourse is central to her approach. This process and hypothesis-testing approach to learning mathematics can also serve to accelerate learning by enabling students to truly understand the learning process and the content, thereby increasing the likelihood of effective application.

GLOBE is an impressive application of learning that is distributed through a community. Scientists and experts offer training to teachers and support and opportunity to students, and students are able to provide raw data for scientific projects. According to Butler and Macgregor (2003), “Students and teachers benefit from the scientists not only as sources of knowledge and modelers of scientific reasoning but also an inspiration and role models for students who may choose to pursue careers in science and technology” (p. 18). Students participating in GLOBE projects have a real and authentic purpose for their work, which should increase engagement and thereby encourage efficient use of class time and deeper student learning both inside and outside of the classroom. They have a real opportunity to be a valuable part of the scientific process. Additionally, there is an opportunity for classes from various locations to team up on a project, thereby enabling each group to learn from the others and to share their own learning. A true community forms as students, educators, and researchers are each able to be teachers and learners.

As a rural teacher, virtual field trips and webcams stand out to be as an excellent opportunity to engage in visual and experiential learning despite challenges of location, time, or money. Being able to watch animal behaviour on camera, explore an otherwise inaccessible location, and interact with experts enables students to develop understandings that would not otherwise be readily available. I agree with the students in J. Spicer and J. Stratford’s study, however, who felt that the occurrence of real field trips and virtual field trips should not be mutually exclusive. “[I]nstead of allowing VFTs to be thought of as alternatives to ‘real’ field trips perhaps it would be best to explore how a VFT might either enhance preparations for a real field trip and act as a revision tool after a field trip, both approaches potentially giving ‘value-added’ to the real field trip” (Spicer & Stratford, 2001, p.352). From a business perspective, virtual field trips can be more cost effective; however, the experience is not the same. Both virtual field trips and real field trips offer students valuable learning experiences, but these experiences could be best implemented in complement to one another, as opposed to in place of one another, wherever possible.

As a whole, student learning will be accelerated by experiences that enable them to make insightful connections, understanding the reasoning behind learning, and feel like they are part of something greater than themselves. Distributed cognition in which different participants in learning have different strengths and understandings to offer helps to reinforce the value of community in learning. An effective teacher will recognize and create opportunities for experts to be involved in lessons. Each member of the learning community can improve the learning experience, and the larger the community is, the greater the power of the distributed knowledge. The whole is stronger than the sum of its parts. Learning math and science on demand and just in time simulates the scientific and mathematical research processes in which research is conducted in response to a need. As students are able to learn in timely contexts, they can be better able to make connections between what they are learning and the applications and importance of it. This can be a challenge, however, when a need arises and the community of support is not available or accessible. In these situations, students must be confident that they can rely on their own understanding of the learning process and their previous knowledge to help them explore solutions.

Resources

Butler, D.M., & MacGregor, I.D. (2003). GLOBE: Science and education. Journal of Geoscience Education, 51(1), 9-20.

Lampert, M. (1990). When the problem is not the question and the solution is not the answer: Mathematical knowing and teaching. American educational research journal, 27(1), 29-63.

Spicer, J., & Stratford, J. (2001). Student perceptions of a virtual field trip to replace a real field trip. Journal of Computer Assisted Learning, 17, 345-354.

So You Want to Be a Mathematician: Physical Aptitude Required

My readings for this lesson revealed the following key ideas:

The idea of coupling describes a mutually influential dynamic of interaction between learners and their environments. (Winn)
The real power in augmented reality lies in using digital technology to enable students to see the world around them in new ways and to engage with realistic issues in a student-connected context. (Klopfer & Sheldon)
If physical objects focus a child’s attention on irrelevant aspects of a procedure rather than on the underlying concept, the child may be unable to generalize learning to a new context. (Novack, Congdon, Hemani-Lopez, and Goldin-Meadow)
A meta-analytical study of research articles by Wu, Wu, Chen, Kao, Lin, and Huang found that only 5% of the studied articles investigated the affective domain during mobile learning and only 5% evaluated the influence of learner characteristics in the mobile learning process.

As a math teacher who regularly recommends and models physical manipulative for math learning, I was initially saddened by Novack, Congdon, Hemani-Lopez, and Goldin-Meadow (2014) that action-based learning can actually inhibit students from applying their learning to novel contexts. Their further explanation, however, of the concreteness fading theory was reassuring as it pointed to the way in which I strive to use physical manipulatives. According to this theory, the most effective way to use representations for learning is to first introduce concrete representations then transition learners to more symbolic or abstract representations. Symbolic and abstract representation is where I envision a valuable role for augmented reality and mobile apps for learning. Students can progress from a concrete physical tool to a digitally represented tool, and ideally eventually to an abstract gesture approach that allows them to apply their learning in novel contexts without the limitations of technology availability. One way I envision using embodied learning with my senior math students is using body and arm positioning to represent the shape of particular types of functions, such as the trigonometric functions, a cubic, etc. By using movement to represent these forms, it is my hope that it will help them to apply the abstract rules to the physical position and movement. Following from Novack et al’s findings, having my students orally say certain conditions and rules while performing the gestures will potentially help them better internalize the learning.

Winn (2003) explains that internal rules or procedures that specify how a student interacts with his/her environment change through adaptation primarily based on their success at producing fruitful behaviour. Students working with physical manipulatives such as base-ten blocks will be able to use them fruitfully for particular contexts for a period of time. Eventually, they will reach a point where they are no longer applicable or efficient. Movement to a different method of exploration can thereby return the learning to fruitful levels. Eventually, the development of an abstract concept will likely enable the student to use abstract strategies to produce fruitful behaviour that was not possible with other tools. For my own STEM practice, this reinforces the idea of scaffolding learning experiences to move students from the concrete to the abstract in progressive stages that allow them to also recognize limitations and learning needs for themselves. With the growth of mobile learning opportunities and device proliferation, this process can be further expanded into the home as students are able to engage in representative learning activities on personal devices as well. Wu et al (2012) highlight the conclusion of Ketamo (2003) that while mobile technology can generally bring some added value to network-based learning, it cannot replace conventional computers. As mobile devices continue to advance in their development they offer more possibilities, but there remain tasks that are far better suited to a computer, such as those that require large amounts of memory, processing power, electrical power, or certain forms of tactile interaction such as a full-size keyboard. Thus, I still recognize connected but different roles for both mobile technologies and computer-based technologies as components of the learning process.

Questions Arising:

If educational philosophy is increasingly focusing on student engagement through personal connection and the affective component of holistic development, how can we reconcile a push for these personalized approaches with a seeming lack of sufficient research on the affective and learner-centered influence of mobile learning opportunities?

Winn explains Umwelt as the environment as seen and understood by different individuals. Recognizing that understanding a student’s Umwelt is essential to engaging them in meaningful and fruitful learning opportunities, what are strategies a teacher can use to gain a deeper understanding of a student’s Umwelt in any given situation, particularly when a student is currently lacking in engagement?

Novack et al found that gesture was an important component of grade 3 students learning how to group when adding more than two numbers as they used their hand to gesture the v-formation of combining values. How can gesture be incorporated into the teaching of more complex mathematical processes?

Resources:

Klopfer, E., & Sheldon, J. (2010). Augmenting your own reality: Student authoring of science‐based augmented reality games. New directions for youth development, 2010(128), 85-94.

Novack, M. A., Congdon, E. L., Hemani-Lopez, N., & Goldin-Meadow, S. (2014). From action to abstraction: Using the hands to learn math. Psychological Science, 25(4), 903-910.

Winn, W. (2003). Learning in artificial environments: Embodiment, embeddedness, and dynamic adaptation. Technology, Instruction, Cognition and Learning, 1(1), 87-114.

Wu, W. H., Jim Wu, Y. C., Chen, C. Y., Kao, H. Y., Lin, C. H., & Huang, S. H. (2012). Review of trends from mobile learning studies: A meta-analysis. Computers & Education, 59(2), 817-827.

Enhancement, Not Replacement

	Basic Premise	Similarities	Differences
T-GEM & Chemland	*Cyclical process of Generating, Evaluating, and Modifying hypotheses	Emphasis on inquiry, with student building and creating their own understandings Technological tools to provide simulation opportunities not otherwise available Moves away from more traditional rote instruction and memorization Students work with specific goals in mind *Collaborative opportunities provided and often required for ultimate learning	Digital tool enables exploration of concept to generate hypotheses and synthesizes vast quantities of data Enables students to interact with concepts too small, rare, or dangerous to interact with otherwise in a school context
Anchored Instruction & Jasper	*Placing learning in authentic, rich contexts based on problem-solving		Digital tool provides context for activity Context is not as immediately adaptable to other student interests or needs, but teachers can create their own designs based on the model on their own
SKI & WISE	Connect to personal context of prior knowledge and relevant problems Support learning with scaffolding		Digital tool provides scaffolding opportunities in exploration Ongoing community of practice with expanding resources
LfU & MyWorld	*Integration of concepts with discipline-specific skills and processes		Digital tool compiles data for students and expedites analysis process Enables students to interact with potentially immense land masses and complex patterns in a scale representation

I find these four foundational technology enhanced learning environments and approaches to be similar in their core principles, but subtly different in their specific application and implementation. At the root of these TELEs is an emphasis on student-directed learning through inquiry and skill development. This is a movement away from a teacher-directed model of learning in which the students are the passive receivers of information. Through these models, the students are the creators and discoverers of knowledge, while the teacher steps into the role of the guide, supporter, and facilitator. This enables more personalized and individualized learning experiences. For example, students can create their own personal hypotheses through a T-Gem activity rather than being told what they should be looking for. They have the opportunity to test their own theories, which would also be similar to the LfU principle that students should use discipline-specific processes when working with concepts. The value of community is also a common thread, as students learn from their interactions with others online or face-to-face, and educators can connect as well through databases of projects and ideas. The ultimate goal is for students to engage in authentic and meaningful learning experiences that foster understanding, growth, and further learning.

The role of the technological tool itself can differ somewhat between the approaches. For example,in the Jasper video series, the videos are not customizable and provide the context for the problem solving. The story-based design engages interest and sets up the need for new learning, but the manipulation and experimentation occurs outside of the tool. In Chemland, the technology allows students to visualize and manipulate concepts that would not otherwise be observable in a classroom environment, but the goal of the technology use is to develop theories and experiment with them. Chemland is both the context and the exploration area for the learning.

Working with technology enhanced learning environments in this module has expanded my understanding of the options that are available to students and to teachers. My approach to learning through guided and independent inquiry and student-led learning was validated by the goals and approaches of the theories and programs we explored. These models, however, have provided me with more specific frameworks in which to design and situate learning experiences. I have also been able to envision new technology tools I can use in my senior mathematics classroom, as well as new ways I can apply the technologies I already use with my students. For example, when working with statistics, the authentic contexts provided by the scientific modeling programs can provide valuable and real experiences for my students to develop a better understanding of the actual meaning of what they are doing.

An important overall takeaway for teachers integrating technology is that while technology enhances learning experiences and environments in each of these approaches, it does not replace the personal relationships of learning. TELE is not about putting a student in front of a screen and walking away, but rather, it is about leveraging technology to provide students with better learning experiences that support their learning needs, while also engaging students in collaborative discourse. While the role of the teacher may change, it does not become diminished.

Lines, Curves, and Equations – Oh My! [Desmos and Equation Development Using T-GEM]

Initially, I found it challenging to apply T-GEM to mathematics as I found it hard to picture, but after completing this activity, it seems to be very similar to many of the activities I already strive to do with my students. A challenging concept for my students in secondary math is creating and understanding equations for lines and quadratic polynomials using graphs or scenario details. This challenge has been identified through concept pre-assessments, the nature of their questions throughout their work, and continued struggles on summative assessments. They struggle to make the connection between the information they are given and the algebraic, abstract representation.

Generating: For the generating phase, I would have students brainstorm what they already think they know about equations with regards to what they mean and how they can be used. Students will randomly select equations from a centralized equation bank to explore using Desmos (available either online or as an app). Working in pairs, students will develop a set of rules for creating equations based on their explorations of manipulating equations within Desmos.

Evaluating: Once students have developed their guidelines, pairs of pairs will be joined into groups of 4, in which students will compare their initial hypotheses and justify their perspectives. Ideally, reflection would occur as students need to explain how they arrived at particular conclusions. At this point, scenario-based problems will be introduced to the original pairs, expanding upon the initial work with base equations. Students now need to determine if the rules they established in their initial phases apply appropriately to their new scenarios. If they are not able to use their rules to accurately create an equation to represent the scenario and use it to solve problems, they will need to identify the gaps and determine what adjustments need to be made. Desmos will continue to be the technology tool at this level, as students are able to easily test, adjust, and visualize their inputs.

Modifying: In their pairs, students will reevaluate their list of rules for equations, taking into consideration their initial hypotheses, their discussions with peers, and their testing of their hypotheses. As a class, we would come back together for a large class discussion to compile their ideas into a community-based understanding.

This process could be further expanded to include different types of polynomials. For example, students initially working with equations of lines could then generate, evaluate, and modify new hypotheses regarding quadratics, based on their work with linear relationships. Subsequently, work with quadratics could be further cycled to work with cubics, then quartics and other higher order polynomials, as appropriate.

By having students use Desmos to work with the different parameters of the equations, they are able to actually experiment to see the effects of changes, rather than simply being told to memorize, for example, that the c value of a quadratic equation determines the vertical position of the graph but doesn’t directly affect its shape when a and b stay constant.

I believe that many mathematical principles and concepts can be approached using similar strategies to those used for scientific principles and concepts, and aim to include them when possible in my teaching. One challenge I have in senior math is the perception of teachers regarding the comparable value of experimentation and hands-on math in the university-bound courses as compared to the middle school or college/workplace-bound courses. I am often met with resistance from colleagues who don’t believe there is a place for experimental or hands-on learning in the higher-level university-bound courses, and that such activities are frivolous at that level. Do you feel that there is value in hands-on math learning for the senior level university-bound math students?

Sources Consulted:

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905

Khan, S. (2010). New pedagogies for teaching with computer simulations. Journal of Science Education and Technology, 20(3), 215-232.

Channeling My Inner Miss Frizzle – in Math

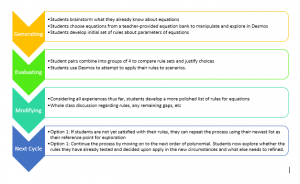
In what ways would you teach an LfU-based activity to explore a concept in math or science? Draw on LfU and My World scholarship to support your pedagogical directions. Given its social and cognitive affordances, extend discussion by describing how the activity and roles of the teacher and students are aligned with LfU principles.

My school division uses the Math Makes Sense line of mathematics textbooks and programming as the main resource for math education up to Grade 9. I, and most teachers I know of, have a love-hate relationship with the approach of MMS, as it tends to be very abstract and conceptual without always including as many hands-on, nitty-gritty experiences for the students. While each lesson begins with an exploration task, these explorations are often too difficult for students when they lack a meaningful context or are really just pencil-and-paper tasks. After exploring the readings and activities this week, I have somewhat softened my perspective of MMS, however, in the sense that I believe the general approach of the programming aligns to a certain extent with LfU principles, although the execution may not always follow suit. This can be accounted for with supplemental and substitutional experiences designed by the instructor. I like to use hands-on exploration activities with my students, but I often situate them after I have provided initial instruction. LfU would dictate that students begin with rich exploration tasks, and then the teacher supports consolidation of learning afterwards.

One big take-away I gained from this week’s reading was in Perkins, Hazelton, Erickson, and Allan’s article regarding place-based education. They explained that, “Introducing GIS and GPS in the students’ familiar and immediate surroundings more easily bridges the gap between the real and digital worlds. Each student has tangible experience with their schoolyard and, therefore, some sense of that space that will allow them to construct new knowledge in the context of a place that they know” (2010, p. 217).When working with measurement in math, and specifically with unit conversions in early high school, LfU-based activities can involve students exploring the actual space of the classroom, school, and school yard to look for patterns in relationships between measurements taken using different measurement devices. Providing students with specific tools that can provide or not provide specific measurements can create a need for strategies to use the tools at hand to accomplish the task. Following an investigation of such measurements, discussion regarding patterns and trends could follow, with students also having an opportunity to ask peers questions regarding incomplete connections or misunderstandings. The teacher can help to build a common record of findings and patterns, working towards conversion rules. This investigation could be followed up with an application to a space of their choice – the rink, a baseball diamond, a theatre, with students needing to determine certain measurements in order to refurbish the space with the appropriate materials. Students are the drivers of the conceptual and skill development, with teachers taking on the role of guide and supporter.

A second concept that I feel is very important is that “The designer or teacher must also pay attention to the preparedness of the learner to receive the information and the processing and use of the information that the student will be asked to do in the learning context” (Edelson, 2001, p. 377). Teachers need to meet students where they are at, not where we think they should be. If a task offers too much challenge for a student, s/he will likely not find the motivation necessary for LfU, or may struggle with the tools themselves. As teachers, we can support students in LfU-type activities by ensuring that the learning activities and tools are equitably accessible to all students. Students who need additional supports to participate in the investigation can still explore and create their own learning, and will benefit greatly from doing so. For example, a student with weak short-term memory skills, may need a written list of steps for the process of a particular activity, but these steps can be written by the student with the assistance of a teacher or educational assistant so that they are not directive, but rather supportive of the learning exploration process. The same way that some students need glasses to see, we need to remember that some students need specialized supports or adaptations in order to be able to properly access and participate in the learning. Such supports could include strategic grouping or pairing, outlined step lists, exemplars, scribing, audio support, etc. Students with academic challenges deserve to participate in exploratory activities as much as students who do not require additional supports.

Ultimately, the teacher’s job is to provide the context for learning experiences that stimulate motivation and curiosity, support students in their problem solving skill development, gently guide students in a better direction when they get off course, and explore with the students. When students see teachers learning with them, it creates less of a perception of teachers as the keepers of all knowledge. Ths also reinforces the LfU idea that “the construction of understanding is a continuous, iterative, often cyclical process that consists of gradual advances, sudden breakthroughs, and backward slides” (Edelson, 2001, p. 377). Teachers as learners reinforces the concept of ongoing learning.

Students need to be given agency to explore and “get messy” with their learning. There are many interesting and open-ended tasks for learning in mathematics if teachers are willing to provide these opportunities for their students. In the words of one of my favourite television teachers, Miss Frizzle, teachers and students engaging in LfU-styled learning need to be willing and prepared to ‘Take chances, make mistakes, get messy!’

References:

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching,38(3), 355-385. http://ezproxy.library.ubc.ca/login?url=http://dx.doi.org/ 10.1002/1098-2736(200103)38:3<355::aid-tea1010>3.0.CO;2-M

Perkins, N., Hazelton, E., Erickson, J., & Allan, W. (2010). Place-based education and geographic information systems: Enhancing the spatial awareness of middle school students in Maine. Journal of Geography, 109(5), 213-218. http://ezproxy.library.ubc.ca/login?url=http://dx.doi.org.ezproxy.library.ubc.ca/10.1080/00221341.2010.501457

Is It Getting Hot in Here?

I explored the project titled “What Impacts Global Climate Change”, as while I do not teach science, I identified a potential connection to social studies as well. This project is an excellent opportunity for interdisciplinary exploration. While the majority of the project appears to be effective as is, I introduced the Maldives as a case study example of the potential consequences of rising sea levels. Ideally, students would be able to connect with the issue of global warming and rising sea levels through either the plight of the Bengal Tigers or the threat to humans. Most students should be able to make a connection to how their own lives would be impacted if water was encroaching on their living space. I also added a brainstorm question at the beginning of the fourth activity that asks the students to suggest ways they and their families can reduce their carbon footprint. After working through the remainder of activity four and activity five, students are asked the same question again. Student responses in both instances are entered anonymously in an effort to encourage students to be open and honest, and to build a collective set of options for the class. Additionally, students must submit their own response before being able to see their peers’ responses, so as to appeal to their actual personal thoughts, and not simply what they feel like everyone else is saying or expects.

Using this WISE project with my grade 8/9 class, I would begin with a graffiti style brainstorm in which students are presented with blank pages titled “global warming”, “greenhouse gas”, and “human impact” spread throughout the classroom. Students have a certain amount of time at each station to add any of their initial ideas to the brainstorming sheets. These initial brainstorms will help us organize what we already know, what we think we know, and what we want to find out. Students will then begin to work through the WISE modules at their own pace. This allows students to move more quickly or take more time as needed to further their personal understanding. At the end of each class, students will be given a sticky note on which to write a question about the concepts that they still want to learn more about. We will compile our ongoing questions on a class “I Wonder” board. These questions can be basic knowledge questions or deeper conceptual connection questions. We will take time as a class to revisit our questions on Wednesdays and Friday to see which questions students can provide answers or elaboration to and which questions require further inquiry.

The weaving of varied feedback opportunities throughout both the online activity sequence and the oral discussions should work towards what Hattie and Temperley identify as the purpose of feedback – the reduction of discrepancies between current understandings and performance and a desired goal. The ongoing question board is one meaning of helping students to set goals and purpose for their inquiry. Since the questions are student-generated, they should also be connected to the interests of the students themselves, hopefully encouraging them to embrace the inquiry process both inside and outside of the classroom. As students work through their self-paced work, I am available to check in with students in sustained interactions to gauge their understanding and help address misconceptions. SKI principles are addressed through students needing to be able to rationalize their choices and explain their thinking process, addressing prerequisite knowledge gaps in small group and one-on-one support sessions, encouraging students to share their learning with one another to approach the class-generated questions, and striving to support the personal learning interests of the students. Ideally, I would like to follow up the WISE project with students applying their learning in the development of a personal action plan or community project to promote positive citizenship and real-life application of learning.

Hattie, H. & Timperly, H. (2007). The power of feedback. Review of Educational Research, 77(1), 81-112.

Linn, M., Clark, D., & Slotta, J. (2003). Wise design for knowledge integration. Science Education, 87(4), 517-538

Williams, M. Linn, M.C. Ammon, P. & Gearhart, M. (2004). Learning to teach inquiry science in a technology-based environment: A case study. Journal of Science Education and Technology, 13(2), 189-206

Disengagement and Disconnection: Anchored Instruction as Active Involvement

The Jasper materials appear to be responding to issues of disengagement and disconnection. When student are not interested in their learning, they become disengaged, and disengagement can lead to reduced effort, reduced learning, and increased classroom management problems. When there is a perceived or actual disconnection between learning situations and the real world, between concepts, and between students and peers, it can be difficult for students to truly learn and understand a concept. Even the most well-intentioned teacher can miss the mark in creating “authentic” example scenarios for students. I agree with both of these problems. If educators want students to crave learning, students need to be able to recognize value and themselves in their learning experiences. While the Jasper materials are not a one-size-fits-all magic solution, they can definitely be a valuable component of a student-centered classroom and learning design.
The primary way in which the Jasper materials address these issues is through giving students an authentic purpose to their learning. The goal is not simply to complete a certain number of questions, memorize a particular formula, or use the right word at the right time. Instead, the goal with the Jasper materials is for students to connect with the story and decide on how it will be resolved. Students are in control of the path and the methods used, which allows them to find confidence in their own thinking processes, regardless of how they get to the destination. In their description of the application of anchored learning to high school statistics, Prado and Gravoso explain how although three student groups were not able to arrive at a correct answer, all groups applied the correct formula and values; the error was in computation. Anchored instruction tells students that their process is valuable, just as it is in a non-school situation. Even those groups who made a minor computational error likely showed increased problem solving skills and interest in statistics. Releasing some of the structure of the solution process can be challenging for teachers who are inclined to see the “correct” solution in a particular way. A pedagogical shift, however, to allow students to direct the process enables more engagement and more connection, both for the students and the teacher.
Contemporary videos available for math instruction seem to rest somewhere between a traditional classroom model of instruction and anchored instruction. While videos are becoming more and more interactive and open-ended, such as in embedded quizzes in some Khan Academy videos, the primary goal of most of these videos seems to be basic instruction. Although a video affords the viewer the ability to pause, fast forward, rewind, and access the instruction from any location at any time, ultimately, watching many of these videos is still a passive process. While these videos offer many classroom benefits such as being able to work with split grades, support students with different learning needs, help students stay caught up from home, and supporting a teacher when he/she is not confident in the material, the Jasper materials go further into collaboration and higher order thinking. The other videos can help ignite some interest among students and depending on the context, can help students better understand broad connections, but they are not nearly as effective as an experience that allows them to direct the process, that frames a narrative (kids like stories!), and that keeps them wondering and wanting to learn more.

Resources:

Cognition and Technology Group at Vanderbilt (1992a). The Jasper experiment: An exploration of issues in learning and instructional design. Educational Technology, Research and Development, 40(1), 65-80.

Prado, M. M., & Gravoso, R. S. (2011). Improving high school students’ statistical reasoning skills: A case of applying anchored instruction. Asia-Pacific Education Researcher (De La Salle University Manila), 20(1).

Shyu, H. Y. C. (2000). Using video‐based anchored instruction to enhance learning: Taiwan’s experience. British Journal of Educational Technology, 31(1), 57-69.