If the Wicked Witch of the West co-authored this week’s reading, it may have been subtitled,
“U’m- Welting!”
I always know when I am enjoying a week more than others, based on the amount of effort I put into the reading and note taking. And without any sarcastic undertones, I can honestly say that this week was a huge time suck. Perhaps it is the “science-geek” in me that really favors learning about theories that are neurologically situated (I’m not a neurologist, and I don’t even play one on TV, but neurological research unquestionably fascinates me). Perhaps it is that I am a self-proclaimed Queen of Analogies. All I know is that this week really blew my hair back! Floated my boat! I really picked up what the authors were laying down! Hopefully, you are reading my mail, here. (OK… I think I’m done now.)
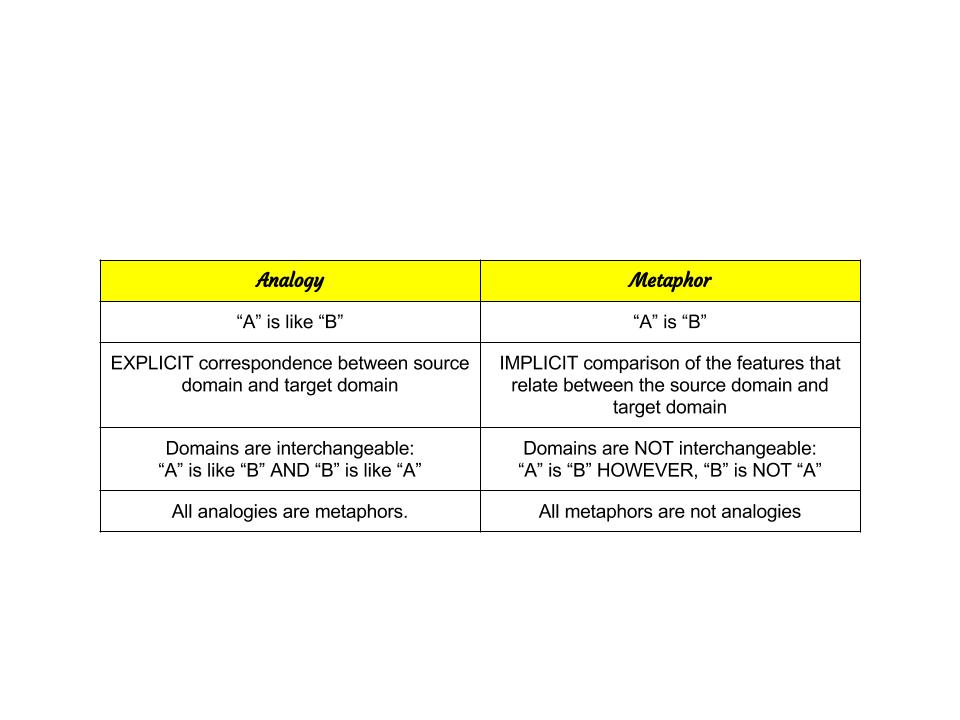
If you did not read, “Understanding Needs Embodiment: A Theory-Guided Reanalysis of the Role of Metaphors and Analogies in Understanding Science” (Neibert, Marsch, & Treagust, 2012), I highly recommend that you save the PDF for recreational reading at a later time. Although you may not profess to be the King, Queen, and/or Joker of Analogies in your classroom, there is no possible way that one can avoid using analogies/metaphors (and yes, there is a difference) within one’s day-to-day speech. The authors provide a simple example such as “I see your point” as a metaphorical representation of understanding and vision. As a teacher in a school with 20% of the population being in our International Program, I am very careful to explain some of our “weird” Canadian sayings— just this week I was explaining the analogy “Six of one and half dozen of the other.”
So what makes a great analogy/metaphor (a/m) versus an ineffective analogy/metaphor?
- Your a/m should utilize everyday embodied sources that ALSO can be imaginable—it is ineffective to use sources for our a/m that a student hasn’t any personal experience with and/or can not relate to.
- The learning goal (target domain) should involve a first or second-hand direct learning experience—have students actually touch things!
- Models can serve as an embodied source domain that enables reexperience and reflection opportunities surrounding abstract concepts.
- Recognize the limitations of the a/m: they may bring to light (“highlight”) the key ideas yet simultaneously misinform (“hide”) other related concepts.
Questions to chew on:
- What is your favorite analogy or metaphor to use in a science or math context? How do you know that it is an effective analogy? (It’s OK if you don’t!)
- Have you ever had an analogy or metaphor “backfire” on you?
Looks like I’m past my word count… time to make like a baby and head out!
P.S. In case you were curious…
References
Niebert, K., Marsch, S., & Treagust, D. F. (2012). Understanding needs embodiment: A theory‐guided reanalysis of the role of metaphors and analogies in understanding science. Science Education, 96(5), 849-877. http://ezproxy.library.ubc.ca/login?url=http://dx.doi.org/ 10.1002/sce.21026
Winn, W. (2003). Learning in artificial environments: Embodiment, embeddedness, and dynamic adaptation. Technology, Instruction, Cognition and Learning, 1(1), 87-114. Full-text document retrieved on January 17, 2013, from: http://www.hitl.washington.edu/people/tfurness/courses/inde543/READINGS-03/WINN/winnpaper2.pdf
It may be “bad form” to respond to my own question, but what the heck. One metaphor that I really like to use in Math is likening a raw egg to a number. The number pi is a great example, (Happy Pi Day, by the way!)— I would guess that most teachers have students initially use the approximate value of 3.14 in their calculations. Starting in at least Math 10, however, we insist on using the entire number, or the exact value, in our calculations, so we make sure that students use their pi-button. The egg comes into play when I ask the students if I could pass them an egg— if they want the entire egg, I should probably keep the egg intact (use the pi-button) but if most of the egg is sufficient, I could crack the egg open and scoop it up with my hands and pass them the gooey mess. Some of the egg would be left on the table, but in many cases, what I have dripping in my hands is ample to get the job done. It is a neat way to visualize why 3.14 is approximately but not exactly equivalent to pi. Since everyone has experience with cracking eggs, presumably, this metaphor should be very easily embodied into the students’ prior experience. (I have a math toilet metaphor for inputs, outputs and functions… I will just let you imagine that one, though.)
Hi Dana,
This is not necessarily an analogy but I have found this to be very effective in helping students understand the solar system and the way planets move with in it. During this unit, students create a “to scale” version of our solar system featuring the planets and sun. For the secondary part of this groups are asked to dramatize the solar system (it’s action and interactions). For the most part, it is considered successful if students have different planetary bodies rotating around the sun (they are not expected to show overlapping orbits), kids get creative and dress as their planet etc. Students have a lot of fun with this activity although some get pretty dizzy when they take the spinning too far. On occasion, groups have astounded me (this is grade 6) with planetary orbits taped out on the floor, spinning speeds in relation to one another and the actual speed the planet rotates at. Costumes that represent the size of the planet again to scale with other planets (with in reason). Every year students say this was the first time they understood how it all worked.
Catherine
Hi Catherine, How awesome would that be? I would have really dug into this as a student, for sure. Your idea really reinforces this week’s readings regarding the physical nature of embodiment, as well! I don’t know if you get into the conversation about why/how the planets orbit the sun, but this is a neat visualization that I use in my Physics 11 class: https://www.youtube.com/watch?v=MTY1Kje0yLg Thanks for sharing! Dana 🙂
Hi Dana,
One analogy I have found effective is the use of temperature/thermometers to teach students integers. I am lucky (sometimes I question this…) to live in a climate where the temperature varies from up to +30 degrees Celsius in the summer, to down to -30 degrees Celsius in the winter. Generally this strategy works quite well because students have a concept of temperature already as it is a lived experience for them every year – every summer the temperature goes up, and every winter the temperature goes down. It is also made easier by the fact that we have thermometers for the students to use at the school (so they have the opportunity for hands-on learning) and virtual thermometers are also available online if I wanted to incorporate technology or an online graphing application into the equation. The area where this falls short is when students must subtract a negative. This concept continues to be a struggle, although a couple of posts back, Gloria discussed a strategy she uses where students take away/subtract unhappy/negative feelings which then makes them happier. I thought this was a really neat analogy that I would like to try in the future!
Another analogy I have always wanted to try, but have not yet had the opportunity to try is the pizza fractions math. I have a colleague who bakes pizzas with her students and then they do fractions with their pizzas before they eat them. I always thought this was such a neat idea. It would also be engaging for the students, although I’m not sure how focused on the math they would be once the pizzas came out. Reading Catherine’s response this week, titled “3D Geometry with Leap Motion: A lesson in interpretive Dance” I was interested to learn that this pizza idea may not actually support the learning of fractions to the extent that I had envisioned it would. Catherine shared, “I was fascinated by Pouw et al. (2014) article on the use of manipulatives with children in math and science and how the type of manipulative affected learning. Students who used symbolic representations of an item (for example pie pieces to learn fractions) were less able to transfer that knowledge to other scenarios while transfer of learning was higher for students who learned with arbitrary symbolic representations such as blocks (p. 64).” I did not read this article, but it looks like I should in order to gather more information before I start buying ingredients for a lesson that may not allow for the transfer of knowledge to other situations.
Hi Mary, Thank you for sharing! There are definitely a few advantages to living in “extreme” weather regions— I never thought that math would be one of them. 🙂 In light of Catherine’s research, my thoughts with the pizza would be to use it as a “reinforcer” as opposed to a “teacher”. It would be the same pedagogical reasoning that I use when I give a Kahoot in class. It may not be the most educational, but it is at least related, and it is a hoot to do! ~Dana
Thank you for continuing to make these posts amusing and fun to read. I, too, got lost in the readings and finding more and more information that I wanted to know about these topics. Although I can acknowledge your place as the Queen of Analogies, I use them most frequently in my class as well. (Funny though as soon as you ask for one I had a hard time thinking of all the things I say on a day to day basis) However, I find that the one I have been using most often this year refers to the level of work that students have been turning in for assessment. I am not sure if they are lazy, don’t care, or never learned what exemplary work looks like, but whatever they are doing it is not it. I found myself referring to going to MacDonald’s and ordering a Big Mac. I asked them how it would be if they ordered the hamburger but was only given part of it, say the bun and the meat, but were missing the rest. How would they feel? Most said they would complain and either ask for another one or get their money back. Bingo! I said exactly, most of you are giving me just the bare minimum of the bun and the meat, but no cheese, lettuce, or special sauce. One student caught on right away and said, some of us are only giving you the bun. I then put up the hamburger rubric to demonstrate exactly what I meant and what level the bun and lettuce was compared to the whole hamburger or even a deluxe. I can now confidently say to them “This is not the whole hamburger!” and they get it.
Anne
Hi Dana,
I am enjoying reading about the various uses of analogies in your classrooms, also in part, because some of my graduate students’ research has focused on their use:
Khan, S. & Chan, V. (2011). An exploration of digital representations in chemistry education. Journal of the
Research Center for Educational Technology, 7(2), 2-38.
Trey, L., & Khan, S. (2008). How science students can learn about unobservable phenomena using computer-based
analogies. Computers & Education, 51(2), 519-529.
If you do have time, however, I would first recommend checking out the interesting work on the “bridging analogy” by John Clement.
Kind regards, Samia
I couldn’t help but giggle many times throughout your post, Dana, and then afterwards as I began to think about analogies that flopped. Our school literally stares at the bottom of our local ski hill, and we are very lucky to be able to take the whole school a few times for ski days. Typically I have lucked out with my grade 3 students and get to ski with them for the day but this year I ended up on Bunny Hill duty. Picture this: packed bunny hill, kids (kindergarten-grade 3) shakily trying out skiing and snowboarding for the first time, adults spread all over the bunny hill lifting kids off the “magic carpet” ramp, and then the odd kid here and there just ripping straight down the hill bullet straight and all these adults turning and yelling PIZZA!!! PIZZAAAAAAAAA! And I got to thinking…yes, to an adult it makes sense that the traditional plough stop when learning skiing may look sort of like a slice of pizza, but as this eyes wide shaky child begins to go faster and faster down the hill, I am not sure that this analogy is as helpful as we may think it is. Thanks for an amusing post that helped me relate more to the readings this week!
Allison