ETEC 533 – Technology in the Mathematics and Science Classroom
Working through the e-folio process has been quite insightful. I actually ended up laughing, with a bit of head scratching, at some of my earlier writings from the beginning of the course. 🙂
Here is the link for anyone interested, as well as an alternate way for Samia to access the blog.
Cheers, everyone! It’s been a pleasure!
https://blogs.ubc.ca/jessicaholderetec533/
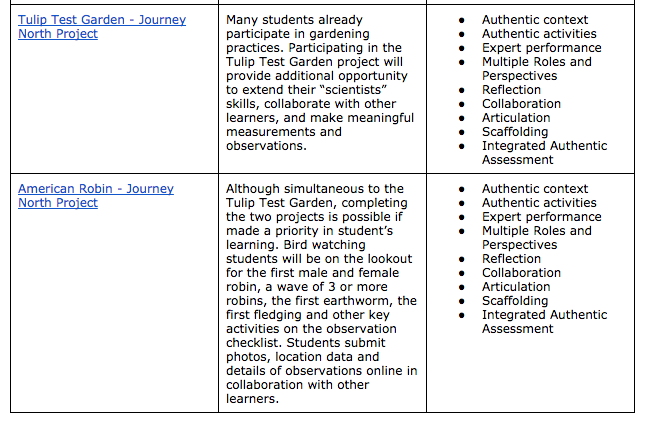
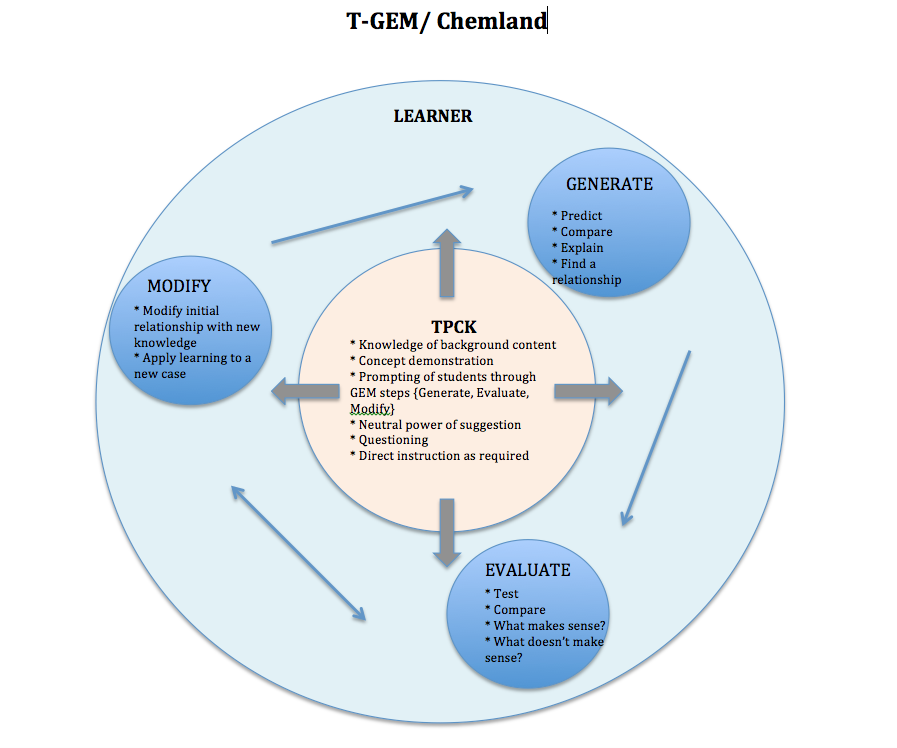
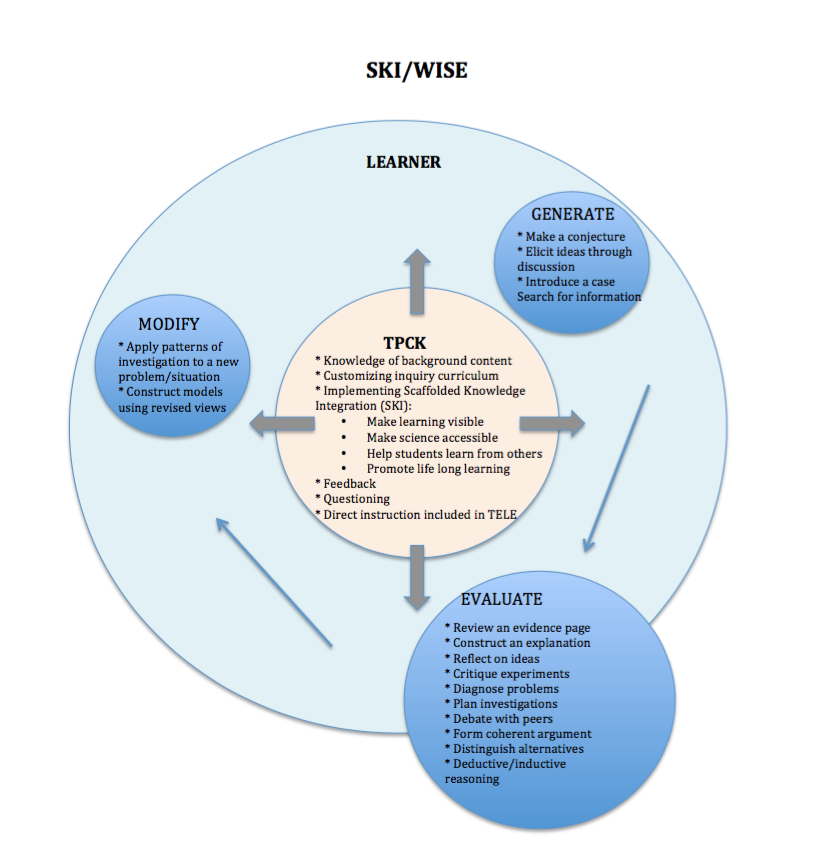
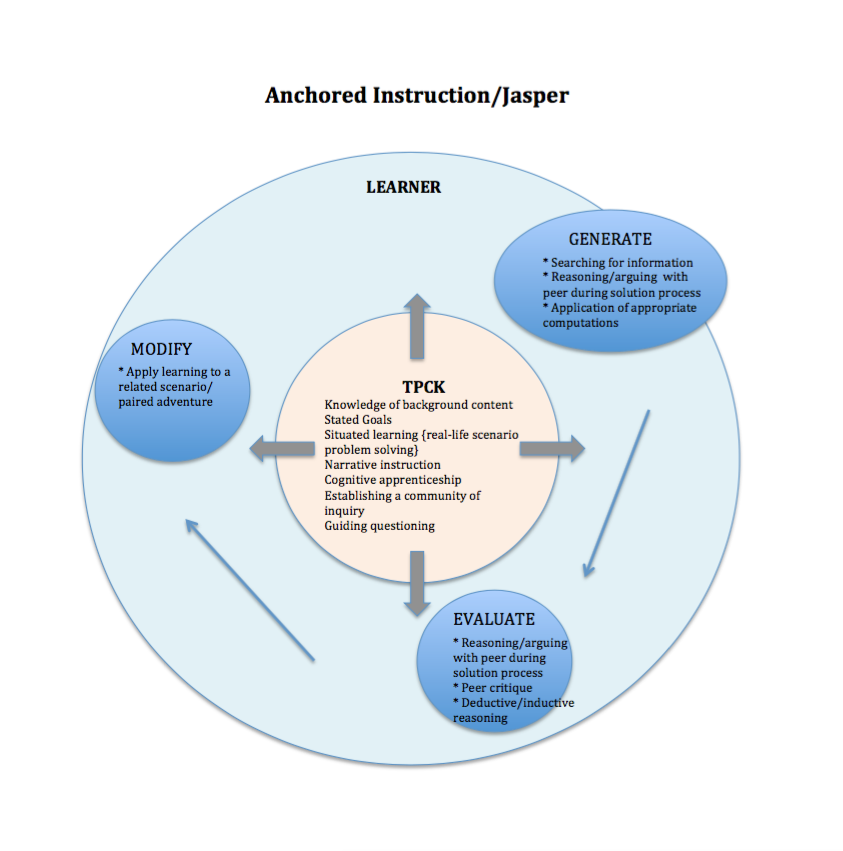
Throughout this MET533 course, the instructor, Dr. Samia Khan, has diligently commented on student blog postings by affirming student thinking, offering further guidance, and posing questions for deeper inquiry. Several of Dr. Khan’s comments to my own posts were intentionally written, I believe, to spur me on to more thoroughly consider model-based learning and its purposes. Using prior knowledge and previous perceptions I attempted to ponder the use of models within instruction and teaching, yet it was not until the T-GEM lesson during Module B that I began to see more clearly the direction which Dr. Khan was gently pointing.
For interest’s sake, samples from Dr. Khan’s comment prompts on model-based instruction and learning are posted below:
From “Conceptualizing Misconceptions”:
Your careful comparison of the role of visual representations in fostering partial or incorrect conceptions leads one to wonder in what ways can children’s drawings contribute to understanding in math and science and our assessment of what they know?
From “Plate Tectonics: Reshaping the Ground Below Us”
I wanted to focus in on the process of modeling for this post, which lends itself nicely to the area of plate tectonics. Gobert et al. in their paper that students can engage in model-based reasoning with models, be they dynamic, runnable visual models in WISE or ones created from physical materials such as that of the plates or the Earth. For example, one of the first activities is for students to draw a model of how mountains are formed and then explain within WISE what happens to each of the layers when a mountain is formed. Students then critique peer models using prompts in WISE such as, what do you think should be added to this model that would make it better for someone who does not know geology. Peers then revised their models by examining and considering these recommendations.
The Geology Book is currently being used to support the construction of models, and I was wondering in what ways some of these model construction, reconsideration processes be fostered in some of the activities that you already have (eg. with WISE) or with hands on-materials?
From “Staying Afloat: Sink and Float Density T-GEM”
In the modification phase, asking students to design a pictorial representation (model) of the data is one way to begin to inspect their conceptual understanding. it will be interesting to see how students represent heaver objects in these pictorial models.
By investigating varied information visualizations, specifically NetLogo, further understanding of model-based instruction and learning has developed. NetLogo contains similar features to some of the instructional frameworks studied in Module B i.e. WISE/ SKI and T-GEM. These similarities offer rich inquiry opportunities for learners and include: being overseen by experts, allowing for teacher collaboration and authoring, offering exploration of micro and macro phenomena, and student modification of models to observe patterns and anomalies. Other affordances evident in NetLogo are similar to PhET as described in the study conducted by Finkelstein, Adams, Keller, Kohl, Perkins, Podolefsky and Reid (2005). Key characteristics of inquiry simulations include an emphasis on discovery rather than verification and allow for the exploration of microscopic behaviors or patterns that are not physically observable in real-life scenarios. As described by Finkelstein et al. (2005), “[a] variety of visual cues in … computer simulations make concepts visible that are otherwise invisible to students” (p.6). Students are able to make meaning of observable global activity by viewing localized patterns. Resnick and Wilensky (1998) refer to this type of modelling as an exploratory model “start[ing] with rules for the individual parts of a system, and … observ[ing] the group-wide patterns that arise from the interactions” (p. 162). Another feature described by Finkelstein et al. (2005) is the limited nature of simulations when each simulation is designed around a focused topic. This aspect of limitation is evident in NetLogo as specific models are available across a breadth of domains, including sciences {biology, physics, chemistry, social,} mathematics, computer science, and art. Interestingly, rather than inhibiting learning, the limitations tend to result in enhancing learning by minimizing distractions caused by excessive choices. Finkelstein et al. (2005) describe this enhanced productivity in the following way:
[B]ecause the system under investigation is constrained in particular ways, students are able to make progress they cannot in an unconstrained environment… Simulations provide the instructor considerably more freedom in designing and applying constraints to ensure that students’ messing about leads to productive learning. Constraints are also valuable as students mimic real scientists and mathematicians by isolating individual variables. This isolation of variables supports student understanding “by focusing attention to relevant details… [that can be] effectively applied to physical “real world” applications. (p.7)
As a distance learning teacher working with elementary students within a range of grade levels, I have concluded that NetLogo simulations are a better fit for upper level elementary learning (i.e. grades 4-7). Browsing through the model library does takes time, yet even within my own limited exploration several models were found that could effectively be incorporated into student elementary programs i.e. Biology/Autumn (Wilensky, 2005), Biology/Sunflower (Wilensky, 2003) and Mathematics/Color Fractions (Wilensky, 2005). This latter model is quite interesting as students decide upon and view a connection between fractions, decimals and visual box patterns. This model is simple enough for a grade 4 student to modify when beginning to learn how to correlate fractions and decimals through curriculum. Each NetLogo model contains a “Model Info” section which is an invaluable feature providing teachers and students with an explanation of the model, what to pay attention to when modifying, ideas for modification, and extension ideas. This “Model Info” is an asset for successful understanding and implementation.
In conclusion, NetLogo is one example of a simulation exemplifying theoretical research through a model-based learning experience. Students are provided the opportunity to explore and discover patterns determined by their choice of modifications and scaffolding is provided within the “Model Info” section to help direct students through guided inquiry. It is necessary for both teachers and students to understand the need for invested time to become familiar with the limited variables, as this is essential in building connections. Viewing phenomena in a new and meaningful way is highly probable through NetLogo and this affordance is something, that I believe, Dr. Khan’s guided inquiry was helping lead me to see.
Finkelstein, N.D., Perkins, K.K., Adams, W., Kohl, P., Podolefsky, N., & Reid, S. (2005). When learning about the real world is better done virtually: A study of substituting computer simulations for laboratory equipment. Physics Education Research,1(1), 1-8.
Holder, J. (January 12, 2017). Conceptualizing misconceptions [Web log message]. Retrieved from https://blogs.ubc.ca/stem2017/2017/01/12/conceptualizing-misconceptions/
Holder J. (February 20, 2017). Plate tectonics: Reshaping the ground below us [Web log message]. Retrieved from https://blogs.ubc.ca/stem2017/2017/02/20/plate-tectonics-reshaping-the-ground-below-us/
Holder, J. (March 3, 2017). Staying afloat: Sink and float density t-gem [Web log message]. Retrieved from https://blogs.ubc.ca/stem2017/2017/03/03/staying-afloat-sink-and-float-density-t-gem/
Resnick, M. & Wilensky, U. (1998). Diving into complexity: Developing probabilistic decentralized thinking through role-playing activities, Journal of the Learning Sciences, 7(2), 153-172. DOI: 10.1207/s15327809jls0702_1
Wilensky, U. (2005). NetLogo Autumn model. http://ccl.northwestern.edu/netlogo/models/Autumn. Center for Connected Learning and Computer-Based Modeling, Northwestern University, Evanston, IL.
Wilensky, U. (2005). NetLogo Color Fractions model. http://ccl.northwestern.edu/netlogo/models/ColorFractions. Center for Connected Learning and Computer-Based Modeling, Northwestern University, Evanston, IL.
Wilensky, U. (2003). NetLogo Sunflower model. http://ccl.northwestern.edu/netlogo/models/Sunflower. Center for Connected Learning and Computer-Based Modeling, Northwestern University, Evanston, IL.
Background:
Financial literacy is highlighted throughout the elementary grade levels in the Content area of BC’s New Curriculum. Most paper-pencil curricula address money identification, counting coin and dollar amounts, and one or two step word problems connected to money. However, these paper-pencil activities minimally equip students for financial literacy skills and applications. While exploring the information visualization simulators during this past week, the elementary and middle school simulations from Illuminations were easy to understand and seemed quite plausible to implement into already developed curriculum.
Literature Support for Lesson Cornerstones:
In a study conducted by Srinivasan, Pérez, Palmer, Brooks, Wilson and Fowler (2006), engineering freshman students who completed learning using MATLAB did not experience what they perceive as an authentic experience. The students felt that their experience was disconnected from real expert experience because they manipulated a simulated system rather than a real-life system. The researchers conclude that a probable reason for this disconnect is that the students “need/want authenticity to be able to make connections the experts make with the simulation” (Srinivasan, 2006, p.140). This perception from the students leads educators to consider the value of real-life experiences in connection with simulated experiences.
Transferring simulated experiences to real-life experiences is supported through the study completed by Finkelstein, Adams, Keller, Kohl, Perkins, Podolefsky and Reid (2005). In their study, students in a second semester introductory physics course, who had used a simulation first to design a circuit system, were more successful later in designing real-life models. These same students also achieved greater success on related exam material that was completed two months after the simulated and real-life circuit building experience (Finkelstein, 2005). Due to these findings, authenticity of learning through the transferring of knowledge from simulation to real-life experience is a main cornerstone of the following lesson design.
In addition to authenticity, two lesser cornerstones, rich content and goal challenge motivation, are also incorporated into the lesson design as supported through the writings of Srinivasan et al. (2006). A pre-test assessment begins the lesson in order to determine prior knowledge and the optimal area of learning for the individual student. As well, this pre-test assessment can be used to determine pairings/groupings throughout the lesson activities. By providing rich content within the lesson plan, this affords opportunity for students with less prior knowledge to acquire new knowledge before exploring the simulated and real-life experiences. Building prior knowledge within students is critical for their success as Srinivasan et al. (2006) state, “Prior knowledge accounts for the largest amount of variance when predicting the likelihood of success with learning new material” (p.138). In regards to gaining knowledge of the student’s optimal area of learning, this connects closely to Vygotsky’s zone of proximal development, but is also supported by goal oriented motivation when learning goals are neither too steep, nor too simple: “If learning goals are too steep for a learner’s current context, learning is not successful. On the other hand, when learning is simple for the learner, the instruction can become over-designed and lead to diminished performance” (Srinivasan, 2006, p. 139).
Lesson Overview:
The following lesson incorporates the instructional framework of anchored instruction. This has been accomplished through a narrative multi-step problem solving feature. The three cornerstones highlighted in the section above are evident within the lesson: goal challenge motivation {decided by pre-test assessment}, content-rich material, and authenticity through real-life application.
LESSON
Pre-test Assessment:
Provide paper-pencil assessment including photos of Canadian coins asking students to identify individual coins.
Addition questions for pre-test assessment may include:
Include two ‘making change’ questions that require student to calculate amount of change from $1.
Content-rich Material:
Read and discuss Dave Ramsay’s book entitled, My Fantastic Fieldtrip on saving money.
Provide pairs of students with real sets of Canadian coins with accompanying anchored money solving problems. Problems may require students to interact with other students in the class or with the teacher. An example of an anchored money problem solving scenario follows:
Macey has been saving her allowance for seven weeks. She has a saving goal of $20.00. Each week she receives $1.50. Three weeks ago, Macey decided to buy her sister a rubber ball for her birthday which cost $1.00. She used a loony from her savings . After seven weeks, Macey wanted to exchange all of her quarters for loonies, but she also wanted to keep half a dozen quarters for when she visited the candy machine at the grocery store when she went shopping with her mom. She knew that several of her classmates had loonies that they could exchange for her quarters. (At this time, go around to your classmates and exchange your quarters for loonies just like Macey wanted to.) Once Macey exchanged her quarters for loonies with her classmates, how many loonies does Macey have? How much money does Macey have all together? How much more money will Macey need to save to reach her saving goal?
Simulation Activity:
Illuminations – Coin Box {elementary level}: Initially, direct instruction is required to demonstrate how by clicking on the cent icon in the bottom right corner, the student can see the amount of each coin as they are US coins and difficult to decipher visually. Direct instruction should also be provided to guide the student to the “Instructions” tab and show the subtitled areas “Modes”. Student can then have time exploring the “Activity” section using the dropdown menu in the top left corner. Student should have ample time to explore all five activities including: “Count”, “Collect”, “Exchange”, “Change from Coins”, and “Change from Value”.
Transfer to ‘Real-Life’ Context: Students should have opportunity to transfer the simulated learning to a real-life context. An example of a real-life context is provided below, however adapting this to uniqueness of the learning community is recommended:
Cookie Sale – Each student bakes one dozen cookies to sell to classmates and other students at the school. Pricing: 1 cookie = $0.40, 2 cookies = $0.75, 3 cookies = $1.00, 4 cookies = $1.25, 5 cookies = $1.45, 6 cookies = $1.70. This activity allows for assessment by the teacher through observation. Student’s accuracy and ease of providing change could be assessed using a simple checklist. Students should work in pairs or small groups to help ensure that change to buyer is accurate.
Self Assessment/Reflection: A reflection activity is to be completed by each student. This activity requires the student to reflect on and share about growth and relevancy of learning. A self assessment printable is here:
Finkelstein, N.D., Perkins, K.K., Adams, W., Kohl, P., Podolefsky, N., & Reid, S. (2005). When learning about the real world is better done virtually: A study of substituting computer simulations for laboratory equipment. Physics Education Research,1(1), 1-8.
Srinivasan, S., Pérez, L.C., Palmer, R.D., Brooks, D.W., Wilson, K., & Fowler, D. (2006). Reality versus simulation. Journal of Science Education and Technology, 15(2), 137-141. doi: 10.1007/sl0956-006-9007-5
Through the readings from this past week, I have explored a seemingly disjointed array of ideas. Following is a brief overview:
Carraher, Carraher and Schliemann (1985) present the effect of contextualized learning on mental math computation processes with street vendor children in Brazil; Falk and Storksdieck (2010) share results from their study on adult leisure science learning at the California Science Center in Los Angeles; Butler and MacGregor (2003) provide an in-depth explanatory overview of the GLOBE program designed to enable “authentic science learning, student-scientist partnership, and inquiry-based pedagogy into practice on an unprecedented scale” (p.17)! Although these three readings are diverse in study and purpose, one significant theme pronounced itself throughout: the theme of contextualized learning. Regardless of the age of learner, socio-economical position, or location on this great planet, contextualized learning offers authenticity of learning and effective growth in both content areas and competencies.
When considering authentic learning, I like to refer to Herrington and Kervin’s (2007) definition:
The nine characteristics of authentic learning include:
(Herrington & Kervin, 2007)
Although all of these characteristics of learning are not prominently practiced in the networked communities explored during this past week, many, if not all, can be emphasized through teacher design by incorporating a combination of non-technology based and network community activities.
The follow learning outline is designed using the network community called Journey North along with other on-going non-technology nature study activities. As an individual and an educator who advocates for regular nature study as a part of one’s life, the Journey North community peeked my interest as a very viable resource to integrate with already implemented nature study practices with students from grades K-4. I have chosen two projects at Journey North that could be easily implemented with my younger distance learning students. Following is a chart with resources and activities aligned with the authentic characteristics of learning as described by Herrington et al. (2007).
While exploring the networked communities of GLOBE and Journey North, I was reminded of Tomatosphere which is supported through the Canadian based organization Let’s Talk Science.
Tomatosphere supports inquiry learning for K-12 students, inviting students to act like scientists. The premise of this networked community is to provide students the opportunity to investigate the growing of food for space-like conditions. Students receive two packets of seeds, one space-simulated packet and one regular. Students are provided with a story narrative to help them contextualize the purpose of their proceeding investigations. Students then plant the seeds and observe and record germination data. Data is then submitted and collaborated with results from other Canadian classrooms. The site states that this data is used by Canadian scientists to further understand long-term space exploration issues.
The Tomatosphere site includes a fairly extensive online Resource Library, as well as printable resources for student planning, investigation processes and data keeping.
Enjoy exploring!
I have been the facilitator of gathering students together to represent the unseen phenomena of molecular movement in states of matter. Students who are “solid” stand very close together and jiggle on the spot, while the “liquid” students stand further apart and move a bit more freely. The students who represent gas find their own space and move around in comparable bliss. I have had students dramatize the story of Archimedes and the king’s golden crown, and have seen a line of students model each part of the ear as sound moves through it. These students are taking on the roles of scientific phenomena, but their role play, as Resnick and Wilensky (1998) would suggest, is merely representing the results rather than “the processes and interactions that give rise to the results” (p.168).
Traditionally role play has found itself in the arts and humanities, helping students view themselves and society through varied lenses, making connections and altering perspectives. Winn (2003) quotes Reyes and Zarama suggesting that in the sciences, too, perspectives of self can be changed. The learned distinctions can often “tell us more about ourselves than about the world we are describing” (Winn, 2003, p.19). As well, Resnick and Wilensky (1998) have found that “role-playing activities provide a framework in which learners can start to make … distinctions – learning to project only the specific parts of their own experiences that are useful for understanding other creatures and objects” (pp.168-9). Can role play in the sciences and mathematics classroom aid in growing these distinctions? In subject areas where traditionally there is one correct answer, can seemingly random and indeterminate role play help bring order and understanding to complex ideas?
Resnick and Wilensky (1998) would affirmatively attest that role play is not intended for simply representing a result, but for “developing new relations with the knowledge underlying the phenomena” (p.167). In fact, they assert that for complex and system sciences, role play is ideal for providing “a natural path for helping learners develop an understanding of the causal mechanisms at work in complex systems. By acting out the role of an individual within a system…, participants can gain an appreciation for the perspective of the individual while also gaining insights into how interactions among individuals give rise to larger patterns of behavior” (p.167). Gaining insights into how localized patterns influence larger-scale, or globalized activity, is essential in understanding the intricacies of a complex system.
The enactivism theory of cognition supports Resnick and Wilensky’s affinity for role play within the sciences and mathematics. As described by Proulx (2013): “[e]nactivism is an encompassing term given to a theory of cognition that views human knowledge and meaning-making as processes understood and theorized from a biological and evolutionary standpoint. By adopting a biological point of view on knowing, enactivism considers the organism as interacting with/in an environment” (p.313). As the organism and environment interact, both change and adapt in response to the interaction, making them even more compatible. This evolution of structure is referred to as coupling (Proulx, 2013). Learning through enactivism is neither simple nor linear, but rather complex and undetermined. Using role play to understand mathematics and complex and science systems takes the student through an evolutionary process of change. The student takes on a role, interacting with the problems (environment) presented, and through this interaction poses new problems and pathways of solution. Along the way, the student finds their initial role is changing too, in order to adapt to the changing environment.
Interestingly, the chaos theory of instructional design also recognizes the value of instruction and learning that is evolutionary in nature (You, 1993). Similarly to Resnick and Wilensky, the chaos theory allows for patterns and order to emerge from seemingly randomness and chaos. You (1993) states that central to the chaos theory is “[t]he discovery that hidden within the unpredictability of disorderly phenomena are deep structures of order” (p.18). Quoting from Hayles (1990, 1991), the characteristics of the chaos theory are described with such phrases as a pattern of order within disorder; chaos is the precursor and partner to order rather than the opposite; and chaos is paradoxically locally random, but stable within a global pattern (You, 1993).
To bring this back to role play in mathematics and sciences, there is a need to recognize that complex ideas can be defined and understood through role play scenarios and interactions whether technology-based or non-technology-based. Through role play, localized complexities can be more clearly defined through continual problem solving and problem posing that allow the learner to begin to see and interpret patterns that emerge. As Proulx (2013) states, “The problems that we encounter and the questions that we undertake are thus as much a part of us as they are part of the environment; they emerge from our interaction with it” (p.315). Perhaps by opening the world of role play to mathematics and science students, we will see more students acting like Barbara McClintock, a Nobel-winning biologist who attributes “her greatest discoveries to the fact that she had a “feeling for the organism” and was able to imagine herself as one of the genes within the corn (Keller, 1983)” (Resnick & Wilensky, 1998, p.168). Perhaps McClintock’s experience is a call for educators to consider further the possibilities for when students are handed permission to relate and interact through imagination, and hence are given opportunity to experience phenomena.
The possible’s slow fuse is lit by the imagination. ~ Emily Dickinson
Resnick, M. & Wilensky, U. (1998) Diving into complexity: Developing probabilistic decentralized thinking through role-playing activities, Journal of the Learning Sciences, 7(2), 153-172. DOI: 10.1207/s15327809jls0702_1
Proulx, J. (2013). Mental mathematics, emergence of strategies, and the enactivist theory of cognition. Educational Studies in Mathematics, 84, 309-328.
Winn, W. (2003). Learning in artificial environments: Embodiment, embeddedness, and dynamic adaptation. Technology, Instruction, Cognition and Learning, 1(1), 87-114.
You, Y. (1993). What can we learn from the chaos theory: An alternative approach to instructional system design. Educational Technology Research and Development 41(3), 17-32. Retrieved from http://www.jstor.org/stable/30218385
Embodied learning acknowledges the individualism of the learner. The individual’s cognitive behaviour connects to past cognitive experiences and present interpretations in ways that are unpredictable and dependent on the the learner’s Umwelt. Umwelt is described as “the environment as the student sees and knows it – a limited view of the real world, ever changing as the student explores it and comes to understand it” (Winn, 2003, p.12). The learner’s interaction with the surrounding environment can be viewed as a biological interaction and a way of knowing. Metaphorically, the learner is an organism interacting with and within its environment. In effect, both the organism (the learner) and the environment evolve and are changed through the interaction (Proulx, 2013). Proulx refers to this interaction as enactivism and suggests that enactivism is the necessary cognitive theory behind problem solving, or more succinctly “problem posing”, in mental mathematics. Through problem posing, “the solver does not choose from a group of predetermined strategies to solve the task, but engages with the problem in a certain way and develops a strategy tailored to the task (both of which also evolve and are co-defined in the posing). Strategies are thus not predetermined, but continually generated for solving tasks” (Proulx, 2013, p.316).
In the brief article by Barab and Dede (2007), there is evidence of the cognitive theory of enactivism as the science learner is immersed in “narratively driven, experientially immersive, and multi-rich media” (p.1). The learner, as the organism, interacts with the immersive game-based simulated environment, bringing individualized input and then coupling {embedded interaction} with the environment. Problem posing exists as the learner poses solutions and generates strategies as interaction occurs with/in the simulated environment. In contrast to Proulx’s (2013) writing on enactivism and mental math problem posing through which students interact with an unprogrammed environment, Barab and Dede (2007) share studies of learners interacting with a programmed simulated environment. Can learner interaction with a programmed environment, even when programmed to be an adaptable environment, allow for enactivism to truly emerge? Or in other words, is the environment truly evolved by the learner, or is it an illusion? Also, what would be the best practices for teacher assessment and feedback when learners and environments are continually evolving and adapting?
In my own practice, I appreciate Proulx’s view on the individual learner and how this individualism aids the approach and walk through learning. I particularly appreciate that his focus is on mental mathematics, an area that seems to be neglected as students interact largely with workbook based curriculum and predetermined strategies. Continuing to engage students in number talks, breaks the misconception that there is one right way to find a solution, and opens the mindset to evolving possibilities. Immersive simulations that allow students to problem pose and structure solutions through interaction with the environment, and then use the adaptations to further generate strategies for solutions is ideal. I look forward to discovering simulations that encompass enactivism through the remainder of this module.
Following is a collection of visual syntheses comparing and contrasting T-GEM/Chemland with the following technology-enhanced learning environments: Learning for Use (LfU)/My World, Scaffolded Knowledge Integration (SKI)/WISE, and Anchored Instruction/Jasper. The visual syntheses contain a focus on TPCK and learner activity with the guiding TELE being T-GEM/Chemland, and all other TELEs being compared and contrasted through alignment with the T-GEM/Chemland framework.
Each one of these TELEs is developed on inquiry instruction and learning, with T-GEM/Chemland consisting of specifically model-based inquiry. Each one of these TELEs promotes a community of inquiry with purposeful teacher-student and student-student interactions. To emphasize the non-linear processes of inquiry, each visual synthesis is designed in a circular format.
Unique to T-GEM is the cyclical progress that the learner takes moving through the steps of the learning theory. Arrows are placed in each TELE’s visual representation to elicit the learner’s movement in comparison to the T-GEM’s model.
As a general mathematics and science teacher for elementary grade levels, the process of exploring, analyzing and synthesizing the four foundational TELEs presented in this course has been transformational in my development of TPCK. Initially, the importance of CK (Schulman, 1986), and my self-diagnosed lack of CK, was convicting as I tend towards growing in pedagogical ideas and creative ways of implementing them. To further this conviction, my understanding of inquiry processes and the intricate role that the teacher facilitates in conducting a community of inquiry began to become clearer throughout the readings and discussions of Module B. Skillful inquiry instruction requires a facilitator who is saturated in CK, being equipped to prepare, respond, question, prompt, and guide with carefully considered PK. At this time, I am challenged as an educator to begin with one brave adventure in mathematics using an anchored instructional approach, and another brave lesson in physical science using a T-GEM approach. I am certain that I will be generating, evaluating and modifying all along the way.
Cognition and Technology Group at Vanderbilt (1992). The jasper experiment: An exploration of issues in learning and instructional design. Educational Technology Research and Development, (40), 1, pp.65-80
Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching,38(3), 355-385.
Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905.
Linn, M. C., Clark, D. and Slotta, J. D. (2003), WISE design for knowledge integration . Sci. Ed., 87: 517–538. doi:10.1002/sce.10086
Shulman, L.S. (1986). Those who understand: Knowledge growth in teaching. Educational Researcher, 15(2), 4 -14.
When considering a challenging science concept, I recalled struggling with explaining the concept of floatation, or “sink or float”, when teaching kindergarten. Although exploring objects that sink and float in water is highly intriguing for young students, the reasoning behind which objects sink and float can get complicated and too abstract for a student at that age to fully understand. Why does a tiny popcorn kernel sink and a large watermelon float?
In the BC’s New Science Curriculum, density is not specifically addressed until grade six when students investigate heterogenous mixtures. In Suat Unal’s (2008) research, he recognizes that elementary students possess significant misconceptions relating to floatation as evidenced through other research by Biddulph and Osborne (1984) and Gürdal and Macaroglu (1997). This other research finds that “students offered many unrelated factors such as mass and weight” to explain floatation activity, and that even after sink and float investigations and learning of Archimedes had been completed, students “were unable to construct scientific understanding” about sink and float relations (p.135).
In preparing a T-GEM lesson, I wanted to include student investigation of objects that sink and float in water, as well as in other liquids, to help student understanding of the concept of density. Because of this specification, the Gizmos simulation that is included in the following lesson is ideal, whereas other simulations that I found online provide investigation solely with water. An image of the simulation follows:
T-GEM Lesson – Density – Grade 6 (BC Curriculum)
| Teaching Strategies | Student Activity | |
| Read Aloud – Chapter 5, “Archimedes and King Hiero’s Crown” from Archimedes and the Door of Science by Jeanne Bendick. | Class discussion narrating ideas presented through reading; teacher comments neutrally | |
|
GEM – Cycle 1 |
||
| G – Generate | Instruct students to investigate selected object in a sink and float investigation using water in a container. Students are to record observations on a T-chart as well as represent observations on a paper chart template using cut and paste images of the selected objects. Ask students to make a prediction about the types of objects that sink vs. float. | With a partner, students test sink and float tendencies of selected objects in water and record on a T-chart; Students place cut out pictures of objects onto chart template; After recording data, student journal predictions about the types of objects that sink vs. float. |
| E – Evaluate | Ask students to record anything that does not make sense about their observations and prediction – questions they may have or confusing patterns; Ask students to think of a way to conduct a sink and float investigation that could help clarify some of the observations and predictions that do not make sense. Prompt students with a change in variable – either the solid objects or the liquid. Teacher guides student through extension investigations using an alternate liquid. | Students discuss with partner and record observations and predictions that don’t makes sense; Share questions and confusing patterns with class and plan a new investigation with changing one variable. Watch teacher directed demonstration and participate in class discussion. |
| M- Modify | Ask students to determine what changes they need to add to their T-chart and paper pictorial chart to accommodate the new information accessed from the teacher-led investigation | Student makes adjustments to representations of t-chart and paper pictorial chart by including results with variable change. |
|
T-GEM – Cycle 2 |
||
| G- Generate | Direct students to Gizmos online simulations: https://www.explorelearning.com {Teacher needs to previously set up an account and select simulation to add to their “class”.}
Lead students to the the elementary level lesson under Physics called “Density” Provide a short explanation of the activity, sharing that instructions are provided in text within the simulation. Remind students to record on a new chart the weight (g) of the object when measured on the scale, the volume displacement (mL)of the object within the graduated cylinder, and the the ability of the object to sink and float in each of the available liquids (water, oil, gasoline, sea water and corn syrup). After all objects have been tested, journal a relation statement based on the acquired data. |
In partners, students use the Density simulation measuring weight and volume displacement of the following objects: ping pong ball, golf ball, toy soldier, apple, chess piece, penny, egg, rock, gold nugget, crown 1 and crown 2. Students will test the floatation of each object in five different liquids and record their observations. Students will analyze their data and make a relation statement in their journal. |
| E-Evaluate | Teacher provides students with the equation for density:
Density = Mass/Volume Water = 1.00 g/mL Oil = 0.92 g/mL Gasoline = 0.70 g/mL Sea Water = 1.03 g/mL Syrup = 1.33 g/mL |
Students compare the density of the measured objects using the density equation and with the density of the liquids and evaluate their relation statement making changes as necessary. |
| M-Modify | Ask students to design a pictorial representation (model) of the data. Students can choose to represent objects that sink, or float, or both. The model should include density measurements of both the liquids and objects. The model should include a comparison of two or more liquids. Recommend using a chart or graph format with pictorial representations of objects. | Students choose data to include in their model representation following criteria provided by teacher. |
Edelson’s (2001) writing on the framework of Learning for Use (LfU) model requires the teacher and learner to situate inquiry-based learning within a context of technology use and relevant future use. LfU is designed with three processes of learning, each incorporating the use of technology and causing the student to recognize the “usefulness of the content beyond the learning environment” (p.373). These three processes are defined as motivation, construction and refinement.
Edelson goes into significant depth about the LfU design strategies and elements contained within the Create-a-World Project, as well as a reasoning description of the purpose for including technology into the LfU model. For each strategy supporting a learning process, Edelson states the purpose behind the technology. These purposes include: a way of affording constructive learning , “improv[ing] upon the real world for discrepant events [i.e.] phenomena that are too small or too large, too fast or too slow, too hot or too cold for direct observation can all be reproduced using recording or simulation technologies” (p.376), offering students participation in “guided discovery by allowing them to conduct investigations with data … [and] by providing simulations of physical phenomena that students can directly interact with” (p.377). Furthermore, technology provides “[t]he ability to present information in a wide variety of formats … [i.e.] text, graphics, audio, and interactive computational objects” (p.378) as well as support the act of record keeping during inquiries for student reflection. Edelson’s intentional use of technology within the LfU framework, offers a standard for designers when considering the inclusion of technology within a learning framework. Does the technology enhance knowledge construction by affording practical tools for inquiry? Edelson’s inclusion of technology is extended in necessitating use and application: “Because knowledge application requires meaningful, goal-directed tasks, the technologies that can support knowledge application are the technologies that will allow learners to conduct meaningful tasks” (p.380).
Within both Edelson’s example of students using Create-a-World Project and Perkins, Hazelton, Erickson and Allen’s (2010) study on students using a GIS (Geographic Information Systems), there is a connection to what David Sobel (2004) refers to as place-based learning. Sobel describes place-based education as “the process of using the local community and environment as a starting point to teach concepts … emphasizing hands-on, real-world learning, enhanc[ing] students’ appreciation for the natural world, and creat[ing] a heightened commitment to serving as active, contributing citizens” (Sobel, 2004).
The connection between LfU and place-based learning is worth consideration as GIS tools afford the opportunity for students to interact initially within their community and then beyond. Interestingly, the practice of place-based learning is promoted within the BC Ministry’s curriculum in relation to indigenous learning. Combining place-based learning with GIS tools offers opportunity for indigenous and western learners to gain a deeper understanding of their local world, and intuitively of the world beyond them. Inquiries related to physical environmental changes, population increase or decline of species, migration patterns and weather patterns are all relevant areas of situated learning for both indigenous and western learners.
In Perkins’ et al (2010) study, there is support for the inclusion of place-based learning with GIS tools as middle school students participate in mapping their school yard using My World GIS curriculum. Perkins et al (2010) find a significant increase in students’ spatial skills after only three days of working with the GIS and GPS tools. They partially attribute this increase in skills to the inclusion of place-based learning: “Introducing GIS and GPS in the students’ familiar and immediate surroundings more easily bridges the gap between the real and digital worlds. Each student has tangible experience with their schoolyard and, therefore, some sense of that space that will allow them to construct new knowledge in the context of a place that they know”(p.217).
In closing, the LfU model requires highly structured inquiry-based processes such as “hypothesizing, collecting and evaluating evidence, and defending conclusions based on evidence” (Edelson, 2001, p. 362). Furtak (2006) describes guided scientific inquiry as inquiry when the teacher knows the answer, but is cautious with the power of suggestion. In Linn, Clarke and Slotta’s (2003) article on WISE, a more structured approach to inquiry is also suggested: “If inquiry steps are too precise, resembling a recipe, then students will fail to engage in inquiry. If steps are too broad, then students will flounder and become distracted. Finding the right level of detail requires trial and refinement and, in some cases, customization to local conditions and knowledge” (p.522). Through the explorations of various technology-based inquiry environments, it is evident that the teacher and/or designer is an expert in processes and in content, allowing for processes of inquiry to be experienced and developed, while supporting inquiry problem-solving and refinements through in-depth knowledge of content.
