Monthly Archives: March 2017

Summary Synthesis

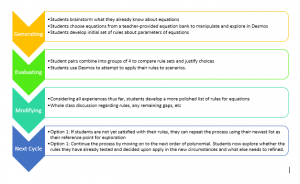
The following is an analysis of the four learning environments that aid in using technology to teach math and science. The analysis consists of similarities and differences between Anchored Instruction, WISE, LfU, and T-GEM.

	Anchored Instruction and Jasper	SKI and WISE	LfU and MyWorld	T-GEM and Chemland
Similarities	1. All models enforced the scientific process that began with creating hypotheses. Once hypotheses are created, data is analyzed, problems are solved and the hypotheses are reworked, adjusted, and modified. 2. All models also favor cooperation among students in groups so to teach students the vital skill of working with others in any field of math or science. 3. All models require balance of direct instruction and inquiry led discussions to help students both learn content, and then use that content in the right contexts.
	Differences
1. Mode of Operation	Jasper used videos of complex problems that have inviting and engaging themes to help students learn content by problem solving.	The WISE environment was an immersive experience where students were exposed to content, problems, and methods of recording and analyzing thoughts and data.	The LfU model emphasized the importance of teaching with the right context and involved three phases: motivation, knowledge construction, and knowledge retrieval.	T-GEM via Chemland was an enlightening model that provided students with the three phases of learning: generating hypotheses, evaluating these hypotheses and then modifying the hypotheses based on new data.
2. Type of technology involved	Jasper focused primarily on creating videos with engaging dialogue.	The WISE environment was a completely immersive and interactive computer environment with a large database of projects.	The LfU model gave the teacher freedom to use technologies that would best fit the three phase model.	Chemland was a collection where students could observe different ways of analyzing data simultaneously like videos, graphs, simulations etc.

As a direct result of analysis of these four learning models, important lessons have emerged. The presence of math and science inquiry is vital in the classroom for students to truly understand and appreciate the ways experts and professionals essentially do math or do science. These learning models are exemplary in guiding teachers to conduct projects in their classrooms that follow the scientific process and at the same time teach content as well. I have learned through this analysis that content and the scientific process can be taught simultaneously instead of compartmentalizing them as instruction days versus lab days.

T-Gem summary chart

	Key Words	Guiding philosophies	Key design points	Participant role	Teacher role	Benefits	Drawbacks	Associated technology
Anchored Instruction	Authentic contexts	Constructivism, Cognitive apprenticeship, Social Cognitive Theory, inquiry	Knowledge is constructed through engagement with richly details and complex problems. Real life situations	Problem solver, Researcher, Data gatherer	Facilitator, Error correction, occasional hints, scaffolding as needed	Highly engaging. Transferable problem solving skills,. Authentic contexts	Very time consuming, requires at least 1 device per small group, may lead to high student frustration, limited supply of availabel materials	Jasper Lessons
SKI	Evidence based revision of conceptions	Constructivism, Social Cognitive Theory, inquiry	1) making thinking visible, (2) making science accessible, (3) helping students learn from each other, and (4) promoting lifelong learning.	Revisor of personal knowledge, Connector of concepts,	Activity designer, provider of pivotal cases, directing integration of conceptions with new knowledge and evidence	Flexible related authoring environment (WISE), promotes flexible thinking	Must monitor carefully for alternative conceptions, limited resources available as yet for lower grades. Smaller groups may require more technology resources	WISE
LfU	Making learning useful	Constructivism, Cognitive apprenticeship, Situated cognition, inquiry	Learning is most effective when it is directed at a goal. How knowledge is constructed determines how it will be used in the future. To be useful, knowledge must be converted from declarative to procedural knowledge	Problem solver, concept revisor, data gatherer	Poser of problems, Just in time knowledge provider	High relevance to students’ home context	Complex tools for younger students. Activities may need revision to reflect home context. Data may be hard for younger students to digest	ArcGis
T-Gem	Iterative thinking	Constructivism, inquiry	Using technology, generate hypotheses, evaluate them, and modify the hypotheses according to the results	Theorist, Tester/experimenter, Research evaluator	Provide tools/simulations and background knowledge. Confirm accuracy of final conceptions/models	Models the real work of scientists. Builds pattern recognition	Give a false sense of the ease of generating data. Requires significant lateral thinking	ChemLand, PHET simulations

Synthesizing the 4 Learning Environments-My Thoughts

Synthesis of the Four Learning Environments Explored- table

The link provided (table) is the synthesis that I’ve created to compare and contrast the four learning environments. I look forward to any discussions that arise from this table, as I was feeling a bit unsure about a few of my presumptions after having explored each one. I did find MANY overlapping ideas/tenets and I also feel that as these learning environments change based on upgrades, new understandings and student/educator needs that more overlap is inevitable. I do think that each technology supported environment provides its own “positives” depending on the style of the educator, the needs of the students, the age of the students and access to technology. In addition, timelines must be considered and I believe each of these requires more time to allow students to find relationships, deepen their understandings, communicate with each other and reflect on their learnings, and even more time if they are to apply these understandings in real-world contexts. That being said it cannot be understated that these environments provide deep, rich understandings. In addition, I would like to add that supporting and educating teachers to use these valuable resources should be a goal so that science/math education can continue to support deep, engaging and meaningful learning for students.

Since I am an elementary educator I would also liike to put forth that these should be used in the early grades so that students can begin to consolodate their scientific understandings before “the damage is done”, so to speak. What I mean is that it seems that many misconceptions re: science concepts are formed in early learning and providing for engaging science problem solving and investigations that address these misconceptions would go a long way in hopefully curbing this trend. That being said, just using “technology” to teach scienc e is not a panacea, as there is much misinformation represented in a variety of science vidoes, interactive games, etc. online that is purposely “dumbed down” to be accessible to younger students. In addition, the ideas about technology integration held by the educator cannot be overlooked, as these understandings can colour how the technology is implemented. We need to be cognizant of this as educators and work towards adapting sound technologically enhanced learning environments into our early elementary classrooms.

Refraction of Light and T-Gem Principles

One challenge for students is to understand the refraction of light. For example, when a student observes a straw in a glass of water, the straw looks like it is bending. This is due to the properties of light, but this understanding can be fraught with misconceptions regarding how light behaves. Some interesting misconceptions about light may be that water does not reflect or absorb light but light can go through it, light always passes straight through transparent objects (without changing direction) or that light needs air to travel (Sampson & Schleigh, 2016).

Research notes that although light is an everyday phenomenon that we constantly observe, students often display learning difficulties and hold unscientific understanding on physics concepts of light wave (Srisawadi & Kroothkeaw, 2014). In addition, concepts of light such as its speed and wave length are removed from the range of perceptions of the human senses, and so optics instruction can be subject to interpretation, so there is a need for careful consideration in physics teaching process (Srisawadi & Kroothkeaw, 2014). Computer simulations can broach this divide. As noted, computer simulations can enhance generating relationships and allow students and teachers the opportunity to view trends, variables and visual representation in more concrete ways which may lead to more accurate conceptual understandings (Khan, 2011).

In order to generate information about this phenomenon the educator can begin an open-ended discussion to find out current concepts about light. Questions such as:

What is light?

Where do you think light comes from?

How does light travel?

This will allow the educator to begin to understand what conceptions and misconceptions the students may hold about light and will also allow the students to begin thinking about the concept. As this discussion is occurring the educator can note responses on chart paper or interactive whiteboard so that ideas can be reviewed as the process of understanding continues. As an educator I would incorporate “accountable talk” which will allow students to defend their ideas and question others about their understandings. Examples of accountable talk would be statements like;

“I wonder why….?

“I see what you are saying (rephrase)”

“What you said made me think….”

Then as an educator I would facilitate a review of the ideas generated in the group discussion through referring and restating the list created by students. I would break this down further into “Our First Ideas about Light” and then create another section for questions we now have about light. This would be labelled “Our Questions about Light”. We would brainstorm some questions that we have. Then I would provide students with appropriate books and internet resources about light. I would also show them a model or a picture of a straw in a glass of water. The straw appears to bend and so I would ask them how they would explain the phenomenon. After they have a chance to read/view this information, I would ask them to work with a partner, independently or in a small group (provide choice) and to draw or create a clay model of their understanding of light.

We would then reconvene and compare our models. I would give students time to explain their models to their peers so that I could continue to assess possible misconceptions. At this point the students may begin to reformulate their understandings based on new learning from their peers. Then we would watch several simulations about light refraction. I would ask the students to consider their previous understandings by asking “Do you need to change your original drawing/model? Or “Do you think you need to modify your original drawing/model?” Our new understanding would be discussed and a new category would be added to our discussion titled “New Understandings”.

Bending Light Simulations

Refraction in Water Simulation

Bending Light Simulation

References

Bending Light. (n.d) Retrieved March 1, 2017, from https://phet.colorado.edu/sims/html/bending-light/latest/bending-light_en.html

Khan, Samia (2011). New pedagogies on teaching science with computer simulations. Journal of Science Education and Technology 20, 3 pp. 215-232.

Refraction in water. (n.d.) Retrieved February 29, 2017, from https://www.khanacademy.org/science/physics/geometric-optics/reflection-refraction/v/refraction-in-water

Sampson, V., & Schleigh, S. (2016). Scientific Argumentation in Biology [PDF file]. Arlington,Virginia. NSTA Press Book. Retrieved from http://static.nsta.org/files/PB304Xweb.pdf

Srisawasdi, N. & Kroothkeaw, Supporting students’ conceptual development of light refraction by simulation-based open inquiry with dual-situated learning model. S. J. Comput. Educ. (2014) 1: 49. doi:10.1007/s40692-014-0005-y

Synthesis

Visual Compare and Contrast of four environments on learning goals and theory: https://www.mindmeister.com/851561193/foundational-technology-enhanced-learning-environments

My concept map of the four technology-enhanced learning environments, I have noticed that many ideas of the different environments overlapped. As can be seen, connections are made between some related concepts and ideas. I realize that there are more connections to be made, which is why I chose a concept map so that I can continuously add more connections in the future. After exploring these four design of technology-enhanced learning environments, it has inspired me with a diversity of ways of integrating learning in the math and sciences for my students. In particular, I have learned that the skills students acquire in the learning process are more important than the content. Some of these skills include inquiring, reflecting, creating, critiquing, problem solving, collaborating, evaluating, generating, among others. For instance, teaching math has always followed a strict curriculum of different separate units but is not taught in ways that are applicable to students. However, Anchored Instruction integrates math into meaningful case studies that allow students to make connections between the math concepts with real life applications. As well, the Scaffolded Knowledge Integration emphasizes the importance of peer-to-peer learning experiences in learning. Another example is Learning-for-Use, which introduces the critical idea of motivation in learning that leads to knowledge construction. Finally, T-GEM is the use of technology-enhanced methods to engage in the process of generating, evaluating and modifying relationships in knowledge. It has impacted how I will teach in my own teaching context (i.e. Grade 6s and 7s) because it seems that the teacher’s role is to facilitate and guide students’ learning rather than directly teach content and skills to them. Furthermore, teachers are encouraged to be participate as a shared learner in the process. This frees the teacher from being the sole source of knowledge and be more available to students observe students’ processes and performance of learning. Overall, learning about these different technology-enhanced learning environments has opened up a plethora of possibilities to teach all types of students in authentic, meaningful ways.

Cognition and Technology Group at Vanderbilt (1992a). The Jasper experiment: An exploration of issues in learning and instructional design. Educational Technology, Research and Development, 40(1), 65-80.

Edelson, D.C. (2001). Learning-for-use: A framework for the design of technology-supported inquiry activities. Journal of Research in Science Teaching,38(3), 355-385.

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905.

Linn, M., Clark, D., & Slotta, J. (2003). Wise design for knowledge integration. Science Education, 87(4), 517-538.

Lines, Curves, and Equations – Oh My! [Desmos and Equation Development Using T-GEM]

Initially, I found it challenging to apply T-GEM to mathematics as I found it hard to picture, but after completing this activity, it seems to be very similar to many of the activities I already strive to do with my students. A challenging concept for my students in secondary math is creating and understanding equations for lines and quadratic polynomials using graphs or scenario details. This challenge has been identified through concept pre-assessments, the nature of their questions throughout their work, and continued struggles on summative assessments. They struggle to make the connection between the information they are given and the algebraic, abstract representation.

Generating: For the generating phase, I would have students brainstorm what they already think they know about equations with regards to what they mean and how they can be used. Students will randomly select equations from a centralized equation bank to explore using Desmos (available either online or as an app). Working in pairs, students will develop a set of rules for creating equations based on their explorations of manipulating equations within Desmos.

Evaluating: Once students have developed their guidelines, pairs of pairs will be joined into groups of 4, in which students will compare their initial hypotheses and justify their perspectives. Ideally, reflection would occur as students need to explain how they arrived at particular conclusions. At this point, scenario-based problems will be introduced to the original pairs, expanding upon the initial work with base equations. Students now need to determine if the rules they established in their initial phases apply appropriately to their new scenarios. If they are not able to use their rules to accurately create an equation to represent the scenario and use it to solve problems, they will need to identify the gaps and determine what adjustments need to be made. Desmos will continue to be the technology tool at this level, as students are able to easily test, adjust, and visualize their inputs.

Modifying: In their pairs, students will reevaluate their list of rules for equations, taking into consideration their initial hypotheses, their discussions with peers, and their testing of their hypotheses. As a class, we would come back together for a large class discussion to compile their ideas into a community-based understanding.

This process could be further expanded to include different types of polynomials. For example, students initially working with equations of lines could then generate, evaluate, and modify new hypotheses regarding quadratics, based on their work with linear relationships. Subsequently, work with quadratics could be further cycled to work with cubics, then quartics and other higher order polynomials, as appropriate.

By having students use Desmos to work with the different parameters of the equations, they are able to actually experiment to see the effects of changes, rather than simply being told to memorize, for example, that the c value of a quadratic equation determines the vertical position of the graph but doesn’t directly affect its shape when a and b stay constant.

I believe that many mathematical principles and concepts can be approached using similar strategies to those used for scientific principles and concepts, and aim to include them when possible in my teaching. One challenge I have in senior math is the perception of teachers regarding the comparable value of experimentation and hands-on math in the university-bound courses as compared to the middle school or college/workplace-bound courses. I am often met with resistance from colleagues who don’t believe there is a place for experimental or hands-on learning in the higher-level university-bound courses, and that such activities are frivolous at that level. Do you feel that there is value in hands-on math learning for the senior level university-bound math students?

Sources Consulted:

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905

Khan, S. (2010). New pedagogies for teaching with computer simulations. Journal of Science Education and Technology, 20(3), 215-232.

Diffusion

One of the areas that I noticed my students struggling with when I taught science in the past was diffusion and osmosis. There were a few ways I could tell this was a topic rife with misconceptions:

They were concepts that most students struggled to define/explain adequately on tests.
Even high performing students had trouble differentiating the two.
Their hypotheses and reflections on labs showed a lack of understanding

I think one of the reasons is that they have difficulty with the scale that is involved and the concept of concentration gradients. From a scale point of view, I think students have misconceptions about liquids because of their sensory observations. They see liquids as homogenous substances, and struggle to understand that there are tiny atoms/molecules moving around and colliding. With concentration gradients, I think they have a difficult time understanding why particles move from areas of high concentration to areas of low concentration. Two prominent misconceptions I have noticed arise from some of the most popular ways of describing diffusion. The first is the personification of particles – teachers often imbue consciousness on particles by describing diffusion in terms of particles ‘seeing’ the high concentration and ‘knowing’ that they must move to another place. Another way of describing concentration gradients is the idea of a ‘downhill’ force that takes particles from high concentration to low concentration. My students would often take this explanation and turn it into a misconception that diffusion was driven by gravity.

For the purposes of my T-GEM, I have ‘created’ a new tech tool – an interactive demo/game in which particles move around the screen in a way consistent with kinetic molecular theory (please forgive my improvised attempt at showing this visually in my video!), and students can control the variables. I think interacting with a demo/game like this would help dispel misconceptions and help students make meaning of the process.

T-GEM: Building Circuits

Last year I taught Grade 7 Science and one of the more difficult outcomes to teach was Electricity. Many of the students know that electricity is there but since they cannot “see” it the struggled with the concepts.

These were the outcomes for the electricity unit:

Construct and draw a simple series circuit and a simple parallel circuit (P1)
Compare the characteristics of series and parallel circuits (P2)
Describe simple applications for series and parallel circuits (P3)

The following is a 3-step T-GEM cycle for Electricity :

Teacher

Students

Generate:

Teacher asks students to create a mind map around the word electricity.

Teacher listens while taking note of the misconceptions that they may possess.

In small groups students will generate a mind map, letting their ideas and words flow.

Students present their mind maps and hang them on the wall.

Evaluate:

Teacher gives students a battery, wires, switch, and a light bulb. Ask students to form a complete circuit and try to light the bulb.

Teacher walks around observing how students are constructing the circuits

Students working together they try and conduct a circuit.

Modify:

Teachers helps any groups that needs it and explains the difference between a series and a parallel circuit.

Students look at where they went wrong and modify accordingly.

I used the phet website (https://phet.colorado.edu/en/simulation/legacy/circuit-construction-kit-dc)

This was an excellent resource to use during this unit because the resources at our school are few and far between and this really helped the students understand the concept of building circuits.

Staying Afloat – Sink and Float Density T-GEM

When considering a challenging science concept, I recalled struggling with explaining the concept of floatation, or “sink or float”, when teaching kindergarten. Although exploring objects that sink and float in water is highly intriguing for young students, the reasoning behind which objects sink and float can get complicated and too abstract for a student at that age to fully understand. Why does a tiny popcorn kernel sink and a large watermelon float?

In the BC’s New Science Curriculum, density is not specifically addressed until grade six when students investigate heterogenous mixtures. In Suat Unal’s (2008) research, he recognizes that elementary students possess significant misconceptions relating to floatation as evidenced through other research by Biddulph and Osborne (1984) and Gürdal and Macaroglu (1997). This other research finds that “students offered many unrelated factors such as mass and weight” to explain floatation activity, and that even after sink and float investigations and learning of Archimedes had been completed, students “were unable to construct scientific understanding” about sink and float relations (p.135).

In preparing a T-GEM lesson, I wanted to include student investigation of objects that sink and float in water, as well as in other liquids, to help student understanding of the concept of density. Because of this specification, the Gizmos simulation that is included in the following lesson is ideal, whereas other simulations that I found online provide investigation solely with water. An image of the simulation follows:

T-GEM Lesson – Density – Grade 6 (BC Curriculum)

	Teaching Strategies	Student Activity
	Read Aloud – Chapter 5, “Archimedes and King Hiero’s Crown” from Archimedes and the Door of Science by Jeanne Bendick.	Class discussion narrating ideas presented through reading; teacher comments neutrally
GEM – Cycle 1
G – Generate	Instruct students to investigate selected object in a sink and float investigation using water in a container. Students are to record observations on a T-chart as well as represent observations on a paper chart template using cut and paste images of the selected objects. Ask students to make a prediction about the types of objects that sink vs. float.	With a partner, students test sink and float tendencies of selected objects in water and record on a T-chart; Students place cut out pictures of objects onto chart template; After recording data, student journal predictions about the types of objects that sink vs. float.
E – Evaluate	Ask students to record anything that does not make sense about their observations and prediction – questions they may have or confusing patterns; Ask students to think of a way to conduct a sink and float investigation that could help clarify some of the observations and predictions that do not make sense. Prompt students with a change in variable – either the solid objects or the liquid. Teacher guides student through extension investigations using an alternate liquid.	Students discuss with partner and record observations and predictions that don’t makes sense; Share questions and confusing patterns with class and plan a new investigation with changing one variable. Watch teacher directed demonstration and participate in class discussion.
M- Modify	Ask students to determine what changes they need to add to their T-chart and paper pictorial chart to accommodate the new information accessed from the teacher-led investigation	Student makes adjustments to representations of t-chart and paper pictorial chart by including results with variable change.
T-GEM – Cycle 2
G- Generate	Direct students to Gizmos online simulations: https://www.explorelearning.com {Teacher needs to previously set up an account and select simulation to add to their “class”.} Lead students to the the elementary level lesson under Physics called “Density” Provide a short explanation of the activity, sharing that instructions are provided in text within the simulation. Remind students to record on a new chart the weight (g) of the object when measured on the scale, the volume displacement (mL)of the object within the graduated cylinder, and the the ability of the object to sink and float in each of the available liquids (water, oil, gasoline, sea water and corn syrup). After all objects have been tested, journal a relation statement based on the acquired data.	In partners, students use the Density simulation measuring weight and volume displacement of the following objects: ping pong ball, golf ball, toy soldier, apple, chess piece, penny, egg, rock, gold nugget, crown 1 and crown 2. Students will test the floatation of each object in five different liquids and record their observations. Students will analyze their data and make a relation statement in their journal.
E-Evaluate	Teacher provides students with the equation for density: Density = Mass/Volume And the density measurements for the 5 liquids within the simulation: Water = 1.00 g/mL Oil = 0.92 g/mL Gasoline = 0.70 g/mL Sea Water = 1.03 g/mL Syrup = 1.33 g/mL Ask student to evaluate their relation statement using this new information	Students compare the density of the measured objects using the density equation and with the density of the liquids and evaluate their relation statement making changes as necessary.
M-Modify	Ask students to design a pictorial representation (model) of the data. Students can choose to represent objects that sink, or float, or both. The model should include density measurements of both the liquids and objects. The model should include a comparison of two or more liquids. Recommend using a chart or graph format with pictorial representations of objects.	Students choose data to include in their model representation following criteria provided by teacher.

Bendick, J., (1995). Archimedes and the door of science. Bathgate ND: Bethlehem Books.

BC’s New Curriculum, (n.d.). Science 6. Retrieved from https://curriculum.gov.bc.ca/curriculum/science/6

ExploreLearning, (2017). Gizmos. Retrieved from https://www.explorelearning.com

Khan, S. (2007). Model-based inquiries in chemistry. Science Education, 91(6), 877-905. Doi 10.1002/sce.2022

Unal, S.,(2008). Changing students misconceptions of floating and sinking using hands-on activities. Journal of Baltic Science Education, 7(3), 134-146. Retrieved from http://oaji.net/articles/2014/987-1404719938.pdf

T-GEM and Earth’s rotation

Challenging Concept:

The concept that I choose was one that my grade 4 class struggled with early on in the school year. Understanding the lunar cycle, phases of the moon, along with the tilt of the earth’s axis and its impact on the various seasons was a challenge for them. I used various demonstrations with a globe, flashlight and pictures on the projector. When they completed an extension activity afterwards, many of them still couldn’t explain how those things worked.

3 Step T-Gem cycle


	Discuss the moon and Earth’s gravity and rotation
Generate	Demonstrate the rotation of the Earth using a model around the Sun. Use a flashlight to shine on various parts of the and Earth to show where light would hit and various times of the year and day. Ask questions about where the students think it is cold/hot, daylight, nighttime
Evaluate	Allow students to create their own diagrams of the Earth’s rotation and demonstrate how that impacts the various seasons.
Modify	Ask students to consider their original ideas then consider how the various shapes of the moon are impacted by the rotation around the Earth

http://highered.mheducation.com/olcweb/cgi/pluginpop.cgi?it=swf::800::600::/sites/dl/free/0072482621/78778/Lunar_Nav.swf::Lunar%20Phases%20Interactive

I found a website the models lunar phases and provides students with a few different vantage points with regards to positing on the moon and what that would look like on earth based on the different times and days of the year. You can see the sun rise and fall as the clock moves throughout the day, the moon’s position around the earth change, as well as the calendar year moving through each day.